Quot scheme

In algebraic geometry, the Quot scheme is a scheme parametrizing locally free sheaves on a projective scheme. More specifically, if X is a projective scheme over a Noetherian scheme S and if F is a coherent sheaf on X, then there is a scheme 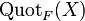$\operatorname{Quot}_F(X)$ whose set of T-points 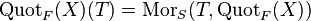$\operatorname{Quot}_F(X)(T) = \operatorname{Mor}_S(T, \operatorname{Quot}_F(X))$ is the set of isomorphism classes of the quotients of 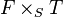$F \times_S T$ that are flat over T. The notion was introduced by Alexander Grothendieck.

It is typically used to construct another scheme parametrizing geometric objects that are of interest such as a Hilbert scheme. (In fact, taking F to be the structure sheaf 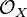$\mathcal{O}_X$ gives a Hilbert scheme.)

References

Nitsure, N. Construction of Hilbert and Quot schemes. Fundamental algebraic geometry: Grothendieck’s FGA explained, Mathematical Surveys and Monographs 123, American Mathematical Society 2005, 105–137.
https://amathew.wordpress.com/2012/06/02/the-stack-of-coherent-sheaves/