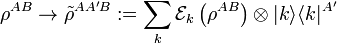Quantum instrument

In physics, a quantum instrument is a mathematical abstraction of a quantum measurement, capturing both the classical and quantum outputs. It combines the concepts of measurement and quantum operation.

Definition

Quantum instruments are usually defined as a weighted collection of completely positive maps, the sum of which is trace preserving. That is, it is a collection $\mathcal{E}_k$ of operators that act as

\rho^{AB} \rightarrow \tilde{\rho}^{AA'B} := \sum_k \mathcal{E}_k \left( \rho^{AB} \right)\otimes \vert k \rangle \langle k\vert^{A'}

A quantum instrument is more general than a quantum operation because it records the outcome k of which operator acted on the state. An expanded development of quantum instruments is given in quantum channel.

References

E. Davies, J. Lewis. An operational approach to quantum probability, Comm. Math. Phys., vol. 17, pp. 239-260, 1970.
Distillation of secret key paper
Another paper which uses the concept