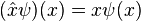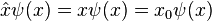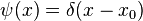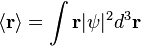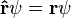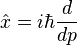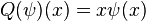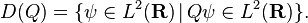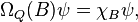Position operator

In quantum mechanics, the position operator is the operator that corresponds to the position observable of a particle. The eigenvalue of the operator is the position vector of the particle.[1]

Introduction

In one dimension, the wave function 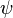\psi represents the probability density of finding the particle at position 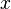x . Hence the expected value of a measurement of the position of the particle is

\langle x \rangle = \int_{-\infty}^{+\infty} x |\psi|^2 dx = \int_{-\infty}^{+\infty} \psi^* x \psi dx

Accordingly, the quantum mechanical operator corresponding to position is 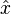\hat{x} , where

(\hat{x} \psi)(x) = x\psi(x)

Eigenstates

The eigenfunctions of the position operator, represented in position basis, are dirac delta functions. To show this, suppose 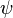\psi is an eigenstate of the position operator with eigenvalue 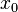x_0 . We write the eigenvalue equation in position coordinates,

\hat{x}\psi(x) = x \psi(x) = x_0 \psi(x)

recalling that 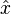\hat{x} simply multiplies the function by 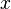x in position representation. Since 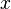x is a variable while 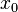x_0 is a constant, 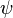\psi must be zero everywhere except at 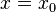x = x_0 . The normalized solution to this is

\psi(x) = \delta(x - x_0)

Although such a state is physically unrealizable and, strictly speaking, not a function, it can be thought of as an "ideal state" whose position is known exactly (any measurement of the position always returns the eigenvalue 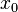x_0 ). Hence, by the uncertainty principle, nothing is known about the momentum of such a state.

Three dimensions

The generalisation to three dimensions is straightforward. The wavefunction is now 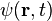\psi(\bold{r},t) and the expectation value of the position is

\langle \bold{r} \rangle = \int \bold{r} |\psi|^2 d^3 \bold{r}

where the integral is taken over all space. The position operator is

\bold{\hat{r}}\psi=\bold{r}\psi

Momentum space

In momentum space, the position operator in one dimension is

\hat{x} = i\hbar\frac{d}{dp}

Formalism

Consider, for example, the case of a spinless particle moving in one spatial dimension (i.e. in a line). The state space for such a particle is L2(R), the Hilbert space of complex-valued and square-integrable (with respect to the Lebesgue measure) functions on the real line. The position operator, Q, is then defined by:[2][3]

Q (\psi)(x) = x \psi (x)

with domain

D(Q) = \{ \psi \in L^2({\mathbf R}) \,|\, Q \psi \in L^2({\mathbf R}) \}.

Since all continuous functions with compact support lie in D(Q), Q is densely defined. Q, being simply multiplication by x, is a self adjoint operator, thus satisfying the requirement of a quantum mechanical observable. Immediately from the definition we can deduce that the spectrum consists of the entire real line and that Q has purely continuous spectrum, therefore no discrete eigenvalues. The three-dimensional case is defined analogously. We shall keep the one-dimensional assumption in the following discussion.

Measurement

As with any quantum mechanical observable, in order to discuss measurement, we need to calculate the spectral resolution of Q:

Q = \int \lambda d \Omega_Q(\lambda).

Since Q is just multiplication by x, its spectral resolution is simple. For a Borel subset B of the real line, let 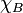\chi _B denote the indicator function of B. We see that the projection-valued measure ΩQ is given by

\Omega_Q(B) \psi = \chi _B \psi ,

i.e. ΩQ is multiplication by the indicator function of B. Therefore, if the system is prepared in state ψ, then the probability of the measured position of the particle being in a Borel set B is

|\Omega_Q(B) \psi |^2 = | \chi _B \psi |^2 = \int _B |\psi|^2 d \mu ,

where μ is the Lebesgue measure. After the measurement, the wave function collapses to either

\frac{\Omega_Q(B) \psi}{ \|\Omega_Q(B) \psi \|}

or

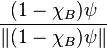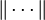\frac{(1-\chi _B) \psi}{ \|(1-\chi _B) \psi \|} , where \| \cdots \| is the Hilbert space norm on L2(R).

See also

References

  1. ↑ Atkins, P.W. (1974). Quanta: A handbook of concepts. Oxford University Press. ISBN 0-19-855493-1.
  2. ↑ McMahon, D. (2006). Quantum Mechanics Demystified (2nd ed.). Mc Graw Hill. ISBN 0 07 145546 9.
  3. ↑ Peleg, Y.; Pnini, R.; Zaarur, E.; Hecht, E. (2010). Quantum Mechanics (2nd ed.). McGraw Hill. ISBN 978-0071623582.