Polar space

In mathematics, in the field of geometry, a polar space of rank n (n ≥ 3), or projective index n − 1, consists of a set P, conventionally the set of points, together with certain subsets of P, called subspaces, that satisfy these axioms:

Every subspace, together with its own subspaces, is isomorphic with a projective geometry PG(d, q) with −1 ≤ d ≤ (n − 1) and q a prime power. By definition, for each subspace the corresponding d is its dimension.
The intersection of two subspaces is always a subspace.
For each point p not in a subspace A of dimension of n − 1, there is a unique subspace B of dimension n − 1 such that A ∩ B is (n − 2)-dimensional. The points in A ∩ B are exactly the points of A that are in a common subspace of dimension 1 with p.
There are at least two disjoint subspaces of dimension n − 1.

A polar space of rank two is a generalized quadrangle. Finite polar spaces (where P is a finite set) are also studied as combinatorial objects.

Examples

In PG(d, q), with d odd and d ≥ 3, the set of all points, with as subspaces the totally isotropic subspaces of an arbitrary symplectic polarity, forms a polar space of rank (d + 1)/2.
Let Q be a nonsingular quadric in PG(n, q) with character ω. Then the index of Q will be g = (n + w − 3)/2. The set of all points on the quadric, together with the subspaces on the quadric, forms a polar space of rank g + 1.
Let H be a nonsingular Hermitian variety in PG(n, q²). The index of H will be 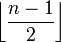$\left\lfloor \frac{n-1}{2}\right\rfloor$ . The points on H, together with the subspaces on it, form a polar space of rank $\left\lfloor\frac{n+1}{2}\right\rfloor$ .

Classification

Jacques Tits proved that a finite polar space of rank at least three, is always isomorphic with one of the three structures given above. This leaves only the problem of classifying generalized quadrangles.

References

Cameron, Peter J. (1991), Projective and polar spaces, QMW Maths Notes 13, London: Queen Mary and Westfield College School of Mathematical Sciences, MR 1153019