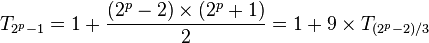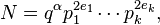Perfect number

In number theory, a perfect number is a positive integer that is equal to the sum of its proper positive divisors, that is, the sum of its positive divisors excluding the number itself (also known as its aliquot sum). Equivalently, a perfect number is a number that is half the sum of all of its positive divisors (including itself) i.e. σ₁(n) = 2n.

This definition is ancient, appearing as early as Euclid's Elements (VII.22) where it is called τέλειος ἀριθμός (perfect, ideal, or complete number). Euclid also proved a formation rule (IX.36) whereby 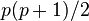is an even perfect number whenever is what is now called a Mersenne prime. Much later, Euler proved that all even perfect numbers are of this form.^[1] This is known as the Euclid–Euler theorem.

It is not known whether there are any odd perfect numbers, nor whether infinitely many perfect numbers exist.

Examples

The first perfect number is 6, because 1, 2, and 3 are its proper positive divisors, and 1 + 2 + 3 = 6. Equivalently, the number 6 is equal to half the sum of all its positive divisors: ( 1 + 2 + 3 + 6 ) / 2 = 6. The next perfect number is 28 = 1 + 2 + 4 + 7 + 14. This is followed by the perfect numbers 496 and 8128 (sequence A000396 in OEIS).

Discovery

These first four perfect numbers were the only ones known to early Greek mathematics, and the mathematician Nicomachus had noted 8128 as early as 100 AD.^[2] In a manuscript written between 1456 and 1461, an unknown mathematician recorded the earliest reference to a fifth perfect number, with 33,550,336 being correctly identified for the first time.^[3][4] In 1588, the Italian mathematician Pietro Cataldi identified the sixth (8,589,869,056)^[5] and the seventh (137,438,691,328) perfect numbers.^[6]

Even perfect numbers

List of unsolved problems in mathematics

Are there infinitely many perfect numbers?

Euclid proved that 2^p−1(2^p − 1) is an even perfect number whenever 2^p − 1 is prime (Euclid, Prop. IX.36).

For example, the first four perfect numbers are generated by the formula 2^p−1(2^p − 1), with p a prime number, as follows:

for p = 2:   2¹(2² − 1) = 6

for p = 3:   2²(2³ − 1) = 28

for p = 5:   2⁴(2⁵ − 1) = 496

for p = 7:   2⁶(2⁷ − 1) = 8128.

Prime numbers of the form 2^p − 1 are known as Mersenne primes, after the seventeenth-century monk Marin Mersenne, who studied number theory and perfect numbers. For 2^p − 1 to be prime, it is necessary that p itself be prime. However, not all numbers of the form 2^p − 1 with a prime p are prime; for example, 2¹¹ − 1 = 2047 = 23 × 89 is not a prime number.^[7] In fact, Mersenne primes are very rare—of the 9,592 prime numbers p less than 100,000,^[8] 2^p − 1 is prime for only 28 of them.

Over a millennium after Euclid, Ibn al-Haytham (Alhazen) circa 1000 AD conjectured that every even perfect number is of the form 2^p−1(2^p − 1) where 2^p − 1 is prime, but he was not able to prove this result.^[9] It was not until the 18th century that Leonhard Euler proved that the formula 2^p−1(2^p − 1) will yield all the even perfect numbers. Thus, there is a one-to-one relationship between even perfect numbers and Mersenne primes; each Mersenne prime generates one even perfect number, and vice versa. This result is often referred to as the Euclid–Euler theorem. As of April 2015, 48 Mersenne primes are known,^[10] and therefore 48 even perfect numbers (the largest of which is 2^57885160 × (2^57885161 − 1) with 34,850,340 digits).

An exhaustive search by the GIMPS distributed computing project has shown that the first 44 even perfect numbers are 2^p−1(2^p − 1) for

p = 2, 3, 5, 7, 13, 17, 19, 31, 61, 89, 107, 127, 521, 607, 1279, 2203, 2281, 3217, 4253, 4423, 9689, 9941, 11213, 19937, 21701, 23209, 44497, 86243, 110503, 132049, 216091, 756839, 859433, 1257787, 1398269, 2976221, 3021377, 6972593, 13466917, 20996011, 24036583, 25964951, 30402457, and 32582657 (sequence A000043 in OEIS).^[11]

Four higher perfect numbers have also been discovered, namely p = 37156667, 42643801, 43112609, and 57885161, though there may be others within this range. It is not known whether there are infinitely many perfect numbers, nor whether there are infinitely many Mersenne primes.

As well as having the form 2^p−1(2^p − 1), each even perfect number is the (2^p − 1)th triangular number (and hence equal to the sum of the integers from 1 to 2^p − 1) and the 2^p−1th hexagonal number. Furthermore, each even perfect number except for 6 is the ((2^p + 1)/3)th centered nonagonal number and is equal to the sum of the first 2^(p−1)/2 odd cubes:

Even perfect numbers (except 6) are of the form

with each resulting triangular number (after subtracting 1 from the perfect number and dividing the result by 9) ending in 3 or 5, the sequence starting with 3, 55, 903, 3727815, ....^[12] This can be reformulated as follows: adding the digits of any even perfect number (except 6), then adding the digits of the resulting number, and repeating this process until a single digit (called the digital root) is obtained, always produces the number 1. For example, the digital root of 8128 is 1, because 8 + 1 + 2 + 8 = 19, 1 + 9 = 10, and 1 + 0 = 1. This works with all perfect numbers 2^p−1(2^p − 1) with odd prime p and, in fact, with all numbers of the form 2^m−1(2^m − 1) for odd integer (not necessarily prime) m.

Owing to their form, 2^p−1(2^p − 1), every even perfect number is represented in binary as p ones followed by p − 1  zeros:

6₁₀ = 110₂

28₁₀ = 11100₂

496₁₀ = 111110000₂

8128₁₀ = 1111111000000₂

33550336₁₀ = 1111111111111000000000000₂.

Thus every even perfect number is a pernicious number.

Note that every even perfect number is also a practical number (c.f. Related concepts).

Odd perfect numbers

List of unsolved problems in mathematics

Are there any odd perfect numbers?

It is unknown whether there are any odd perfect numbers, though various results have been obtained. Carl Pomerance has presented a heuristic argument that suggests that no odd perfect numbers exist. All perfect numbers are also Ore's harmonic numbers, and it has been conjectured as well that there are no odd Ore's harmonic numbers other than 1.

Any odd perfect number N must satisfy the following conditions:

• N > 10¹⁵⁰⁰.^[13]
• N is not divisible by 105.^[14]
• N is of the form N ≡ 1 (mod 12), N ≡ 117 (mod 468), or N ≡ 81 (mod 324).^[15]
• N is of the form

where:

• q, p₁, ..., p_k are distinct primes (Euler).
• q ≡ α ≡ 1 (mod 4) (Euler).
• The smallest prime factor of N is less than (2k + 8) / 3.^[16]
• Either q^α > 10⁶², or p _j^2e_j  > 10⁶² for some j.^[13]
• N < 2^4k+1.^[17]

• The largest prime factor of N is greater than 10⁸.^[18]
• The second largest prime factor is greater than 10⁴, and the third largest prime factor is greater than 100.^[19][20]
• N has at least 101 prime factors and at least 9 distinct prime factors. If 3 is not one of the factors of N, then N has at least 12 distinct prime factors.^[13][21]

In 1888, Sylvester stated:^[22]

...a prolonged meditation on the subject has satisfied me that the existence of any one such [odd perfect number] — its escape, so to say, from the complex web of conditions which hem it in on all sides — would be little short of a miracle.

Minor results

All even perfect numbers have a very precise form; odd perfect numbers either do not exist or are rare. There are a number of results on perfect numbers that are actually quite easy to prove but nevertheless superficially impressive; some of them also come under Richard Guy's strong law of small numbers:

• The only even perfect number of the form x³ + 1 is 28 (Makowski 1962).
• 28 is also the only even perfect number that is a sum of two positive integral cubes (Gallardo 2010).^[23]
• The reciprocals of the divisors of a perfect number N must add up to 2 (to get this, take the definition of a perfect number, 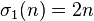, and divide both sides by n):
  • For 6, we have ;
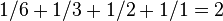  • For 28, we have , etc.
• The number of divisors of a perfect number (whether even or odd) must be even, because N cannot be a perfect square.
  • From these two results it follows that every perfect number is an Ore's harmonic number.
• The even perfect numbers are not trapezoidal numbers; that is, they cannot be represented as the difference of two positive non-consecutive triangular numbers. There are only three types of non-trapezoidal numbers: even perfect numbers, powers of two, and the numbers of the form 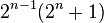formed as the product of a Fermat prime 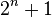with a power of two in a similar way to the construction of even perfect numbers from Mersenne primes.^[24]
• The number of perfect numbers less than n is less than 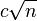, where c > 0 is a constant.^[25] In fact it is 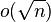, using little-o notation.^[26]
• Every even perfect number ends in 6 or 28, base ten.^[12]
• The only square-free perfect number is 6.^[27]

Related concepts

The sum of proper divisors gives various other kinds of numbers. Numbers where the sum is less than the number itself are called deficient, and where it is greater than the number, abundant. These terms, together with perfect itself, come from Greek numerology. A pair of numbers which are the sum of each other's proper divisors are called amicable, and larger cycles of numbers are called sociable. A positive integer such that every smaller positive integer is a sum of distinct divisors of it is a practical number.

By definition, a perfect number is a fixed point of the restricted divisor function s(n) = σ(n) − n, and the aliquot sequence associated with a perfect number is a constant sequence. All perfect numbers are also 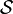-perfect numbers, or Granville numbers.

A semiperfect number is a natural number that is equal to the sum of all or some of its proper divisors. A semiperfect number that is equal to the sum of all its proper divisors is a perfect number. Most abundant numbers are also semiperfect; abundant numbers which are not semiperfect are called weird numbers.

See also

• Jan Brożek
• List of perfect numbers
• Superperfect numbers

Notes

References

Further reading

• Nankar, M.L.: "History of perfect numbers," Ganita Bharati 1, no. 1–2 (1979), 7–8.
• Hagis, P. (1973). "A Lower Bound for the set of odd Perfect Prime Numbers". Mathematics of Computation 27: 951–953. doi:10.2307/2005530. 
• Riele, H.J.J. "Perfect Numbers and Aliquot Sequences" in H.W. Lenstra and R. Tijdeman (eds.): Computational Methods in Number Theory, Vol. 154, Amsterdam, 1982, pp. 141–157.
• Riesel, H. Prime Numbers and Computer Methods for Factorisation, Birkhauser, 1985.
• Sándor, Jozsef; Crstici, Borislav (2004). Handbook of number theory II. Dordrecht: Kluwer Academic. pp. 15–98. ISBN 1-4020-2546-7. Zbl 1079.11001. 

External links

• Hazewinkel, Michiel, ed. (2001), "Perfect number", Encyclopedia of Mathematics, Springer, ISBN 978-1-55608-010-4 
• David Moews: Perfect, amicable and sociable numbers
• Perfect numbers – History and Theory
• Weisstein, Eric W., "Perfect Number", MathWorld.
• "Sloane's A000396 : Perfect numbers", The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
• OddPerfect.org A projected distributed computing project to search for odd perfect numbers.
• Great Internet Mersenne Prime Search
• Perfect Numbers, math forum at Drexel.
• Grimes, James. "8128: Perfect Numbers". Numberphile. Brady Haran. 

Divisibility-based sets of integers Overview Integer factorization Divisor Unitary divisor Divisor function Prime factor Fundamental theorem of arithmetic Arithmetic number Factorization forms Prime number Composite number Semiprime number Pronic number Sphenic number Square-free number Powerful number Perfect power Achilles number Smooth number Regular number Rough number Unusual number Constrained divisor sums Perfect number Almost perfect number Quasiperfect number Multiply perfect number Hemiperfect number Hyperperfect number Superperfect number Unitary perfect number Semiperfect number Practical number Erdős–Nicolas number With many divisors Abundant number Primitive abundant number Highly abundant number Superabundant number Colossally abundant number Highly composite number Superior highly composite number Weird number Aliquot sequence-related Untouchable number Amicable number Sociable number Betrothed number Other sets Deficient number Friendly number Solitary number Sublime number Harmonic divisor number Frugal number Equidigital number Extravagant number

Classes of natural numbers Powers and related numbers Achilles Power of two Power of 10 Square Cube Fourth power Fifth power Perfect power Powerful Prime power Of the form a × 2b ± 1 Cullen Double Mersenne Fermat Mersenne Proth Thabit Woodall Other polynomial numbers Carol Hilbert Idoneal Kynea Leyland Lucky numbers of Euler Repunit Recursively defined numbers Fibonacci Jacobsthal Leonardo Lucas Padovan Pell Perrin Possessing a specific set of other numbers Knödel Riesel Sierpinski Expressible via specific sums Nonhypotenuse Polite Practical Primary pseudoperfect Ulam Wolstenholme Generated via a sieve Lucky Code related Meertens Figurate numbers 2-dimensional centered Centered triangular Centered square Centered pentagonal Centered hexagonal Centered heptagonal Centered octagonal Centered nonagonal Centered decagonal Star non-centered Triangular Pentagonal Hexagonal Heptagonal Octagonal Nonagonal Decagonal Dodecagonal 3-dimensional centered Centered tetrahedral Centered cube Centered octahedral Centered dodecahedral Centered icosahedral non-centered Tetrahedral Octahedral Dodecahedral Icosahedral Stella octangula pyramidal Square pyramidal Pentagonal pyramidal Hexagonal pyramidal Heptagonal pyramidal 4-dimensional centered Centered pentachoric Squared triangular non-centered Pentatope Pseudoprimes Carmichael number Catalan pseudoprime Elliptic pseudoprime Euler pseudoprime Euler–Jacobi pseudoprime Fermat pseudoprime Frobenius pseudoprime Lucas pseudoprime Somer–Lucas pseudoprime Strong pseudoprime Combinatorial numbers Bell Cake Catalan Dedekind Delannoy Fuss–Catalan Lazy caterer's sequence Lobb Motzkin Narayana Ordered Bell Schröder Schröder–Hipparchus Arithmetic functions By properties of σ(n) Abundant Almost perfect Arithmetic Colossally abundant Descartes Hemiperfect Highly abundant Hyperperfect Multiply perfect Perfect Primitive abundant Quasiperfect Refactorable Sublime Superabundant Superior highly composite Superperfect By properties of Ω(n) Almost prime Semiprime By properties of φ(n) Highly cototient Highly totient Noncototient Nontotient Perfect totient Sparsely totient By properties of s(n) Amicable Betrothed Deficient Semiperfect Euclid Fortunate Dividing a quotient Wieferich Wall–Sun–Sun Wilson Other prime factor or divisor related numbers Blum Erdős–Woods Friendly Frugal Giuga Harmonic divisor Highly composite Lucas–Carmichael Pronic Regular Rough Smooth Sociable Sphenic Størmer Super-Poulet Zeisel Recreational mathematics Base-dependent numbers Automorphic Cyclic Digit-reassembly Dudeney Equidigital Extravagant Factorion Friedman Happy Harshad Kaprekar Keith Lychrel Missing-digit sum Narcissistic Palindromic Pandigital Parasitic Pernicious Polydivisible Primeval Repdigit Repunit Self Self-descriptive Smarandache–Wellin Strictly non-palindromic Strobogrammatic Sum-product Transposable Trimorphic Undulating Vampire Aronson's sequence Ban Pancake number