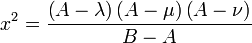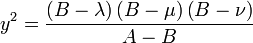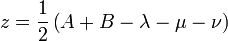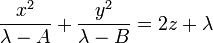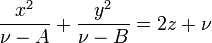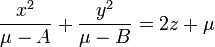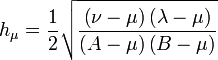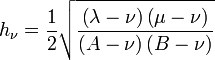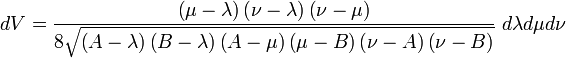Paraboloidal coordinates

Paraboloidal coordinates are a three-dimensional orthogonal coordinate system 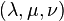$(\lambda, \mu, \nu)$ that generalizes the two-dimensional parabolic coordinate system. Similar to the related ellipsoidal coordinates, the paraboloidal coordinate system has orthogonal quadratic coordinate surfaces that are not produced by rotating or projecting any two-dimensional orthogonal coordinate system.

Coordinate surfaces of the three-dimensional paraboloidal coordinates.

Basic formulae

The Cartesian coordinates 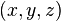$(x, y, z)$ can be produced from the ellipsoidal coordinates 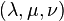$( \lambda, \mu, \nu )$ by the equations

x^{2} = \frac{\left( A - \lambda \right) \left( A - \mu \right) \left( A - \nu \right)}{B - A}

y^{2} = \frac{\left( B - \lambda \right) \left( B - \mu \right) \left( B - \nu \right)}{A - B}

z = \frac{1}{2} \left( A + B - \lambda - \mu -\nu \right)

where the following limits apply to the coordinates

\lambda < B < \mu < A < \nu

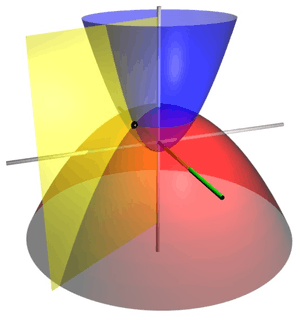
Consequently, surfaces of constant 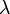$\lambda$ are elliptic paraboloids

\frac{x^{2}}{\lambda - A} + \frac{y^{2}}{\lambda - B} = 2z + \lambda

and surfaces of constant 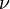$\nu$ are likewise

\frac{x^{2}}{\nu - A} + \frac{y^{2}}{\nu - B} = 2z + \nu

whereas surfaces of constant 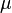$\mu$ are hyperbolic paraboloids

\frac{x^{2}}{\mu - A} + \frac{y^{2}}{\mu - B} = 2z + \mu

Scale factors

The scale factors for the paraboloidal coordinates 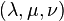$(\lambda, \mu, \nu )$ are

h_{\lambda} = \frac{1}{2} \sqrt{\frac{\left( \mu - \lambda \right) \left( \nu - \lambda \right)}{ \left( A - \lambda \right) \left( B - \lambda \right)}}

h_{\mu} = \frac{1}{2} \sqrt{\frac{\left( \nu - \mu \right) \left( \lambda - \mu \right)}{ \left( A - \mu \right) \left( B - \mu \right)}}

h_{\nu} = \frac{1}{2} \sqrt{\frac{\left( \lambda - \nu \right) \left( \mu - \nu \right)}{ \left( A - \nu \right) \left( B - \nu \right)}}

Hence, the infinitesimal volume element equals

dV = \frac{\left( \mu - \lambda \right) \left( \nu - \lambda \right) \left( \nu - \mu\right)}{8\sqrt{\left( A - \lambda \right) \left( B - \lambda \right) \left( A - \mu \right) \left( \mu - B \right) \left( \nu - A \right) \left( \nu - B \right) }} \ d\lambda d\mu d\nu

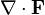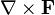Differential operators such as $\nabla \cdot \mathbf{F}$ and $\nabla \times \mathbf{F}$ can be expressed in the coordinates 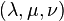$(\lambda, \mu, \nu)$ by substituting the scale factors into the general formulae found in orthogonal coordinates.

References

Bibliography

Morse PM, Feshbach H (1953). Methods of Theoretical Physics, Part I. New York: McGraw-Hill. p. 664. ISBN 0-07-043316-X. LCCN 52011515.
Margenau H, Murphy GM (1956). The Mathematics of Physics and Chemistry. New York: D. van Nostrand. pp. 184–185. LCCN 55010911.
Korn GA, Korn TM (1961). Mathematical Handbook for Scientists and Engineers. New York: McGraw-Hill. p. 180. LCCN 59014456. ASIN B0000CKZX7.
Arfken G (1970). Mathematical Methods for Physicists (2nd ed.). Orlando, FL: Academic Press. pp. 119–120.
Sauer R, Szabó I (1967). Mathematische Hilfsmittel des Ingenieurs. New York: Springer Verlag. p. 98. LCCN 67025285.
Zwillinger D (1992). Handbook of Integration. Boston, MA: Jones and Bartlett. p. 114. ISBN 0-86720-293-9. Same as Morse & Feshbach (1953), substituting u_k for ξ_k.
Moon P, Spencer DE (1988). "Paraboloidal Coordinates (μ, ν, λ)". Field Theory Handbook, Including Coordinate Systems, Differential Equations, and Their Solutions (corrected 2nd ed., 3rd print ed.). New York: Springer-Verlag. pp. 44–48 (Table 1.11). ISBN 978-0-387-18430-2.

External links

MathWorld description of confocal paraboloidal coordinates

Orthogonal coordinate systems

Two dimensional	Cartesian coordinate system Polar coordinate system Parabolic coordinate system Bipolar coordinates Elliptic coordinates

Three dimensional	Cartesian coordinate system Cylindrical coordinate system Spherical coordinate system Parabolic cylindrical coordinates Paraboloidal coordinates Oblate spheroidal coordinates Prolate spheroidal coordinates Ellipsoidal coordinates Elliptic cylindrical coordinates Toroidal coordinates Bispherical coordinates Bipolar cylindrical coordinates Conical coordinates 6-sphere coordinates Flat-ring cyclide coordinates Flat-disk cyclide coordinates Bi-cyclide coordinates Cap-cyclide coordinates Concave bi-sinusoidal single-centered coordinates Concave bi-sinusoidal double-centered coordinates Convex inverted-sinusoidal spherically aligned coordinates Quasi-random-intersection cartesian coordinates