Pappus configuration

In geometry, the Pappus configuration is a configuration of nine points and nine lines in the Euclidean plane, with three points per line and three lines through each point.[1]
It is named after Pappus of Alexandria; Pappus's hexagon theorem states that every two triples of collinear points ABC and abc (none of which lie on the intersection of the two lines) can be completed to form a Pappus configuration, by adding the six lines Ab, aB, Ac, aC, Bc, and bC, and their three intersection points X = Ab·aB, Y = Ac·aC, and Z = Bc·bC. These three points are the intersection points of the "opposite" sides of the hexagon AbCaBc. According to Pappus' theorem, the resulting system of nine points and eight lines always has a ninth line containing the three intersection points X, Y, and Z, called the Pappus line.[2]
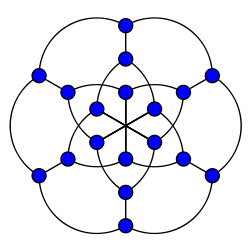
The Levi graph of the Pappus configuration is known as the Pappus graph. It is a bipartite symmetric cubic graph with 18 vertices and 27 edges.[3]

The Pappus configuration can also be derived from two triangles XcC and YbB that are in perspective with each other (the three lines through corresponding pairs of points meet at a single crossing point) in three different ways, together with their three centers of perspectivity Z, a, and A. The points of the configuration are the points of the triangles and centers of perspectivity, and the lines of the configuration are the lines through corresponding pairs of points. The Desargues configuration can also be defined in terms of perspective triangles, and the Reye configuration can be defined analogously from two tetrahedra that are in perspective with each other in four different ways, forming a desmic system of tetrahedra.
For any nonsingular cubic plane curve in the Euclidean plane, three real inflection points of the curve, and a fourth point on the curve, there is a unique way of completing these four points to form a Pappus configuration in such a way that all nine points lie on the curve.[4]
References
- ↑ Grünbaum, Branko (2009), Configurations of points and lines, Graduate Studies in Mathematics 103, Providence, RI: American Mathematical Society, p. xiv+399, ISBN 978-0-8218-4308-6, MR 2510707.
- ↑ Grünbaum (2009), p. 9.
- ↑ Grünbaum (2009), p. 28.
- ↑ Mendelsohn, N. S.; Padmanabhan, R.; Wolk, Barry (1987), "Some remarks on ”n”-clusters on cubic curves", in Colbourn, Charles J.; Mathon, R. A., Combinatorial Design Theory, Annals of Discrete Mathematics 34, Elsevier, pp. 371–378, doi:10.1016/S0304-0208(08)72903-7, ISBN 9780444703286, MR 920661.