Overring

In mathematics, an overring B of an integral domain A is a subring of the field of fractions K of A that contains A: i.e., 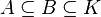A \subseteq B \subseteq K.[1] For instance, an overring of the integers is a ring in which all elements are rational numbers, such as the ring of dyadic rationals.

A typical example is given by localization: if S is a multiplicatively closed subset of A, then the localization S−1A is an overring of A. The rings in which every overring is a localization are said to have the QR property; they include the Bézout domains and are a subset of the Prüfer domains.[2] In particular, every overring of the ring of integers arises in this way; for instance, the dyadic rationals are the localization of the integers by the powers of two.

References

  1. ↑ Fontana, Marco; Papick, Ira J. (2002), "Dedekind and Prüfer domains", in Mikhalev, Alexander V.; Pilz, Günter F., The concise handbook of algebra, Kluwer Academic Publishers, Dordrecht, pp. 165–168.
  2. ↑ Fuchs, Laszlo; Heinzer, William; Olberding, Bruce (2004), "Maximal prime divisors in arithmetical rings", Rings, modules, algebras, and abelian groups, Lecture Notes in Pure and Appl. Math. 236, Dekker, New York, pp. 189–203, MR 2050712. See in particular p. 196.