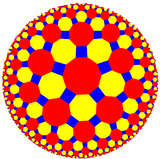
Order-4 pentagonal tiling
| Order-4 pentagonal tiling | |
|---|---|
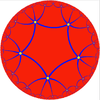
 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic regular tiling |
| Vertex figure | 5.5.5.5 |
| Schläfli symbol | {5,4} r{5,5} |
| Wythoff symbol | 4 | 5 2 2 | 5 5 |
| Coxeter diagram | |
| Symmetry group | [5,4], (*542) [5,5], (*552) |
| Dual | Order-5 square tiling |
| Properties | Vertex-transitive, edge-transitive, face-transitive |
In geometry, the order-4 pentagonal tiling is a regular tiling of the hyperbolic plane. It has Schläfli symbol of {5,4}. It can also be called a pentapentagonal tiling in a bicolored quasiregular form.
Symmetry
This tiling represents a hyperbolic kaleidoscope of 5 mirrors meeting as edges of a regular pentagon. This symmetry by orbifold notation is called *22222 with 5 order-2 mirror intersections. In Coxeter notation can be represented as [5*,4], removing two of three mirrors (passing through the pentagon center) in the [5,4] symmetry.
The kaleidoscopic domains can be seen as bicolored pentagons, representing mirror images of the fundamental domain. This coloring represents the uniform tiling t1{5,5} and as a quasiregular tiling is called a pentapentagonal tiling.
Related polyhedra and tiling
| Symmetry: [5,4], (*542) | [5,4]+, (542) | [5+,4], (5*2) | [5,4,1+], (*552) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
 |
 |
 |
 |
 |
 |
 |
 |
 |
 | |
| {5,4} | t{5,4} | r{5,4} | 2t{5,4}=t{4,5} | 2r{5,4}={4,5} | rr{5,4} | tr{5,4} | sr{5,4} | s{5,4} | h{4,5} | |
| Uniform duals | ||||||||||
 |
 |
 |
 |
 |
 |
 |
 |
 | ||
| V54 | V4.10.10 | V4.5.4.5 | V5.8.8 | V45 | V4.4.5.4 | V4.8.10 | V3.3.4.3.5 | V3.3.5.3.5 | V55 | |
| Symmetry: [5,5], (*552) | [5,5]+, (552) | ||||||
|---|---|---|---|---|---|---|---|
= |
= |
= |
= |
= |
= |
= |
= |
 |
 |
 |
 |
 |
 |
 |
 |
| {5,5} | t{5,5} |
r{5,5} | 2t{5,5}=t{5,5} | 2r{5,5}={5,5} | rr{5,5} | tr{5,5} | sr{5,5} |
| Uniform duals | |||||||
 |
 |
 |
 |
 |
 |
 |
|
| V5.5.5.5.5 | V5.10.10 | V5.5.5.5 | V5.10.10 | V5.5.5.5.5 | V4.5.4.5 | V4.10.10 | V3.3.5.3.5 |
This tiling is topologically related as a part of sequence of regular polyhedra and tilings with pentagonal faces, starting with the dodecahedron, with Schläfli symbol {5,n}, and Coxeter diagram ![]()
![]()
![]()
![]()
![]() , progressing to infinity.
, progressing to infinity.
 {5,3} |
 {5,4} |
 {5,5} |
 {5,6} |
 {5,7} |
This tiling is also topologically related as a part of sequence of regular polyhedra and tilings with four faces per vertex, starting with the octahedron, with Schläfli symbol {n,4}, and Coxeter diagram ![]()
![]()
![]()
![]()
![]() , with n progressing to infinity.
, with n progressing to infinity.
| Spherical | Euclidean | Hyperbolic tilings | ||||||
|---|---|---|---|---|---|---|---|---|
{2,4} |
 {3,4} |
 {4,4} |
 {5,4} |
 {6,4} |
 {7,4} |
 {8,4} |
... |  {∞,4} |
This tiling is topologically related as a part of sequence of regular polyhedra and tilings with vertex figure (4n).
| Finite | Euclidean | Compact hyperbolic | Paracompact | |||
|---|---|---|---|---|---|---|
 {4,3} |
 {4,4} |
 {4,5} |
 {4,6} |
 {4,7} |
 {4,8}... |
 {4,∞} |
| Symmetry *5n2 [n,5] |
Spherical | Hyperbolic... | Paracompact | Noncompact | ||||
|---|---|---|---|---|---|---|---|---|
| *352 [3,5] |
*452 [4,5] |
*552 [5,5] |
*652 [6,5] |
*752 [7,5] |
*852 [8,5]... |
*∞52 [∞,5] |
[iπ/λ,5] | |
| Coxeter | ||||||||
| Quasiregular figures configuration |
 5.3.5.3 |
 5.4.5.4 |
 5.5.5.5 |
 5.6.5.6 |
 5.7.5.7 |
 5.8.5.8 |
 5.∞.5.∞ |
5.∞.5.∞ |
| Dual figures | ||||||||
| Coxeter | ||||||||
| Dual (rhombic) figures configuration |
 V5.3.5.3 |
 V5.4.5.4 |
 V5.5.5.5 |
 V5.6.5.6 |
V5.7.5.7 |
V5.8.5.8 |
V5.∞.5.∞ |
V5.∞.5.∞ |
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
See also
| Wikimedia Commons has media related to Order-4 pentagonal tiling. |
- Square tiling
- Tilings of regular polygons
- List of uniform planar tilings
- List of regular polytopes
External links
- Weisstein, Eric W., "Hyperbolic tiling", MathWorld.
- Weisstein, Eric W., "Poincaré hyperbolic disk", MathWorld.
- Hyperbolic and Spherical Tiling Gallery
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
- Hyperbolic Planar Tessellations, Don Hatch
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
