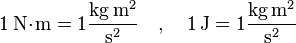Newton metre

The newton metre is a unit of torque (also called "moment") in the SI system. The symbolic form is N m or N·m.^[1] One newton metre, sometimes hyphenated newton-metre, is equal to the torque resulting from a force of one newton applied perpendicularly to a moment arm which is one metre long.

It is also used less commonly as a unit of work, or energy, in which case it is equivalent to the more common and standard SI unit of energy, the joule.^[2] In this very different usage the metre term represents the distance travelled or displacement in the direction of the force, and not the perpendicular distance from a fulcrum as it does when used to express torque. This usage is discouraged by the SI authority, since it can lead to confusion as to whether a given quantity expressed in newton-metres is a torque or a quantity of energy. However, since torque represents energy transferred or expended per angle of revolution, one newton metre of torque is equivalent to one joule per radian.^[3]

Newton metres and joules are "dimensionally equivalent" in the sense that they have the same expression in SI base units:

Again, N⋅m and J are distinguished in order to avoid misunderstandings where a torque is mistaken for an energy or vice versa. Similar examples of "dimensionally equivalent" units include Pa versus J/m³, Bq versus Hz, and ohms versus ohms per square.

Conversion factors

• 1 newton metre ≈ 0.7375621 pound-feet (often "foot-pounds")
• 1 kilogram-force metre = 9.80665 N·m^[4][5]
• 1 pound-foot (often "foot-pound") ≡ 1 pound-force-foot ≈ 1.3558 N·m
• 1 inch-ounce-force = 7.0615518 mN·m
• 1 dyne-centimetre = 10⁻⁷ N·m
• 1 joule = 1 N·m

See also

• Bending moment
• Spring scale
• Torque tester

References