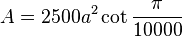Myriagon
| Regular myriagon | |
|---|---|
|
A regular myriagon | |
| Type | Regular polygon |
| Edges and vertices | 10000 |
| Schläfli symbol |
{10000} t{5000} |
| Coxeter diagram |
|
| Symmetry group | Dihedral (D10000), order 2×10000 |
| Internal angle (degrees) | 179.964° |
| Dual polygon | self |
| Properties | convex, cyclic, equilateral, isogonal, isotoxal |
In geometry, a myriagon is a polygon with 10000 sides. Several philosophers have used it to illustrate issues regarding thought.[1][2][3][4][5]
A regular myriagon is represented by Schläfli symbol {10000} and can be constructed as a quasiregular truncated 5000-gon, t{5000}, which alternates two types of edges.
Properties
The measure of each internal angle in a regular myriagon is 179.964°. The area of a regular chiliagon with sides of length a is given by
Because 10000 = 24 × 54, the number of sides is neither a product of distinct Fermat primes nor a power of two. Thus the regular myriagon is not a constructible polygon. Indeed, it is not even constructible with the use of neusis or an angle trisector, as the number of sides is neither a product of distinct Pierpont primes, nor a power of two, three, or six.
Myriagram
A myriagram is an 10000-sided star polygon. There are 1999 regular forms[6] given by Schläfli symbols of the form {10000/n}, where n is an integer between 2 and 5000 that is coprime to 10000. There are also 3000 regular star figures in the remaining cases.
See also
References
- ↑ Meditation VI by Descartes (English translation).
- ↑ Hippolyte Taine, On Intelligence: pp. 9–10
- ↑ Jacques Maritain, An Introduction to Philosophy: p. 108
- ↑ Alan Nelson (ed.), A Companion to Rationalism: p. 285
- ↑ Paolo Fabiani, The philosophy of the imagination in Vico and Malebranche: p. 222
- ↑ 5000 cases - 1 (convex) - 1000 (multiples of 5) - 2500 (multiples of 2)+ 500 (multiples of 2 and 5)
| ||||||||||||||||||||||||||