Moore plane
In mathematics, the Moore plane, also sometimes called Niemytzki plane (or Nemytskii plane, Nemytskii's tangent disk topology), is a topological space. It is a completely regular Hausdorff space (also called Tychonoff space) which is not normal. It is named after Robert Lee Moore and Viktor Vladimirovich Nemytskii.
Definition
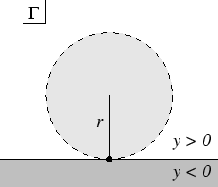
If  is the upper half-plane
is the upper half-plane  , then a topology may be defined on
, then a topology may be defined on 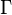 by taking a local basis
by taking a local basis 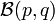 as follows:
as follows:
- Elements of the local basis at points
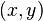 with
with 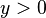 are the open discs in the plane which are small enough to lie within
are the open discs in the plane which are small enough to lie within 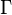 . Thus the subspace topology inherited by
. Thus the subspace topology inherited by  is the same as the subspace topology inherited from the standard topology of the Euclidean plane.
is the same as the subspace topology inherited from the standard topology of the Euclidean plane.
- Elements of the local basis at points
 are sets
are sets 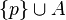 where A is an open disc in the upper half-plane which is tangent to the x axis at p.
where A is an open disc in the upper half-plane which is tangent to the x axis at p.
That is, the local basis is given by
Properties
- The Moore plane
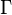 is separable, that is, it has a countable dense subset.
is separable, that is, it has a countable dense subset. - The Moore plane is a completely regular Hausdorff space (i.e. Tychonoff space), which is not normal.
- The subspace
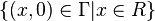 of
of 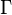 has, as its subspace topology, the discrete topology. Thus, the Moore plane shows that a subspace of a separable space need not be separable.
has, as its subspace topology, the discrete topology. Thus, the Moore plane shows that a subspace of a separable space need not be separable. - The Moore plane is first countable, but not second countable or Lindelöf.
- The Moore plane is not locally compact.
- The Moore plane is countably metacompact but not metacompact.
Proof that the Moore plane is not normal
The fact that this space M is not normal can be established by the following counting argument (which is very similar to the argument that the Sorgenfrey plane is not normal):
- On the one hand, the countable set
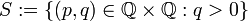 of points with rational coordinates is dense in M; hence every continuous function
of points with rational coordinates is dense in M; hence every continuous function 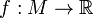 is determined by its restriction to
is determined by its restriction to 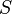 , so there can be at most
, so there can be at most  many continuous real-valued functions on M.
many continuous real-valued functions on M. - On the other hand, the real line
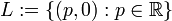 is a closed discrete subspace of M with
is a closed discrete subspace of M with 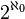 many points. So there are
many points. So there are 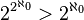 many continuous functions from L to
many continuous functions from L to 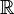 . Not all these functions can be extended to continuous functions on M.
. Not all these functions can be extended to continuous functions on M. - Hence M is not normal, because by the Tietze extension theorem all continuous functions defined on a closed subspace of a normal space can be extended to a continuous function on the whole space.
In fact, if X is a separable topological space having an uncountable closed discrete subspace, X cannot be normal.
See also
References
- Stephen Willard. General Topology, (1970) Addison-Wesley ISBN 0-201-08707-3.
- Steen, Lynn Arthur; Seebach, J. Arthur Jr. (1995) [1978], Counterexamples in Topology (Dover reprint of 1978 ed.), Berlin, New York: Springer-Verlag, ISBN 978-0-486-68735-3, MR 507446 (Example 82)
- Niemytzki plane at PlanetMath.org.
