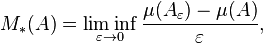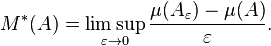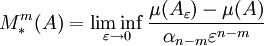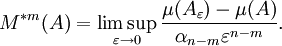Minkowski content

The Minkowski content of a set (named after Hermann Minkowski), or the boundary measure, is a basic concept in geometry and measure theory which generalizes to arbitrary measurable sets the notions of length of a smooth curve in the plane and area of a smooth surface in the space. It is typically applied to fractal boundaries of domains in the Euclidean space, but makes sense in the context of general metric measure spaces. It is related to, although different from, the Hausdorff measure.

Definition

Let 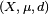\scriptstyle(X,\, \mu,\, d) be a metric measure space, where d is a metric on X and μ is a Borel measure. For a subset A of X and real ε > 0, let

A_\varepsilon = \{ x \in X \, | \, d(x, A) \leq \varepsilon \}

be the ε-extension of A. The lower Minkowski content of A is given by

M_*(A) = \liminf_{\varepsilon \to 0} \frac{\mu(A_\varepsilon) - \mu(A)}{\varepsilon},

and the upper Minkowski content of A is

M^*(A) = \limsup_{\varepsilon \to 0} \frac{\mu(A_\varepsilon) - \mu(A)}{\varepsilon}.

If M*(A) = M*(A), then the common value is called the Minkowski content of A associated with the measure μ, and is denoted by M(A).

Minkowski content in Rn

Let A be a subset of Rn. Then the m-dimensional Minkowski content of A is defined as follows. The lower content is

M_*^m(A) = \liminf_{\varepsilon \to 0} \frac{\mu(A_\varepsilon) - \mu(A)}{\alpha_{n-m}\varepsilon^{n-m}}

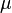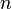where αn−m is the volume of the unit (n−m)-ball and \mu is n-dimensional Lebesgue measure. The upper content is

M^{*m}(A) = \limsup_{\varepsilon \to 0} \frac{\mu(A_\varepsilon) - \mu(A)}{\alpha_{n-m}\varepsilon^{n-m}}.

As before, if the upper and lower m-dimensional Minkowski content of A agree, then the Minkowski content of A, Mm(A), is defined to be this common value.

Properties

See also

References