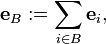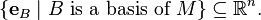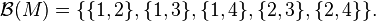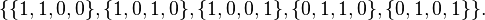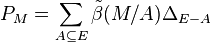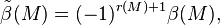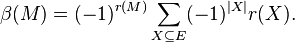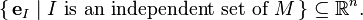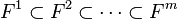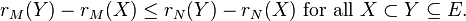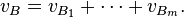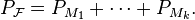Matroid polytope
In mathematics, a matroid polytope, also called a matroid basis polytope or basis matroid polytope to distinguish it from other polytopes derived from a matroid, is a polytope constructed via the bases of a matroid. Given a matroid 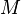 , the matroid polytope
, the matroid polytope  is the convex hull of the indicator vectors of the bases of
is the convex hull of the indicator vectors of the bases of 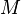 .
.
Definition
Let 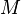 be a matroid on
be a matroid on 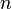 elements. Given a basis
elements. Given a basis 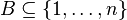 of
of 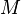 , the indicator vector of
, the indicator vector of 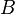 is
is
where  is the standard
is the standard 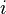 th unit vector in
th unit vector in 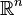 . The matroid polytope
. The matroid polytope  is the convex hull of the set
is the convex hull of the set
Examples


- Let
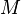 be the rank 2 matroid on 4 elements with bases
be the rank 2 matroid on 4 elements with bases
-
- That is, all 2-element subsets of
 except
except 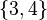 . The corresponding indicator vectors of
. The corresponding indicator vectors of 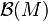 are
are
-
- The matroid polytope of
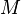 is
is -

- which is the square pyramid.
- Let
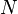 be the rank 2 matroid on 4 elements with bases that are all 2-element subsets of
be the rank 2 matroid on 4 elements with bases that are all 2-element subsets of  . The corresponding matroid polytope
. The corresponding matroid polytope 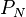 is the octahedron. Observe that the polytope
is the octahedron. Observe that the polytope  from the previous example is contained in
from the previous example is contained in 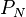 .
.
- If
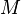 is the uniform matroid of rank
is the uniform matroid of rank  on
on 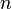 elements, then the matroid polytope
elements, then the matroid polytope  is the hypersimplex
is the hypersimplex 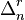 .[1]
.[1]
Properties
- A matroid polytope is contained in the hypersimplex
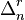 , where
, where  is the rank of the associated matroid and
is the rank of the associated matroid and 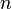 is the size of the ground set of the associated matroid.[2]
is the size of the ground set of the associated matroid.[2]
- Every edge of a matroid polytope
 is a parallel translate of
is a parallel translate of 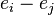 for some
for some 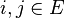 , the ground set of the associated matroid. In other words, the edges of
, the ground set of the associated matroid. In other words, the edges of  correspond exactly to the pairs of bases
correspond exactly to the pairs of bases 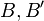 that satisfy the basis exchange property:
that satisfy the basis exchange property:  for some
for some 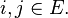 [2]
[2]
- Matroid polytopes are members of the family of generalized permutohedra.[3]
- Let
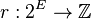 be the rank function of a matroid
be the rank function of a matroid 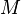 . The matroid polytope
. The matroid polytope  can be written uniquely as a signed Minkowski sum of simplices:[3]
can be written uniquely as a signed Minkowski sum of simplices:[3]
-
- where
 is the ground set of the matroid
is the ground set of the matroid 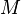 and
and 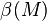 is the signed beta invariant of
is the signed beta invariant of 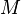 :
:
-
Related polytopes
Independence matroid polytope
The independence matroid polytope is the convex hull of the set
The (basis) matroid polytope is a face of the independence matroid polytope. Given the rank 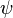 of a matroid
of a matroid 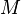 , the independence matroid polytope is equal to the polymatroid determined by
, the independence matroid polytope is equal to the polymatroid determined by 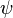 .
.
Flag matroid polytope
The flag matroid polytope is another polytope constructed from the bases of matroids. A flag 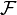 is a strictly increasing sequence
is a strictly increasing sequence
of finite sets.[4] Let  be the cardinality of the set
be the cardinality of the set  . Two matroids
. Two matroids 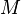 and
and 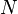 are said to be concordant if their rank functions satisfy
are said to be concordant if their rank functions satisfy
Given pairwise concordant matroids  on the ground set
on the ground set  with ranks
with ranks 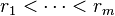 , consider the collection of flags
, consider the collection of flags 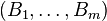 where
where 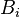 is a basis of the matroid
is a basis of the matroid  and
and 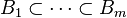 . Such a collection of flags is a flag matroid
. Such a collection of flags is a flag matroid 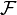 . The matroids
. The matroids  are called the constituents of
are called the constituents of 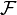 .
For each flag
.
For each flag 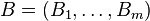 in a flag matroid
in a flag matroid 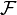 , let
, let 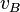 be the sum of the indicator vectors of each basis in
be the sum of the indicator vectors of each basis in 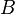
Given a flag matroid 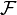 , the flag matroid polytope
, the flag matroid polytope  is the convex hull of the set
is the convex hull of the set
A flag matroid polytope can be written as a Minkowski sum of the (basis) matroid polytopes of the constituent matroids:[4]
References
- ↑ Grötschel, Martin (2004), "Cardinality homogeneous set systems, cycles in matroids, and associated polytopes", The Sharpest Cut: The Impact of Manfred Padberg and His Work, MPS/SIAM Ser. Optim., SIAM, Philadelphia, PA, pp. 99–120, MR 2077557. See in particular the remarks following Prop. 8.20 on p. 114.
- ↑ 2.0 2.1 Gelfand, I.M.; Goresky, R.M.; MacPherson, R.D.; Serganova, V.V. (1987). "Combinatorial geometries, convex polyhedra, and Schubert cells". Advances in Mathematics 63 (3): 301–316. doi:10.1016/0001-8708(87)90059-4.
- ↑ 3.0 3.1 Ardila, Federico; Benedetti, Carolina; Doker, Jeffrey (2010). "Matroid polytopes and their volumes". Discrete and Computational Geometry 43 (4): 841–854. arXiv:0810.3947. doi:10.1007/s00454-009-9232-9.
- ↑ 4.0 4.1 Borovik, Alexandre V.; Gelfand, I.M.; White, Neil (2013). "Coxeter Matroids". Progress in Mathematics 216. doi:10.1007/978-1-4612-2066-4.