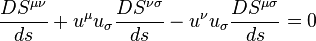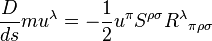Mathisson–Papapetrou–Dixon equations

In physics, specifically general relativity, the Mathisson–Papapetrou–Dixon equations describe the motion of a spinning massive object, moving in a gravitational field. Other equations with similar names and mathematical forms are the Mathisson-Papapetrou equations and Papapetrou-Dixon equations. All three sets of equations describe the same physics.

They are named for M. Mathisson,[1] W. G. Dixon,[2] and A. Papapetrou.[3]

Throughout, this article uses the natural units c = G = 1, and tensor index notation.

For a particle of mass m, the Mathisson–Papapetrou–Dixon equations are:[4][5]

\frac{D}{ds}\left(m u^\lambda + u_\mu \frac{DS^{\lambda\mu}}{ds} \right) = -\frac{1}{2}u^\pi S^{\rho\sigma} R^\lambda{}_{\pi\rho\sigma}

\frac{DS^{\mu\nu}}{ds} + u^\mu u_\sigma \frac{DS^{\nu\sigma}}{ds} - u^\nu u_\sigma \frac{DS^{\mu\sigma}}{ds} = 0

where: u is the four velocity (1st order tensor), S the spin tensor (2nd order), R the Riemann curvature tensor (4th order), and the capital "D" indicates the covariant derivative with respect to the particle's proper time s (an affine parameter).

Mathisson–Papapetrou equations

For a particle of mass m, the Mathisson–Papapetrou equations are:[6][7]

\frac{D}{ds}m u^\lambda = -\frac{1}{2}u^\pi S^{\rho\sigma} R^\lambda{}_{\pi\rho\sigma}

\frac{DS^{\mu\nu}}{ds} + u^\mu u_\sigma \frac{DS^{\nu\sigma}}{ds} - u^\nu u_\sigma \frac{DS^{\mu\sigma}}{ds} = 0

using the same symbols as above.

Papapetrou–Dixon equations


See also

References

Notes

  1. ↑ "Neue Mechanik materieller Systeme". Acta Phys. Polonica 6. 1937. pp. 163–209.
  2. ↑ W. G. Dixon (1970). "Dynamics of Extended Bodies in General Relativity. I. Momentum and Angular Momentum". Proc. R. Soc. Lond. A 314. doi:10.1098/rspa.1970.0020.
  3. ↑ A. Papapetrou (1951). "Spinning Test-Particles in General Relativity. I". Proc. R. Soc. Lond. A 209. doi:10.1098/rspa.1951.0200.
  4. ↑ R. Plyatsko, O. Stefanyshyn, M. Fenyk (2011). "Mathisson-Papapetrou-Dixon equations in the Schwarzschild and Kerr backgrounds". arXiv:1110.1967.
  5. ↑ R. Plyatsko, O. Stefanyshyn (2008). "On common solutions of Mathisson equations under different conditions". arXiv:0803.0121.
  6. ↑ R. M. Plyatsko, A. L. Vynar, Ya. N. Pelekh (1985). "Conditions for the appearance of gravitational ultrarelativistic spin-orbital interaction". Soviet Physics Journal 28 (10) (Springer). pp. 773–776.
  7. ↑ K. Svirskas, K. Pyragas (1991). "The spherically-symmetrical trajectories of spin particles in the Schwarzschild field". Astrophysics and Space Science 179 (2) (Springer). pp. 275–283.

Selected papers