Mathematics and art

Mathematics in art: Albrecht Dürer's copper plate engraving Melencolia I, 1514.

Mathematics and art have a long historical relationship. The ancient Egyptians and ancient Greeks knew about the golden ratio and regarded it as an aesthetically pleasing ratio. They may have incorporated it and other mathematical relationships, such as the 3:4:5 triangle, into the design of monuments including the Great Pyramid,[1] the Parthenon and the Colosseum.[2][3]

Artists who have been inspired by mathematics and studied mathematics as a means of complementing their works include the Greek sculptor Polykleitos, who prescribed a series of mathematical proportions for carving the ideal male nude. Renaissance painters including Piero della Francesca and Leonardo da Vinci made use of mathematics in their work. In modern times, artists like M. C. Escher use mathematical forms intensively, while new branches including Penrose tiles and fractal art have been developed.

Overview

Galileo Galilei in his Il Saggiatore wrote that “[The universe] is written in the language of mathematics, and its characters are triangles, circles, and other geometric figures.”[4] Artists who strive and seek to study nature must therefore first fully understand mathematics. On the other hand, mathematicians have sought to interpret and analyse art through the lens of geometry and rationality.

Ancient times

The Golden Ratio

The Golden Ratio, roughly equal to 1.618, was first formally introduced in text by Greek mathematician Pythagoras and later by Euclid in the 5th century BC. In the fourth century BC, Aristotle noted its aesthetic properties.[5] Aside from interesting mathematical properties, geometric shapes derived from the golden ratio, such as the golden rectangle, the golden triangle, and Kepler’s triangle, were believed to be aesthetically pleasing. As such, many works of ancient art exhibit and incorporate the golden ratio in their design. Various authors can discern the presence of the golden ratio in Egyptian, Summerian and Greek vases, Chinese pottery, Olmec sculptures, and Cretan and Mycenaean products from as early as the late Bronze Age.[6] The prevalence of this special number in art and architecture even before its formal discovery by Pythagoras is perhaps evidence of an instinctive and primal human cognitive preference for the golden ratio.[7]

Pyramids

Pyramid of Khufu.
Possible base:hypotenuse(b:a) ratios for the Pyramid of Khufu: 1:φ (Kepler’s Triangle), 3:5 (3-4-5 Triangle), and 1:4/π.

Evidence of mathematical influences in art is present in the Great Pyramids, built by Egyptian Pharaoh Khufu and completed in 2560BC. Pyramidologists since the nineteenth century have noted the presence of the golden ratio in the design of the ancient monuments. They note that the length of the base edges range from 755–756 feet while the height of the structure is 481.4 feet. Working out the math, the perpendicular bisector of the side of the pyramid comes out to 612 feet.[8] If we divide the slant height of the pyramid by half its base length, we get a ratio of 1.619, less than 1% from the golden ratio. This would also indicate that half the cross-section of the Khufu’s pyramid is in fact a Kepler’s triangle. Debate has broken out between prominent pyramidologists, including Temple Bell, Michael Rice, and John Taylor, over whether the presence of the golden ratio in the pyramids is due to design or chance. Rice contends that experts of Egyptian architecture have argued that ancient Egyptian architects knew the golden ratio. In addition, three other pyramidologists, Martin Gardner, Herbert Turnbull, and David Burton contend that:

Herodotus related in one passage that the Egyptian priests told him that the dimensions of the Great Pyramid were so chosen that the area of a square whose side was the height of the great pyramid equaled the area of the triangle.[9]

This passage, if true, would undeniably prove the intentional presence of the golden ratio in the pyramids. However, the assertion is questionable.[10] Critics of this golden ratio theory note that it is far more likely that the original Egyptian architects modeled the pyramid after the 3-4-5 triangle, rather than the Kepler’s triangle. The Rhind Mathematical Papyrus, the best example of Egyptian mathematics dating back to the Second Intermediate Period of Egypt, proves that the Egyptians used the 3-4-5 triangle extensively in mathematics and architecture. While Kepler's triangle has a face angle of 51°49’, the 3-4-5 triangle has a face angle of 53°8’, very close to the Kepler's triangle.[11] Another triangle that is close is one whose perimeter is 2π its height, such that the base to hypotenuse ratio is 1:4/π. With a face angle of 51°50’, it is also very similar to Kepler’s triangle. It remains unclear which triangle the Egyptians used when designing their pyramids.

Parthenon

The Parthenon is a temple dedicated the Greek goddess Athena, built in the 5th century BC on the Athenian Acropolis. It is contended that Phidias, the main Greek sculptor in charge of decorating the Parthenon, also knew about the golden ratio and its aesthetic properties. In fact, the Greek symbol for the Golden Ratio is named Phi (φ) because of Phidias.[12] The golden rectangle, a rectangle whose length to width ratio is the golden ratio and considered the most pleasing to the eye, is almost omnipresent in the façade and floor plans of the Parthenon. The entire façade may be enclosed within a golden rectangle.[13] The ratio of the length of a metope and triglyph to the height of the frieze, as well as the height of the columns and stylobate to the entire height of the temple is also the golden ratio. Phidias himself constructed many Parthenon statues that meticulously embody the golden ratio.[14] Phidias is also notable for his contributions to the Athena Parthenos and the Statue of Zeus. As with the Pyramids however, more recent historians challenge the purposeful inclusion of the golden ratio in Greek temples, such as the Parthenon, contending that earlier studies have purposefully fitted in measurements of the temple until it conformed to a golden rectangle.

Great Mosque of Kairouan

Floor plan of the Great Mosque of Kairouan.

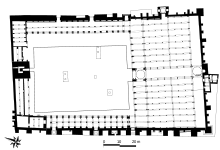
The oldest mosque in North Africa is the Great Mosque of Kairouan (Tunisia), built by Uqba ibn Nafi in 670 A.D. Boussora and Mazouz’s study of the mosque dimensions reveals a very consistent application of the golden ratio in its design. Boussora and Mazouz contend:

“ The geometric technique of construction of the golden section seems to have determined the major decisions of the spatial organisation. The golden section appears repeatedly in some part of the building measurements. It is found in the overall proportion of the plan and in the dimensioning of the prayer space, the court and the minaret. The existence of the golden section in some parts of Kairouan mosque indicates that the elements designed and generated with this principle may have been realised at the same period.[15] ”

Because of urban constraints, the mosque floor plan is not a perfect rectangle. Even so, for example, the division of the courtyard and prayer hall is almost a perfect golden ratio.

Polykleitos

Roman copy of Doryphoros, originally by Polykleitos.

Polykleitos the Elder (c.450–420 B.C.) was a Greek sculptor from the school of Argos, and a contemporary of Phidias. His works and statues consisted mainly of bronze and were of athletes. According to the mathematician Xenocrates, Polykleitos is ranked as one of the most important sculptors of Classical antiquity for his work on the Doryphorus and the statue of Hera in the Heraion of Argos.[16] While his sculptures may not be as famous as those of Phidias, he is better known for his approach towards sculpture. In the Canon of Polykleitos, a treatise he wrote designed to document the “perfect” anatomical proportions of the male nude, Polykleitos gives us a mathematical approach towards sculpturing the human body. The influence of the Canon of Polykleitos is immense both in Classical Greek, Roman, and Renaissance sculpture, with many sculptors after him following Polykleitos’ prescription. While none of Polykleitos’ original works survive, Roman copies of his works demonstrate and embody his ideal of physical perfection and mathematical precision.

Some scholars contend the influence of the mathematician Pythagoras on the Canon of Polykleitos.[17] The Canon applies the basic mathematical concepts of Greek geometry, such as the ratio, proportion, and symmetria (Greek for “harmonious proportions”) and turns it into a system capable of describing the human form through a series of continuous geometric progressions. Polykleitos starts with a specific human body part, the distal phalanges of the little finger, or the tip of the little finger to the first joint, and establishes that as the basic module or unit for determining all the other proportions of the human body.[18] From that, Polykleitos multiplies the length by radical 2 (1.14142) to get the distance of the second phalanges and multiplies the length again by radical 2 to get the length of the third phalanges. Next, he takes the finger length and multiplies it again by radical 2 to get the length of the palm from the base of the finger to the ulna. This geometric series of measurements progress until Polykleitos has formed the arm, chest, body, and so on. Other proportions are less set. For example, the ideal body should be 8 heads high and 2 heads wide. However, ordinary figures are 7½ heads tall while heroic figures are 8½ heads tall.

Renaissance

The Renaissance saw a rebirth of Classical Greek and Roman culture and ideas, among them the study of mathematics as a relevant subject needed to understand nature and the arts. Two major reasons drove Renaissance artists towards the pursuit of mathematics. First, painters needed to figure out how to depict three-dimensional scenes on a two-dimensional canvas. Second, philosophers and artists alike were convinced that mathematics was the true essence of the physical world and that the entire universe, including the arts, could be explained in geometric terms.[19] In light of these factors, Renaissance artists became some of the best applied mathematicians of their times.

Paolo Uccello

Italian painter Paolo Uccello (1397–1475) was fascinated by the study of perspective. A marble mosaic in the floor of the San Marco Basilica in Venice featuring the small stellated dodecahedron is attributed to Uccello.[20]

Piero della Francesca

Rays of light travel from an object to the eye. Where those rays originate from the picture plane, the object is drawn.
Piero della Francesca’s Flagellation of Christ showing Piero’s usage of linear perspective.

Piero della Francesca (c.1415–1492), an early Renaissance artist from Italy, exemplified this new shift in Renaissance thinking. Though chiefly appreciated for his art, he was an expert mathematician and geometer and authored many books on solid geometry and the emerging field of perspective, including De Prospectiva Pingendi (On Perspective for Painting), Trattato d’Abaco (Abacus Treatise), and De corporibus regularibus (Regular Solids).[21][22][23] Historian Vasari in the Lives of the Painters calls Piero the “greatest geometer of his time, or perhaps of any time.”[24] He was deeply interested in the theoretical study of perspective and this was apparent in many of his paintings, including the S. Agostino altarpiece and The Flagellation of Christ. His work on geometry influenced later mathematicians and artists, including Luca Pacioli in his De Divina Proportione and Leonardo da Vinci.

Piero began his study of classical mathematics and the works of the Greek mathematician Archimedes in the library at Urbino.[25] In addition to this classical training, Piero was taught commercial arithmetic in “abacus schools,” evidenced indirectly by his own writings which copies the format of abacus school textbooks.[26] It is possible therefore that he was influenced by the works of Leonardo Pisano (Fibonacci) from which those abacus textbooks were derived. Piero lived in the time when linear perspective was just being introduced in the artistic world. Leon Battista Alberti sums up the idea: “light rays travel in straight lines from points in the observed scene to the eye, forming a kind of pyramid with the eye as vertex.”[27] The painting therefore is a cross-sectional plane of that pyramid. The study of perspective precedes Piero and the Renaissance however. Before perspective, artists typically sized objects and figures according to their thematic importance. Perspective was first observed in 5th century B.C. Greece and Euclid’s Optics first introduced a mathematical theory of perspective. Muslim mathematician Alhazen extended the theory of optics in his Book of Optics in 1021 A.D., although he never applied these principals to art. Perspective first exploded onto the Renaissance artistic scene with Giotto di Bondone, who attempted to draw in perspective using an algebraic method to determine the placement of distant lines. In 1415, Italian architect Filippo Brunelleschi and his friend Leon Battista Alberti demonstrated the geometrical method of applying perspective in Florence, centered around the usage of similar triangles, a mathematical concept formulated long ago by Euclid, in determining the apparent height of distant objects.[28] However, Piero is the first painter to write a practical treatise for the application of this idea in art in his De Prospectiva Pingendi.

In De Prospectiva Pingendi, Piero painstakingly transforms art and his empirical observations into “vera scientia” (true science), i.e. into mathematical proofs. His treatise starts like any mathematics book in the vein of Euclid: he defines the point as “essere una costa tanto picholina quanto e possible ad ochio comprendere” (being the tiniest thing that is possible for the eye to comprehend).[19] From there, Piero uses a series of deductive logic to lead us, theorem by theorem, to the perspective representation of a three-dimensional body. Piero realized that the way aspects of a figure changed with the point of view obeyed precise and determinable mathematical laws. Piero methodically presented a series of perspective problems to gradually ease his reader from easy to increasingly complex problems. Mark Peterson explains:

In Book I, after some elementary constructions to introduce the idea of the apparent size of an object being actually its angle subtended at the eye, and referring to Euclid's Elements Books I and VI, and Euclid's Optics, he turns, in Proposition 13, to the representation of a square lying flat on the ground in front of the viewer. What should the artist actually draw? After this, objects are constructed in the square (tilings, for example, to represent a tiled floor), and corresponding objects are constructed in perspective; in Book II prisms are erected over these planar objects, to represent houses, columns, etc.; but the basis of the method is the original square, from which everything else follows.[29]

Notre Dame

Illustration of the Notre-Dame of Laon cathedral.

In his 1919 book Ad Quadratum, Frederik Macody Lund, a historian who studied the geometry of several gothic structures, claims that the Cathedral of Chartres (begun in the 12th century), the Notre-Dame of Laon (1157–1205), and the Notre Dame de Paris (1160) are designed according to the golden ratio.[30] According to Macody Lund, the superimposed regulator lines show that the cathedral has golden proportions. Other scholars argue that until Pacioli's 1509 publication, the golden ratio was unknown to artists and architects.[31]

Albrecht Dürer

Albrecht Dürer (1471–1528) was a German Renaissance printmaker who made important contributions to polyhedral literature in his book, Underweysung der Messung (Education on Measurement) (1525), meant to teach the subjects of linear perspective, geometry in architecture, Platonic solids, and regular polygons. Dürer was likely influenced by the works of Luca Pacioli and Piero della Francesca during his trips to Italy.[32] While the examples of perspective in Underweysung der Messung are underdeveloped and contain a number of inaccuracies, the manual does contain a very interesting discussion of polyhedra. Dürer is also the first to introduce in text the idea of polyhedral nets, polyhedra unfolded to lie flat for printing.[33] Dürer published another influential book on human proportions called Vier Bücher von Menschlicher Proportion (Four Books on Human Proportion) in 1528.

Dürer's well-known engraving Melencolia I depicts a frustrated thinker sitting by what is best interpreted as a “truncated rhomboid” or a “rhombohedron with 72-degree face angles, which has been truncated so it can be inscribed in a sphere”.[34] It has been the subject of more modern interpretation than almost any other print,[35] including a two-volume book by Peter-Klaus Schuster,[36] and an influential discussion in Erwin Panofsky's monograph of Dürer.[37]

De Divina Proportione

The first printed illustration of a rhombicuboctahedron, by Leonardo da Vinci, published in De divina proportione.

Written by Luca Pacioli in Milan from 1496–98, published in Venice in 1509, De Divina Proportione was about mathematical and artistic proportion. Leonardo da Vinci drew illustrations of regular solids in De divina proportione while he lived with and took mathematics lessons from Pacioli. Leonardo's drawings are probably the first illustrations of skeletonic solids, which allowed an easy distinction between front and back.[38] Skeletonic solids, such as the rhombicuboctahedron, were one of the first solids drawn to demonstrate perspective by being overlaid on top of each other. Additionally, the work also discusses the use of perspective by painters such as Piero della Francesca, Melozzo da Forlì, and Marco Palmezzano.

It is in De Divina Proportione that the golden ratio is defined as the divine proportion. Pacioli also details the use of the golden ratio as the mathematical definition of beauty when applied to the human face.

“The Ancients, having taken into consideration the rigorous construction of the human body, elaborated all their works, as especially their holy temples, according to these proportions; for they found here the two principal figures without which no project is possible: the perfection of the circle, the principle of all regular bodies, and the equilateral square.” from De Divina Proportione (1509)

Da Vinci

Woodcut from De Divina Proportione showing the golden ratio on a human face 
Rectangles superimposed on the Mona Lisa demonstrating golden ratio at work 
Leonardo’s Vitruvian Man 

In the 1490s, Leonardo da Vinci (1452–1519) trained under Luca Pacioli, preparing a series of drawings for De Divina Proportione. He studied Pacioli's Summa, from which he copied tables of proportions and multiplication tables.[39] Notably in Mona Lisa and The Last Supper, Leonardo’s work incorporated linear perspective. By making the lines in the painting converge on a single, invisible point on the horizon, the painting gains apparent depth. The vanishing point creates the illusion that the painting is an extension of the room itself.[40]

In Mona Lisa, the mismatch between the left and right backgrounds creates the illusion of perspective and depth. A Golden Rectangle whose base extends from her right wrist to her left elbow and reaches the very top of her head can be subdivided into smaller Golden Rectangles to produce a Golden Spiral. The edges of the new rectangles come to intersect the focal points of Mona Lisa: chin, eye, nose, and upturned corner of her mouth. The overall shape of the woman is a triangle with her arms as the base and her head as the tip, drawing attention to her face.[41]

In The Last Supper, Leonardo sought to create a perfect harmonic balance between the placement of the characters and the background. He did intensive studies on how the characters should be arranged at the table. The entire painting was constructed in a tight ratio of 12:6:4:3.[42] The entire piece measures 6 by 12 units. The wall in the back is equal to 4 units. The windows are 3 units and the recession of the tapestries on the side walls is 12:6:4:3.[43]

In Vitruvian Man, Leonardo expressed the ideas of the Roman architect Vitruvius.[44] Earlier artists and architects had illustrated Vitruvius' theory, but Leonardo's drawing differs in that the male figure adopts two different positions within the same image. He is simultaneously within the circle and the square; movement and liveliness are suggested by the figure's active arms and legs. The thin lines on his form show the significant points of the proportion scheme and indicate Leonardo’s concern with the architectural meaning of the work. Leonardo is representing the body as a building.

Modern times

M.C. Escher

Circle Limit III by M.C. Escher (1959).

Graphic artist M.C. Escher (1898—1972) was known for his mathematically inspired work.[45] Escher’s interest in tessellations, polyhedrons, shaping of space, and self-reference manifested itself in his work throughout his career.[46] In the Alhambra Sketch, Escher showed that art can be created with polygons or regular shapes such as triangles, squares, and hexagons. Escher used irregular polygons when tiling the plane and often used reflections, glide reflections, and translations to obtain many more patterns. Additionally, Escher arranged the shapes to simulate images of animals and other figures. His work can be noted in Development 1 and Cycles.

Escher’s was especially interested in five specific types of polyhedron, which appear many times in his work. "Regular polyhedrons" are defined as solids that have exactly similar polygonal faces, and are also known as Platonic solids. These tetrahedrons, cubes, octahedrons, dodecahedrons, and icosahedrons are especially prominent in Order and Chaos and Four Regular Solids.[47] Here these stellated figures often reside within another figure which further distorts the viewing angle and conformation of the polyhedrons and providing a multifaceted perspective artwork.[48] Additionally, Escher worked with the shape and logic of space in Three Intersecting Planes, Snakes, High and Low, and Waterfall.

Many of Escher's works contain impossible constructions, made using geometrical objects that cannot exist but are pleasant to the human sight. Some of Escher's tessellation drawings were inspired by conversations with the mathematician H. S. M. Coxeter concerning hyperbolic geometry.[49] Relationships between the works of mathematician Kurt Gödel, artist Escher, and composer Johann Sebastian Bach are explored in Gödel, Escher, Bach, a Pulitzer Prize-winning book.

Salvador Dalí

Dalí's 1954 painting Crucifixion (Corpus Hypercubus) uses the net of a hypercube.

Salvador Dalí (1904–1989) incorporated mathematical themes in several of his later works. His 1954 painting Crucifixion (Corpus Hypercubus) depicts a crucified figure upon the net of a hypercube. In The Sacrament of the Last Supper (1955) Christ and his disciples are pictured inside a giant dodecahedron. Dalí's last painting, The Swallow's Tail (1983), was part of a series inspired by René Thom's catastrophe theory.

Pablo Palazuelo

Pablo Palazuelo (1916–2007) was a contemporary Spanish painter and sculptor focused on the investigation of form. Heavily influenced by cubism and Paul Klee, Palazuelo developed a unique style that he described as the geometry of life and the geometry of all nature. Consisting of simple geometric shapes with detailed patterning and coloring, Palazuelo’s work was noted as powerful, attractive, unhesitant, enigmatic, and always new. From works such as Angular I to Automnes, Palazuelo expressed himself in geometric transformations and translations. Over time as Carmen Bonell notes, Palazuelo’s work evolved very rapidly toward an abstract-geometric language of increasing purity.[50]

John Robinson

John Robinson (1935–2007) was originally a sheep farmer who turned to sculpting. He began a serious sculpting career at the age of 35. Robinson was deeply interested in astronomy and mathematical relationships. According to Ronald Brown, Robinson’s work was extraordinary because of its proportion, line, rhythm, finish, the resonance of the titles and the forms, and because some of the complex forms, such as Rhythm of Life, had hardly been visualized in such an exact way. Robinson’s work from Gordian Knot to Bands of Friendship displayed highly complex mathematical knot theory in polished bronze for the public to see.[51]

Many mathematicians working in the field of topology and specifically with toruses see mathematical relationships in Robinson’s sculptures.

Rhythm of Life arose from experiments with wrapping a ribbon around an inner tube and finding it returned to itself.

Genesis evolved from an attempt at making Borromean rings-a set of three circles, no two of which link but in which the whole structure cannot be taken apart without breaking.

Many of Robinson’s works express the theme of common humanity. In Dependent Beings, the sculpture comprises a square that twists as it travels around the circle, giving it a boundary of two strips in contrasting textures.[52]

Helaman Ferguson

Sculptor Helaman Ferguson has made sculptures in various materials of a wide range of complex surfaces and other topological objects.[53] His work is motivated specifically by the desire to create visual representations of mathematical objects.

Ferguson has created a sculpture called The Eightfold Way at the Berkeley, California, Mathematical Sciences Research Institute based on the projective special linear group PSL(2,7), a finite group of 168 elements.[54][55]

Penrose tiles

A Penrose tiling.

Named after Roger Penrose, Penrose tiles are nonperiodic tiles generated from a simple base tile. In its simplest form, it consists of 36- and 72-degree rhombuses, with "matching rules" forcing the rhombuses to line up against each other only in certain patterns.[56] Penrose tiles lack translational symmetry due to its nonperiodicity, and any finite region in a tiling appears infinitely many times in the tiling.[57]

Both visually complex and simple at the same time, Penrose tiles arise from basic mathematical principles and can be viewed as intricately related to the golden ratio. Two notable relationships between Penrose tiles and the Golden ratio are:

  1. The ratio of thick to thin rhombuses in the infinite tile is the golden ratio 1.618.
  2. The distances between repeated patterns in the tiling grow as Fibonacci numbers when the size of the repetition increases.

Fractal art

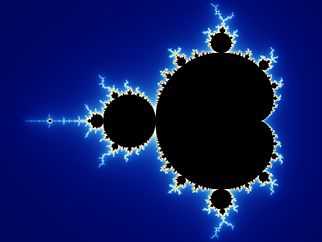
The Mandelbrot set, a common example of fractal art.
Main article: Fractal art

Fractal art is a form of algorithmic art created by calculating fractal objects and representing the results as still images, animations, and media which has developed from the mid-1980s onwards.[58] The Julia set and Mandelbrot sets are considered icons of fractal art.[59]

Platonic solids in art

The Platonic solids and other polyhedra are a recurring theme in Western art. Examples include:

See also

References

  1. ↑ The Great Pyramid, The Great Discovery, and The Great Coincidence, Mark Herkommer
  2. ↑ "Mathematical properties in ancient theatres and amphitheatres". Retrieved 29 January 2014.
  3. ↑ "Architecture: Ellipse?". The-Colosseum.net. Retrieved 29 January 2014.
  4. ↑ Galileo Galilei, The Assayer, as translated by Stillman Drake (1957), Discoveries and Opinions of Galileo, pp. 237–238.
  5. ↑ M.J. Seghers, The Golden Proportion and Beauty, Plastic and Reconstructive Surgery, Vol. 34, 1964.
  6. ↑ Klaus Mainzer, Symmetries of Nature: A Handbook for Philosophy of Nature and Science. Walter de Gruyter. pp. 118.
  7. ↑ Elam, Kimberly (2001). Geometry of Design: Studies in Proportion and Composition. Princeton Architectural Press. ISBN 9781568982496.
  8. ↑ Socrates G. Taseos, Back in Time 3104 B.C. to the Great Pyramid, SOC Publishers, Charlotte, NC, 1990.
  9. ↑ David M. Burton, The History of Mathematics: An Introduction, Allyn and Bacon, Boston, 1985.
  10. ↑ George Markowsky, Misconceptions about the Golden Ratio, College Mathematics Journal Vol 23, January 1992, pages 2–19.
  11. ↑ Midhat Gazale, Gnomon: From Pharaohs to Fractals, Princeton Univ. Press, 1999
  12. ↑ Christopher D. Green, A Review of Psychological Research on the Aesthetics of the Golden Section, Perception, 24, 937-968, 1995.
  13. ↑ H.E. Huntley, The Divine Proportion, Dover, New York, 1970.
  14. ↑ Hemenway, Priya (2005). Divine Proportion: Phi In Art, Nature, and Science. New York: Sterling. pp. p.96.
  15. ↑ Kenza Boussora and Said Mazouz, "The Use of the Golden Section in the Great Mosque of Kairouan", Nexus Network Journal, vol. 6 no. 1 (Spring 2004), pp. 7–16. doi:10.1007/s00004-004-0002-y
  16. ↑ Andrew Stewart, "Polykleitos of Argos," One Hundred Greek Sculptors: Their Careers and Extant Works, 16.73
  17. ↑ J.E. Raven, Polyclitus and Pythagoreanism, CQ, 1951, pp. 147–152.
  18. ↑ Richard Tobin, The Canon of Polykleitos, American Journal of Archaeology, Vol. 79, No. 4 (Oct., 1975), pp. 307–321.
  19. ↑ 19.0 19.1 Emmer, Michelle, Visual Mind, Volume 2, Section 3, MIT Press 2005
  20. ↑ 20.0 20.1 20.2 20.3 20.4 Polyhedra in Art, George W. Hart
  21. ↑ Piero della Francesca, De Prospectiva Pingendi, ed. G. Nicco Fasola, 2 vols., Florence (1942).
  22. ↑ Piero della Francesca, Trattato d'Abaco, ed. G. Arrighi, Pisa (1970).
  23. ↑ Piero della Francesca, L'opera "De corporibus regularibus" di Pietro Franceschi detto della Francesca usurpata da Fra Luca Pacioli, ed. G. Mancini, Rome, (1916).
  24. ↑ G. Vasari, Le Opere, ed. G. Milanesi, vol. 2, Florence (1878), 490.
  25. ↑ T.L. Heath, The Thirteen Books of Euclid's Elements, Cambridge University Press (1908), 97.
  26. ↑ P. Grendler, "What Piero Learned in School: Fifteenth-Century Vernacular Education," in Piero della Francesca and His Legacy, ed. M.A. Lavin, University Press of New England (1995), 161–176.
  27. ↑ Leon Battista Alberti, On Painting, ed. Martin Kemp, trans. Cecil Grayson, London-New York (1991).
  28. ↑ Vasari's Lives of the Artists Chapter on Brunelleschi
  29. ↑ The Geometry of Piero della Francesca by Mark A. Peterson
  30. ↑ CHANFÓN OLMOS, Carlos. Curso sobre Proporción. Procedimientos reguladors en construcción. Convenio de intercambio UNAM–UADY. México – Mérica, 1991
  31. ↑ Livio, Mario (2002). The Golden Ratio: The Story of Phi, The World's Most Astonishing Number. New York: Broadway Books. ISBN 0-7679-0815-5.
  32. ↑ E. Panofsky, The Life and Art of Albrecht Durer, Princeton, 1955.
  33. ↑ George W. Hart. "Durer’s Polyhedra". Georgehart.com. Retrieved 2009-08-13.
  34. ↑ P. Schreiber, "A New Hypothesis on Durer's Enigmatic Polyhedron in His Copper Engraving 'Melencolia I'," Historia Mathematica, 26, pp. 369–377, 1999.
  35. ↑ Dodgson, Campbell (1926). Albrecht Dürer. London: Medici Society. p. 94.
  36. ↑ Schuster, Peter-Klaus (1991). MELENCOLIA I: Dürers Denkbild. Berlin: Gebr. Mann Verlag. pp. 17–83.
  37. ↑ Panofsky, Erwin; Klibansky, Raymond; Saxl, Fritz (1964). Saturn and melancholy. New York: Basic Books, Inc.
  38. ↑ "George Hart Luca Pacioli's Polyhedra". Georgehart.com. Retrieved 2009-08-13.
  39. ↑ "Calter, Paul. Geometry and Art Unit 1". Dartmouth.edu. Retrieved 2009-08-13.
  40. ↑ Brizio, Anna Maria, The Painter. Leonardo: The Artist, McGraw-Hill Co., 1980.
  41. ↑ "Matt Anderson, Jeffrey Frazier, and Kris Popendorf. Leonardo da Vinci". Library.thinkquest.org. Retrieved 2009-08-13.
  42. ↑ Melissa Kimich. "Leonardo Da Vinci's Connection with Mathematics" (PDF). Retrieved 2011-12-19.
  43. ↑ Turner, Richard A., Inventing Leonardo. Alfred A. Knopf, 1992.
  44. ↑ "Vitruvian Man". Davincilife.com. 2006-10-18. Retrieved 2009-08-13.
  45. ↑ "MC Escher". Mathacademy.com. 2007-11-01. Retrieved 2009-08-13.
  46. ↑ "Tour: M.C. Escher – Life and Work". Nga.gov. Retrieved 2009-08-13.
  47. ↑ MC Escher. The World of MC Escher, Random House, 1988.
  48. ↑ MC Escher, JW Vermeulen, K Ford. Escher on Escher: Exploring the Infinite. 1989, HN Abrams.
  49. ↑ Siobhan Roberts. King of Infinite Space. Chapter 11 "Coxetering" with M.C. Escher.
  50. ↑ Emmer, Michelle, Visual Mind, Volume 2, Essay 5, MIT Press 2005
  51. ↑ Emmer, Michelle, Visual Mind, Volume 2, Essay 6, MIT Press 2005
  52. ↑ "John Robinson/Edition Limitee 2007". Bradshawfoundation.com. Retrieved 2009-08-13.
  53. ↑ "Helaman Ferguson web site". Helasculpt.com. Retrieved 2009-08-13.
  54. ↑ The Eightfold Way: A Mathematical Sculpture by Helaman Ferguson, William P. Thurston
  55. ↑ "MAA book review of ''The Eightfold Way: The Beauty of Klein's Quartic Curve''". Maa.org. 1993-11-14. Retrieved 2009-08-13.
  56. ↑ "Penrose Tiles". Ics.uci.edu. Retrieved 2009-08-13.
  57. ↑ Penrose, R., The role of aesthetics in pure and applied mathematical research, Bull. Inst. Math. Appl. 10, (1974), 266–271.
  58. ↑ Bovill, Carl (1996). Fractal geometry in architecture and design. Boston: Birkhauser. p. 153. ISBN 0-8176-3795-8.
  59. ↑ Burger, Edward B.; Michael P. Starbird (2005). The heart of mathematics: an invitation to effective thinking. Springer. p. 475. ISBN 1-931914-41-9.

External links

Wikimedia Commons has media related to Mathematics in art.