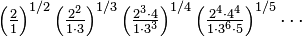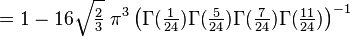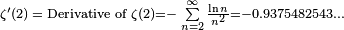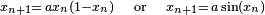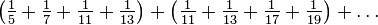Mathematical constants and functions
A mathematical constant is a number, which has a special meaning for calculations. For example, the constant π means the ratio of the length of a circle's circumference to its diameter. This value is always the same for any circle.
Tables structure
- Value numerical of the constant and link to MathWorld or to OEIS Wiki.
- LaTeX: Formula or series in TeX format.
- Formula: For use in program Wolfram Alpha.
- OEIS: On-Line Encyclopedia of Integer Sequences.
- Continued fraction: In the simple form [to integer; frac1, frac2, frac3, ...], overline if periodic.
- Year: Discovery of the constant, or dates of the author.
- Web format: Value in appropriate format for web browsers.
- Nº: Number types.
- R – Rational number
- I – Irrational number
- A – Algebraic number
- T – Transcendental number
- C – Complex number
Table of constants and functions
You can choose the order of the list by clicking on the name, value, OEIS, etc..
| Value | Name | Graphics | Symbol | LaTeX | Formula | Nº | OEIS | Continued fraction | Year | Web format |
|---|---|---|---|---|---|---|---|---|---|---|
| 1.11786 41511 89944 97314 [Mw 1] | Goh-Schmutz Constant [1] | 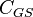 |
 |
Integrate{ log(s+1) /(E^s-1)} |
[1;8,2,15,2,7,2,1,1,1,1,2,3,5,3,5,1,1,4,13,1,...] | 1.11786415118994497314040996202656544 | ||||
| 0.31813 15052 04764 13531 ±1.33723 57014 30689 40 i [Ow 1] |
Fixed points Super-Logarithm [2] · [3] |
 |
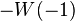 |
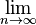 
For an initial value of x different to 0, 1, e, e^e, e^(e^e), etc. |
-W(-1) where W=ProductLog Lambert W function |
C | [-i;1 +2i,1+i,6-i,1+2i,-7+3i,2i,2,1-2i,-1+i,-, ...] | 0.31813150520476413531265425158766451 -1.33723570143068940890116214319371 i | ||
| 0.28016 94990 23869 13303 [Mw 2] | Bernstein's constant [4] |  |
 |
1/(2 sqrt(pi)) | T | [0;3,1,1,3,9,6,3,1,3,14,34,2,1,1,60,2,2,1,1,...] | 1913 | 0.28016949902386913303643649123067200 | ||
| 0.66016 18158 46869 57392 [Mw 3] | Twin Primes Constant [5] |  |
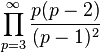 |
prod[p=3 to ∞] {p(p-2)/(p-1)^2 |
[0;1,1,1,16,2,2,2,2,1,18,2,2,11,1,1,2,4,1,...] | 1922 | 0.66016181584686957392781211001455577 | |||
| 1.22674 20107 20353 24441 [Mw 4] | Fibonacci Factorial Constant [6] | 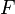 |
 |
prod[n=1 to ∞] {1-((sqrt(5) -3)/2)^n} |
[1;4,2,2,3,2,15,9,1,2,1,2,15,7,6,21,3,5,1,23,...] | 1.22674201072035324441763023045536165 | ||||
| 0.11494 20448 53296 20070 [Mw 5] | Kepler–Bouwkamp constant [7] |  |
 |
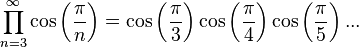 |
prod[n=3 to ∞] {cos(pi/n)} |
[0;8,1,2,2,1,272,2,1,41,6,1,3,1,1,26,4,1,1,...] | 0.11494204485329620070104015746959874 | |||
| 1.78723 16501 82965 93301 [Mw 6] | Komornik–Loreti constant [8] | 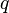 |
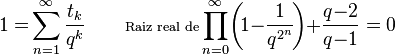
t k = Thue–Morse sequence |
FindRoot[(prod[n=0 to ∞] {1-1/(x^2^n)}+ (x-2)/(x-1))= 0, {x, 1.7}, WorkingPrecision->30] |
T | [1;1,3,1,2,3,188,1,12,1,1,22,33,1,10,1,1,7,...] | 1998 | 1.78723165018296593301327489033700839 | ||
| 3.30277 56377 31994 64655 [Mw 7] | Bronze ratio [9] | 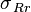 |
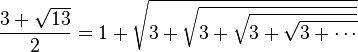 |
(3+sqrt 13)/2 | A | [3;3,3,3,3,3,3,3,3,3,3,3,3,3,3,3,3,3,3,...] = [3;3,...] |
3.30277563773199464655961063373524797 | |||
| 0.82699 33431 32688 07426 [Mw 8] | Disk Covering [10] |  |
 |
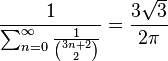 |
3 Sqrt[3]/(2 Pi) | T | [0;1,4,1,3,1,1,4,1,2,2,1,1,7,1,4,4,2,1,1,1,1,...] | 1939 1949 |
0.82699334313268807426698974746945416 | |
| 2.66514 41426 90225 18865 [Mw 9] | Gelfond–Schneider constant [11] | 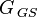 |
 |
2^sqrt{2} | T | [2;1,1,1,72,3,4,1,3,2,1,1,1,14,1,2,1,1,3,1,...] | 1934 | 2.66514414269022518865029724987313985 | ||
| 3.27582 29187 21811 15978 [Mw 10] | Khinchin-Lévy constant [12] |  |
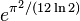 |
e^(\pi^2/(12 ln(2)) | [3;3,1,1,1,2,29,1,130,1,12,3,8,2,4,1,3,55,...] | 1936 | 3.27582291872181115978768188245384386 | |||
| 0.52382 25713 89864 40645 [Mw 11] | Chi Function Hyperbolic cosine integral |
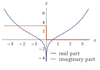 |
 |
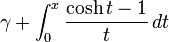
|
Chi(x) | [0;1,1,9,1,172,1,7,1,11,1,1,2,1,8,1,1,1,1,1,...] | 0.52382257138986440645095829438325566 | |||
| 1.13198 82487 943 [Mw 12] | Viswanath Constant[13] | 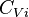 |
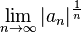 where an = Fibonacci sequence where an = Fibonacci sequence |
lim_(n->∞) |a_n|^(1/n) |
T ? | [1;7,1,1,2,1,3,2,1,2,1,8,1,5,1,1,1,9,1,...] | 1997 | 1.1319882487943 ... | ||
| 1.23370 05501 36169 82735 [Mw 13] | Favard constant [14] | 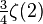 |
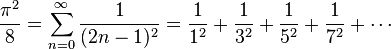 |
sum[n=1 to ∞] {1/((2n-1)^2)} |
T | [1;4,3,1,1,2,2,5,1,1,1,1,2,1,2,1,10,4,3,1,1,...] | 1902 a 1965 |
1.23370055013616982735431137498451889 | ||
| 2.50662 82746 31000 50241 | Square root of 2 pi |  |
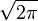 |
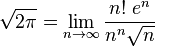 Stirling's approximation Stirling's approximation |
sqrt (2 pi) | T | [2;1,1,37,4,1,1,1,1,9,1,1,2,8,6,1,2,2,1,3,...] | 1692 a 1770 |
2.50662827463100050241576528481104525 | |
| 4.13273 13541 22492 93846 | Square root of Tau·e | 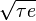 |
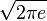 |
sqrt(2 pi e) | [4;7,1,1,6,1,5,1,1,1,8,3,1,2,2,15,2,1,1,2,4,...] | 4.13273135412249293846939188429985264 | ||||
| 0.97027 01143 92033 92574 [Mw 14] | Lochs constant [15] | 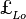 |
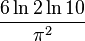 |
6*ln(2)*ln(10)/Pi^2 | [0;1,32,1,1,1,2,1,46,7,2,7,10,8,1,71,1,37,1,1,...] | 1964 | 0.97027011439203392574025601921001083 | |||
| 0.98770 03907 36053 46013 [Mw 15] | Area bounded by the eccentric rotation of Reuleaux triangle [16] |
 |
 |
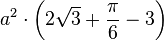 where a= side length of the square where a= side length of the square |
2 sqrt(3)+pi/6-3 | T | [0;1,80,3,3,2,1,1,1,4,2,2,1,1,1,8,1,2,10,1,2,...] | 1914 | 0.98770039073605346013199991355832854 | |
| 0.70444 22009 99165 59273 | Carefree constant 2 [17] | 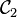 |
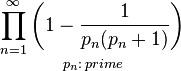 |
N[prod[n=1 to ∞] {1 - 1/(prime(n)* (prime(n)+1))}] |
[0;1,2,2,1,1,1,1,4,2,1,1,3,703,2,1,1,1,3,5,1,...] | 0.70444220099916559273660335032663721 | ||||
| 1.84775 90650 22573 51225 [Mw 16] | Connective constant [18][19] |  |
 |
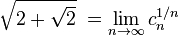
as a root of the polynomial |
sqrt(2+sqrt(2)) | A | [1;1,5,1,1,3,6,1,3,3,10,10,1,1,1,5,2,3,1,1,3,...] | 1.84775906502257351225636637879357657 | ||
| 0.30366 30028 98732 65859 [Mw 17] | Gauss-Kuzmin-Wirsing constant [20] | 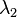 |
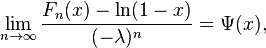
where |
[0;3,3,2,2,3,13,1,174,1,1,1,2,2,2,1,1,1,2,2,1,...] | 1973 | 0.30366300289873265859744812190155623 | ||||
| 1.57079 63267 94896 61923 [Mw 18] | Favard constant K1 Wallis product [21] |
 |
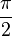 |
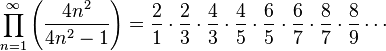 |
Prod[n=1 to ∞] {(4n^2)/(4n^2-1)} |
T | [1;1,1,3,31,1,145,1,4,2,8,1,6,1,2,3,1,4,1,5,1...] | 1655 | 1.57079632679489661923132169163975144 | |
| 1.60669 51524 15291 76378 [Mw 19] | Erdős–Borwein constant[22][23] |  |
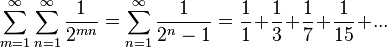 |
sum[n=1 to ∞] {1/(2^n-1)} |
I | [1;1,1,1,1,5,2,1,2,29,4,1,2,2,2,2,6,1,7,1,...] | 1949 | 1.60669515241529176378330152319092458 | ||
| 1.61803 39887 49894 84820 [Mw 20] | Phi, Golden ratio [24] |  |
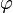 |
 |
(1+5^(1/2))/2 | A | [0;1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,1,...] = [0;1,...] |
-300 ~ | 1.61803398874989484820458633436563812 | |
| 1.64493 40668 48226 43647 [Mw 21] | Riemann Function Zeta(2) | 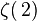 |
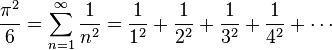 |
Sum[n=1 to ∞] {1/n^2} |
T | [1;1,1,1,4,2,4,7,1,4,2,3,4,10 1,2,1,1,1,15,...] | 1826 to 1866 |
1.64493406684822643647241516664602519 | ||
| 1.73205 08075 68877 29352 [Mw 22] | Theodorus constant[25] |  |
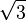 |
![\sqrt[3]{3 \,\sqrt[3]{3 \, \sqrt[3]{3 \,\sqrt[3]{3 \,\sqrt[3]{3 \,\cdots}}}}}](../I/m/e9b71e9d16ee19d82d83df7370bfae7f.png) |
(3(3(3(3(3(3(3) ^1/3)^1/3)^1/3) ^1/3)^1/3)^1/3) ^1/3 ... |
A | [1;1,2,1,2,1,2,1,2,1,2,1,2,1,2,1,2,1,2,1,2,...] = [1;1,2,...] |
-465 to -398 |
1.73205080756887729352744634150587237 | |
| 1.75793 27566 18004 53270 [Mw 23] | Kasner number |  |
 |
Fold[Sqrt[#1+#2] &,0,Reverse [Range[20]]] |
[1;1,3,7,1,1,1,2,3,1,4,1,1,2,1,2,20,1,2,2,...] | 1878 a 1955 |
1.75793275661800453270881963821813852 | |||
| 2.29558 71493 92638 07403 [Mw 24] | Universal parabolic constant [26] | 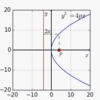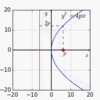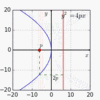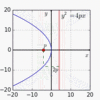 |
 |
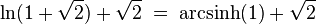 |
ln(1+sqrt 2)+sqrt 2 | T | [2;3,2,1,1,1,1,3,3,1,1,4,2,3,2,7,1,6,1,8,7,2,1,...] | 2.29558714939263807403429804918949038 | ||
| 1.78657 64593 65922 46345 [Mw 25] | Silverman constant[27] |  |
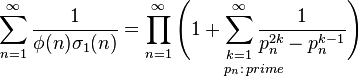 |
Sum[n=1 to ∞] {1/[EulerPhi(n) DivisorSigma(1,n)]} |
[1;1,3,1,2,5,1,65,11,2,1,2,13,1,4,1,1,1,2,5,4,...] | 1.78657645936592246345859047554131575 | ||||
| 2.59807 62113 53315 94029 [Mw 26] | Area of the regular hexagon with side equal to 1 [28] | 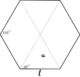 |
 |
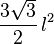 |
3 sqrt(3)/2 | A | [2;1,1,2,20,2,1,1,4,1,1,2,20,2,1,1,4,1,1,2,20,...] [2;1,1,2,20,2,1,1,4] |
2.59807621135331594029116951225880855 | ||
| 0.66131 70494 69622 33528 [Mw 27] | Feller-Tornier constant [29] |
 |
 |
[prod[n=1 to ∞] {1-2/prime(n)^2}] /2 + 1/2 |
T ? | [0;1,1,1,20,9,1,2,5,1,2,3,2,3,38,8,1,16,2,2,...] | 1932 | 0.66131704946962233528976584627411853 | ||
| 1.46099 84862 06318 35815 [Mw 28] | Baxter's Four-coloring constant [30] |
Mapamundi |
 |
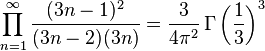
|
3×Gamma(1/3) ^3/(4 pi^2) |
[1;2,5,1,10,8,1,12,3,1,5,3,5,8,2,1,23,1,2,161,...] | 1970 | 1.46099848620631835815887311784605969 | ||
| 1.92756 19754 82925 30426 [Mw 29] | Tetranacci constant |  |
Positive root of  |
Root[x+x^-4-2=0] | A | [1;1,12,1,4,7,1,21,1,2,1,4,6,1,10,1,2,2,1,7,1,...] | 1.92756197548292530426190586173662216 | |||
| 1.00743 47568 84279 37609 [Mw 30] | DeVicci's tesseract constant |  |
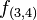 |
The largest cube that can pass through in an 4D hypercube.
Positive root of |
Root[4*x^8-28*x^6 -7*x^4+16*x^2+16 =0] |
A | [1;134,1,1,73,3,1,5,2,1,6,3,11,4,1,5,5,1,1,48,...] | 1.00743475688427937609825359523109914 | ||
| 1.70521 11401 05367 76428 [Mw 31] | Niven's constant [31] |  |
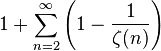 |
1+ Sum[n=2 to ∞] {1-(1/Zeta(n))} |
[1;1,2,2,1,1,4,1,1,3,4,4,8,4,1,1,2,1,1,11,1,...] | 1969 | 1.70521114010536776428855145343450816 | |||
| 0.60459 97880 78072 61686 [Mw 32] | Relationship among the area of an equilateral triangle and the inscribed circle. | 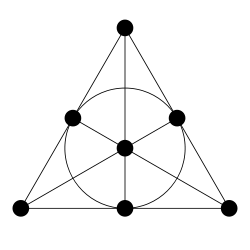 |
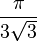 |
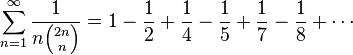 |
Sum[1/(n Binomial[2 n, n]) , {n, 1, ∞}] |
T | [0;1,1,1,1,8,10,2,2,3,3,1,9,2,5,4,1,27,27,6,6,...] | 0.60459978807807261686469275254738524 | ||
| 1.15470 05383 79251 52901 [Mw 33] | Hermite Constant [32] |  |
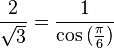 |
2/sqrt(3) | A | 1+ |
[1;6,2,6,2,6,2,6,2,6,2,6,2,6,2,6,2,6,2,6,2,6,2,...] [1;6,2] |
1.15470053837925152901829756100391491 | ||
| 0.41245 40336 40107 59778 [Mw 34] | Prouhet–Thue–Morse constant [33] |  |
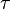 |
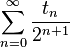 where where  is the Thue–Morse sequence and is the Thue–Morse sequence and Where 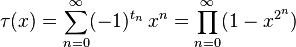 |
T | [0;2,2,2,1,4,3,5,2,1,4,2,1,5,44,1,4,1,2,4,1,1,...] | 0.41245403364010759778336136825845528 | |||
| 0.58057 75582 04892 40229 [Mw 35] | Pell Constant [34] |  |
 |
N[1-prod[n=0 to ∞] {1-1/(2^(2n+1)}] |
T ? | [0;1,1,2,1,1,1,1,14,1,3,1,1,6,9,18,7,1,27,1,1,...] | 0.58057755820489240229004389229702574 | |||
| 0.66274 34193 49181 58097 [Mw 36] | Laplace limit [35] |  |
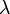 |
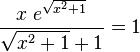 |
(x e^sqrt(x^2+1)) /(sqrt(x^2+1)+1) = 1 |
[0;1,1,1,27,1,1,1,8,2,154,2,4,1,5,1,1,2,1601,...] | 1782 ~ | 0.66274341934918158097474209710925290 | ||
| 0.17150 04931 41536 06586 [Mw 37] | Hall-Montgomery Constant [36] |  |
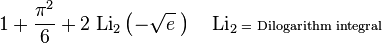 |
1 + Pi^2/6 + 2*PolyLog[2, -Sqrt[E]] | [0;5,1,4,1,10,1,1,11,18,1,2,19,14,1,51,1,2,1,...] | 0.17150049314153606586043997155521210 | ||||
| 1.55138 75245 48320 39226 [Mw 38] | Calabi triangle constant [37] | 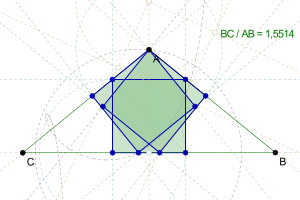 |
 |
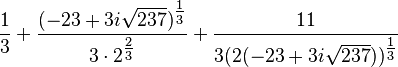 |
FindRoot[ 2x^3-2x^2-3x+2 ==0, {x, 1.5}, WorkingPrecision->40] |
A | [1;1,1,4,2,1,2,1,5,2,1,3,1,1,390,1,1,2,11,6,2,...] | 1946 ~ | 1.55138752454832039226195251026462381 | |
| 1.22541 67024 65177 64512 [Mw 39] | Gamma(3/4) [38] | 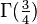 |
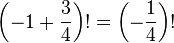 |
(-1+3/4)! | [1;4,2,3,2,2,1,1,1,2,1,4,7,1,171,3,2,3,1,1,8,3,...] | 1.22541670246517764512909830336289053 | ||||
| 1.20205 69031 59594 28539 [Mw 40] | Apéry's constant [39] | 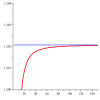 |
 |
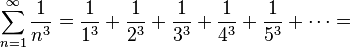
|
Sum[n=1 to ∞] {1/n^3} |
I | [1;4,1,18,1,1,1,4,1,9,9,2,1,1,1,2,7,1,1,7,11,...] | 1979 | 1.20205690315959428539973816151144999 | |
| 0.91596 55941 77219 01505 [Mw 41] | Catalan's constant[40][41][42] | 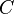 |
 |
Sum[n=0 to ∞] {(-1)^n/(2n+1)^2} |
T | [0;1,10,1,8,1,88,4,1,1,7,22,1,2,3,26,1,11,...] | 1864 | 0.91596559417721901505460351493238411 | ||
| 0.78539 81633 97448 30961 [Mw 42] | Beta(1) [43] |  |
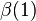 |
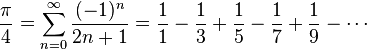 |
Sum[n=0 to ∞] {(-1)^n/(2n+1)} |
T | [0; 1,3,1,1,1,15,2,72,1,9,1,17,1,2,1,5,1,1,10,...] | 1805 to 1859 |
0.78539816339744830961566084581987572 | |
| 0.00131 76411 54853 17810 [Mw 43] | Heath-Brown–Moroz constant[44] | 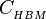 |
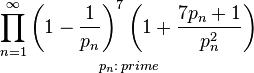 |
N[prod[n=1 to ∞] {((1-1/prime(n))^7) *(1+(7*prime(n)+1) /(prime(n)^2))}] |
T ? | [0;758,1,13,1,2,3,56,8,1,1,1,1,1,143,1,1,1,2,...] | 0.00131764115485317810981735232251358 | |||
| 0.56755 51633 06957 82538 | Module of Infinite Tetration of i |
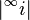 |
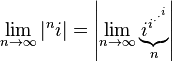 |
Mod(i^i^i^...) | [0;1,1,3,4,1,58,12,1,51,1,4,12,1,1,2,2,3,...] | 0.56755516330695782538461314419245334 | ||||
| 0.78343 05107 12134 40705 [Mw 44] | Sophomore's dream 1 J.Bernoulli [45] |  |
 |
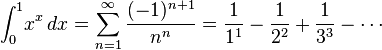 |
Sum[n=1 to ∞] {-(-1)^n /n^n} |
[0;1,3,1,1,1,1,1,1,2,4,7,2,1,2,1,1,1,2,1,14,...] | 1697 | 0.78343051071213440705926438652697546 | ||
| 1.29128 59970 62663 54040 [Mw 45] | Sophomore's dream 2 J.Bernoulli [46] |  |
 |
 |
Sum[n=1 to ∞] {1/(n^n)} |
[1;3,2,3,4,3,1,2,1,1,6,7,2,5,3,1,2,1,8,1,2,4,...] | 1697 | 1.29128599706266354040728259059560054 | ||
| 0.70523 01717 91800 96514 [Mw 46] | Primorial constant Sum of the product of inverse of primes [47] |
 |
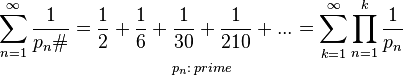 |
Sum[k=1 to ∞] (prod[n=1 to k] {1/prime(n)}) |
I | [0;1,2,2,1,1,4,1,2,1,1,6,13,1,4,1,16,6,1,1,4,...] | 0.70523017179180096514743168288824851 | |||
| 0.14758 36176 50433 27417 [Mw 47] | Plouffe's gamma constant [48] | 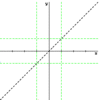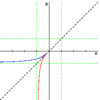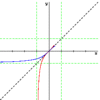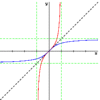 |
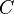 |
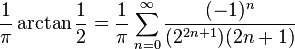 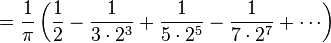 |
Arctan(1/2)/pi | T | [0;6,1,3,2,5,1,6,5,3,1,1,2,1,1,2,3,1,2,3,2,2,...] | 0.14758361765043327417540107622474052 | ||
| 0.15915 49430 91895 33576 [Mw 48] | Plouffe's A constant [49] | 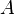 |
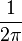 |
1/(2 pi) | T | [0;6,3,1,1,7,2,146,3,6,1,1,2,7,5,5,1,4,1,2,42,...] | 0.15915494309189533576888376337251436 | |||
| 0.29156 09040 30818 78013 [Mw 49] | Dimer constant 2D, Domino tiling[50][51] |
 |

C=Catalan |
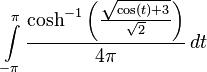 |
N[int[-pi to pi] {arccosh(sqrt( cos(t)+3)/sqrt(2)) /(4*Pi)dt}] |
[0;3,2,3,16,8,10,3,1,1,2,1,3,1,2,13,1,1,4,1,5,...] | 0.29156090403081878013838445646839491 | |||
| 0.49801 56681 18356 04271 0.15494 98283 01810 68512 i |
Factorial(i)[52] | 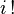 |
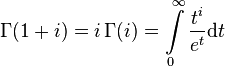 |
Integral_0^∞ t^i/e^t dt |
C | [0;6,2,4,1,8,1,46,2,2,3,5,1,10,7,5,1,7,2,...] - [0;2,125,2,18,1,2,1,1,19,1,1,1,2,3,34,...] i |
0.49801566811835604271369111746219809 - 0.15494982830181068512495513048388 i | |||
| 2.09455 14815 42326 59148 [Mw 50] | Wallis Constant |  |
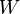 |
![\sqrt[3]{\frac{45-\sqrt{1929}}{18}}+\sqrt[3]{\frac{45+\sqrt{1929}}{18}}](../I/m/92ea83d0662ef5f44e7999cefa82d592.png) |
(((45-sqrt(1929)) /18))^(1/3)+ (((45+sqrt(1929)) /18))^(1/3) |
A | [2;10,1,1,2,1,3,1,1,12,3,5,1,1,2,1,6,1,11,4,...] | 1616 to 1703 |
2.09455148154232659148238654057930296 | |
| 0.72364 84022 98200 00940 [Mw 51] | Sarnak constant | 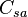 |
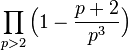 |
N[prod[k=2 to ∞] {1-(prime(k)+2) /(prime(k)^3)}] |
T ? | [0;1,2,1,1,1,1,1,1,1,4,4,1,1,1,1,1,1,1,8,2,1,1,...] | 0.72364840229820000940884914980912759 | |||
| 0.63212 05588 28557 67840 [Mw 52] | Time constant [53] |  |
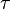 |
|
lim_(n->∞) (1- !n/n!) !n=subfactorial |
T | [0;1,1,1,2,1,1,4,1,1,6,1,1,8,1,1,10,1,1,12,1,...] = [0;1,1,1,2n], n∈ℕ |
0.63212055882855767840447622983853913 | ||
| 1.04633 50667 70503 18098 | Minkowski-Siegel mass constant [54] |  |
![\prod_{n=1}^{\infty} \frac{n!}{\sqrt{2\pi n}\left(\frac{n}{e}\right)^n \sqrt[12]{1+\tfrac1{n}}}](../I/m/c4428bd44a136806f8bba3e3ba545eea.png) |
N[prod[n=1 to ∞] n! /(sqrt(2*Pi*n) *(n/e)^n *(1+1/n) ^(1/12))] |
[1;21,1,1,2,1,1,4,2,1,5,7,2,1,20,1,1,1134,3,..] | 1867 1885 1935 |
1.04633506677050318098095065697776037 | |||
| 5.24411 51085 84239 62092 [Mw 53] | Lemniscate Constant [55] | 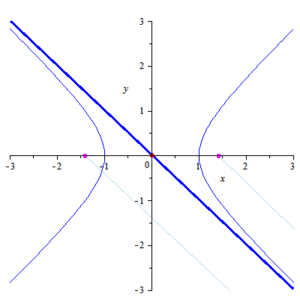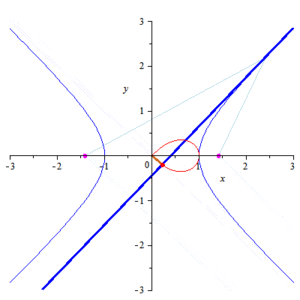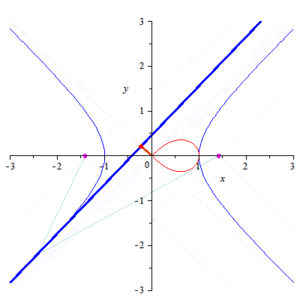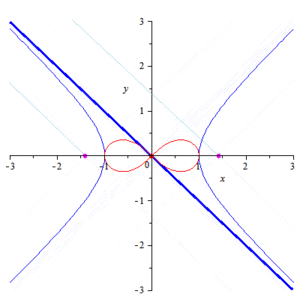 |
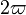 |
![\frac{[\Gamma(\tfrac14)]^2}{\sqrt{2 \pi}} =
4\int^1_0 \frac{dx}{\sqrt{(1-x^2)(2-x^2)}}](../I/m/2695f128dbb22db23d9e66f062715daa.png) |
Gamma[ 1/4 ]^2 /Sqrt[ 2 Pi ] |
[5;4,10,2,1,2,3,29,4,1,2,1,2,1,2,1,4,9,1,4,1,2,...] | 1718 | 5.24411510858423962092967917978223883 | ||
| 0.66170 71822 67176 23515 [Mw 54] | Robbins constant [56] | 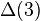 |
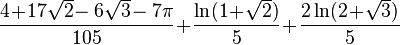 |
(4+17*2^(1/2)-6 *3^(1/2)+21*ln(1+ 2^(1/2))+42*ln(2+ 3^(1/2))-7*Pi)/105 |
[0;1,1,1,21,1,2,1,4,10,1,2,2,1,3,11,1,331,1,4,...] | 1978 | 0.66170718226717623515583113324841358 | |||
| 1.30357 72690 34296 39125 [Mw 55] | Conway constant [57] |  |
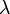 |
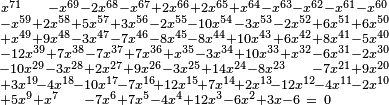 |
A | [1;3,3,2,2,54,5,2,1,16,1,30,1,1,1,2,2,1,14,1,...] | 1987 | 1.30357726903429639125709911215255189 | ||
| 1.18656 91104 15625 45282 [Mw 56] | Khinchin–Lévy constant[58] |  |
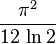 |
pi^2 /(12 ln 2) | [1;5,2,1,3,1,1,28,18,16,3,2,6,2,6,1,1,5,5,9,...] | 1935 | 1.18656911041562545282172297594723712 | |||
| 0.83564 88482 64721 05333 | Baker constant [59] |  |
 |
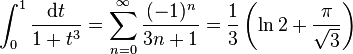 |
Sum[n=0 to ∞] {((-1)^(n))/(3n+1)} |
[0;1,5,11,1,4,1,6,1,4,1,1,1,2,1,3,2,2,2,2,1,3,...] | 0.83564884826472105333710345970011076 | |||
| 23.10344 79094 20541 6160 [Mw 57] | Kempner Serie(0) [60] | 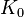 |
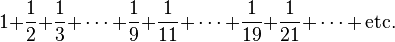
|
1+1/2+1/3+1/4+1/5 +1/6+1/7+1/8+1/9 +1/11+1/12+1/13 +1/14+1/15+... |
[23;9,1,2,3244,1,1,5,1,2,2,8,3,1,1,6,1,84,1,...] | 23.1034479094205416160340540433255981 | ||||
| 0.98943 12738 31146 95174 [Mw 58] | Lebesgue constant [61] |  |
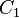 |
 |
4/pi^2*[(2 Sum[k=1 to ∞] {ln(k)/(4*k^2-1)}) -poligamma(1/2)] |
[0;1,93,1,1,1,1,1,1,1,7,1,12,2,15,1,2,7,2,1,5,...] | ? | 0.98943127383114695174164880901886671 | ||
| 0.19452 80494 65325 11361 [Mw 59] | 2nd du Bois-Reymond constant [62] | 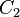 |
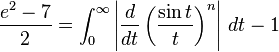 |
(e^2-7)/2 | T | [0;5,7,9,11,13,15,17,19,21,23,25,27,29,31,...] = [0;2p+3], p∈ℕ |
0.19452804946532511361521373028750390 | |||
| 0.78853 05659 11508 96106 [Mw 60] | Lüroth constant[63] | 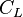 |
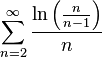 |
Sum[n=2 to ∞] log(n/(n-1))/n |
[0;1,3,1,2,1,2,4,1,127,1,2,2,1,3,8,1,1,2,1,16,...] | 0.78853056591150896106027632216944432 | ||||
| 1.18745 23511 26501 05459 [Mw 61] | Foias constant α [64] | 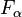 |
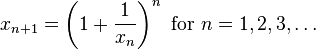
Foias constant is the unique real number such that if x1 = α then the sequence diverges to ∞. When x1 = α, |
[1;5,2,1,81,3,2,2,1,1,1,1,1,6,1,1,3,1,1,4,3,2,...] | 2000 | 1.18745235112650105459548015839651935 | ||||
| 2.29316 62874 11861 03150 [Mw 62] | Foias constant β | 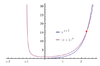 |
 |
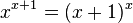 |
x^(x+1) = (x+1)^x |
[2;3,2,2,3,4,2,3,2,130,1,1,1,1,1,6,3,2,1,15,1,...] | 2000 | 2.29316628741186103150802829125080586 | ||
| 0.82246 70334 24113 21823 [Mw 63] | Nielsen-Ramanujan constant [65] | 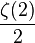 |
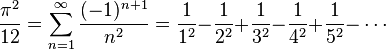 |
Sum[n=1 to ∞] {((-1)^(n+1))/n^2} |
T | [0;1,4,1,1,1,2,1,1,1,1,3,2,2,4,1,1,1,1,1,1,4...] | 1909 | 0.82246703342411321823620758332301259 | ||
| 0.69314 71805 59945 30941 [Mw 64] | Natural logarithm of 2 [66] |  |
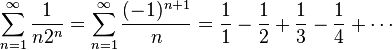 |
Sum[n=1 to ∞] {(-1)^(n+1)/n} |
T | [0;1,2,3,1,6,3,1,1,2,1,1,1,1,3,10,1,1,1,2,1,1,...] | 1550 to 1617 |
0.69314718055994530941723212145817657 | ||
| 0.47494 93799 87920 65033 [Mw 65] | Weierstrass constant [67] | 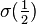 |
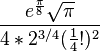 |
(E^(Pi/8) Sqrt[Pi]) /(4 2^(3/4) (1/4)!^2) |
[0;2,9,2,11,1,6,1,4,6,3,19,9,217,1,2,4,8,6...] | 1872 ? | 0.47494937998792065033250463632798297 | |||
| 0.57721 56649 01532 86060 [Mw 66] | Euler-Mascheroni constant |  |
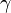 |
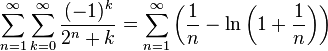
|
sum[n=1 to ∞] |sum[k=0 to ∞] {((-1)^k)/(2^n+k)} |
[0;1,1,2,1,2,1,4,3,13,5,1,1,8,1,2,4,1,1,40,1,...] | 1735 | 0.57721566490153286060651209008240243 | ||
| 1.38135 64445 18497 79337 | Beta, Kneser-Mahler polynomial constant[68] | 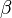 |
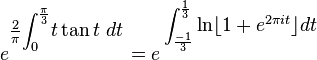 |
e^((PolyGamma(1,4/3) - PolyGamma(1,2/3) +9)/(4*sqrt(3)*Pi)) |
[1;2,1,1,1,1,1,4,1,139,2,1,3,5,16,2,1,1,7,2,1,...] | 1963 | 1.38135644451849779337146695685062412 | |||
| 1.35845 62741 82988 43520 [Mw 67] | Golden Spiral |  |
 |
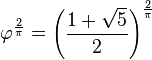 |
GoldenRatio^(2/pi) | [1;2,1,3,1,3,10,8,1,1,8,1,15,6,1,3,1,1,2,3,1,1,...] | 1.35845627418298843520618060050187945 | |||
| 0.57595 99688 92945 43964 [Mw 68] | Stephens constant [69] |  |
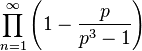 |
Prod[n=1 to ∞] {1-hprime(n) /(hprime(n)^3-1)} |
T ? | [0;1,1,2,1,3,1,3,1,2,1,77,2,1,1,10,2,1,1,1,7,...] | ? | 0.57595996889294543964316337549249669 | ||
| 0.73908 51332 15160 64165 [Mw 69] | Dottie number [70] |  |
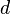 |
![\lim_{x\to \infty} \cos^{[x]}(c) = \lim_{x\to \infty} \underbrace{\cos(\cos(\cos(\cdots(\cos(c)))))}_x](../I/m/40de6e234f0f9a14f753e2389885fb10.png) |
cos(c)=c | T | [0;1,2,1,4,1,40,1,9,4,2,1,15,2,12,1,21,1,17,...] | ? | 0.73908513321516064165531208767387340 | |
| 0.67823 44919 17391 97803 [Mw 70] | Taniguchi constant [71] |  |

 |
Prod[n=1 to ∞] {1 -3/ithprime(n)^3 +2/ithprime(n)^4 +1/ithprime(n)^5 -1/ithprime(n)^6} |
T ? | [0;1,2,9,3,1,2,9,11,1,13,2,15,1,1,1,2,4,1,1,1,...] | ? | 0.67823449191739197803553827948289481 | ||
| 1.85407 46773 01371 91843 [Mw 71] | Gauss' Lemniscate constant[72] | 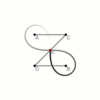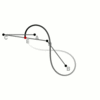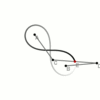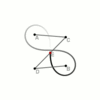 |
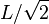 |
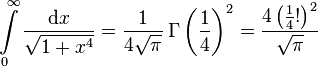
 |
pi^(3/2)/(2 Gamma(3/4)^2) | [1;1,5,1,5,1,3,1,6,2,1,4,16,3,112,2,1,1,18,1,...] | 1.85407467730137191843385034719526005 | |||
| 1.75874 36279 51184 82469 | Infinite product constant, with Alladi-Grinstead [73] |  |
 |
Prod[n=2 to inf] {(1+1/n)^(1/n)} | [1;1,3,6,1,8,1,4,3,1,4,1,1,1,6,5,2,40,1,387,2,...] | 1977 | 1.75874362795118482469989684865589317 | |||
| 1.86002 50792 21190 30718 | Spiral of Theodorus [74] |  |
 |
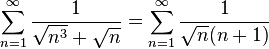 |
Sum[n=1 to ∞] {1/(n^(3/2) +n^(1/2))} |
[1;1,6,6,1,15,11,5,1,1,1,1,5,3,3,3,2,1,1,2,19,...] | -460 to -399 |
1.86002507922119030718069591571714332 | ||
| 2.79128 78474 77920 00329 | Nested radical S5 |  |
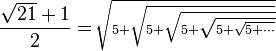
|
(sqrt(21)+1)/2 | A | A222134 | [2;1,3,1,3,1,3,1,3,1,3,1,3,1,3,1,3,1,3,1,3,1,3,...] [2;1,3] |
? | 2.79128784747792000329402359686400424 | |
| 0.70710 67811 86547 52440 +0.70710 67811 86547 524 i [Mw 72] |
Square root of i [75] | 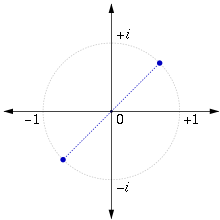 |
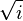 |
![\sqrt[4]{-1} = \frac{1+i}{\sqrt{2}} = e^ \frac{i\pi}{4} =
\cos\left (\frac{\pi}{4} \right ) + i\sin\left ( \frac{\pi}{4} \right )](../I/m/4f23f753477adc58b0602352fdb3f711.png) |
(1+i)/(sqrt 2) | C A | [0;1,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,..] = [0;1,2,...] [0;1,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,..] i = [0;1,2,...] i |
? | 0.70710678118654752440084436210484903 + 0.70710678118654752440084436210484 i | |
| 0.80939 40205 40639 13071 [Mw 73] | Alladi–Grinstead constant [76] | 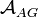 |
 |
e^{(sum[k=2 to ∞] |sum[n=1 to ∞] {1/(n k^(n+1))})-1} |
[0;1,4,4,17,4,3,2,5,3,1,1,1,1,6,1,1,2,1,22,...] | 1977 | 0.80939402054063913071793188059409131 | |||
| 2.58498 17595 79253 21706 [Mw 74] | Sierpiński's constant [77] |  |
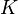 |
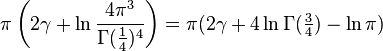
|
-Pi Log[Pi]+2 Pi EulerGamma +4 Pi Log [Gamma[3/4]] |
[2;1,1,2,2,3,1,3,1,9,2,8,4,1,13,3,1,15,18,1,...] | 1907 | 2.58498175957925321706589358738317116 | ||
| 1.73245 47146 00633 47358 [Ow 2] | Reciprocal of the Euler–Mascheroni constant |  |
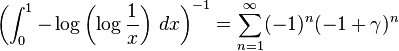 |
1/Integrate_ {x=0 to 1} -log(log(1/x)) |
[1;1,2,1,2,1,4,3,13,5,1,1,8,1,2,4,1,1,40,1,11,...] | 1.73245471460063347358302531586082968 | ||||
| 1.43599 11241 76917 43235 [Mw 75] | Lebesgue constant (interpolation) [78][79] |  |
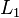 |
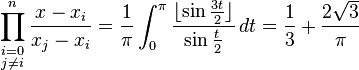 |
1/3 + 2*sqrt(3)/pi | T | [1;2,3,2,2,6,1,1,1,1,4,1,7,1,1,1,2,1,3,1,2,1,1,...] | 1902 ~ | 1.43599112417691743235598632995927221 | |
| 3.24697 96037 17467 06105 [Mw 76] | Silver root Tutte–Beraha constant [80] |
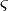 |
![2+2 \cos \frac {2\pi} 7 = \textstyle 2+\frac{2+\sqrt[3]{7 + 7 \sqrt[3]{7 + 7 \sqrt[3]{\, 7 + \cdots}}}}{1+\sqrt[3]{7 + 7 \sqrt[3]{7 + 7 \sqrt[3]{\, 7 + \cdots}}}}](../I/m/1b2319784420d794cbbcd98b891c0eb0.png) |
2+2 cos(2Pi/7) | A | [3;4,20,2,3,1,6,10,5,2,2,1,2,2,1,18,1,1,3,2,...] | 3.24697960371746706105000976800847962 | |||
| 1.94359 64368 20759 20505 [Mw 77] | Euler Totient constant [81][82] |
 |
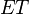 |
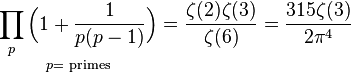 |
zeta(2)*zeta(3) /zeta(6) |
[1;1,16,1,2,1,2,3,1,1,3,2,1,8,1,1,1,1,1,1,1,32,...] | 1750 | 1.94359643682075920505707036257476343 | ||
| 1.49534 87812 21220 54191 | Fourth root of five [83] | ![\sqrt[4]{5}](../I/m/d3588cd9eca9bb8f14872676cfccb02d.png) |
![\sqrt[5]{5 \,\sqrt[5]{5 \, \sqrt[5]{5 \,\sqrt[5]{5 \,\sqrt[5]{5 \,\cdots}}}}}](../I/m/4c5e3b22d120db6aa2b6c4bf08868ab7.png) |
(5(5(5(5(5(5(5) ^1/5)^1/5)^1/5) ^1/5)^1/5)^1/5) ^1/5 ... |
A | [1;2,53,4,96,2,1,6,2,2,2,6,1,4,1,49,17,2,3,2,...] | 1.49534878122122054191189899414091339 | |||
| 0.87228 40410 65627 97617 [Mw 78] | Area of Ford circle [84] |  |
 |
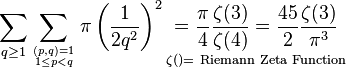 |
pi Zeta(3) /(4 Zeta(4)) | [0;1,6,1,4,1,7,5,36,3,29,1,1,10,3,2,8,1,1,1,3,...] | 0.87228404106562797617519753217122587 | |||
| 1.08232 32337 11138 19151 [Mw 79] | Zeta(4) [85] | 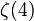 |
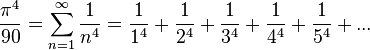 |
Sum[n=1 to ∞] {1/n^4} |
T | [1;12,6,1,3,1,4,183,1,1,2,1,3,1,1,5,4,2,7,23,...] | ? | 1.08232323371113819151600369654116790 | ||
| 1.56155 28128 08830 27491 | Triangular root of 2.[86] |  |
 |
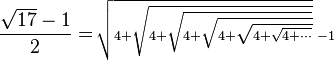
|
(sqrt(17)-1)/2 | A | [1;1,1,3,1,1,3,1,1,3,1,1,3,1,1,3,1,1,3,1,1,3,1,...] [1;1,1,3] |
1.56155281280883027491070492798703851 | ||
| 9.86960 44010 89358 61883 | Pi Squared |  |
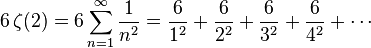 |
6 Sum[n=1 to ∞] {1/n^2} |
T | A002388 | [9;1,6,1,2,47,1,8,1,1,2,2,1,1,8,3,1,10,5,1,3,...] | 9.86960440108935861883449099987615114 | ||
| 1.32471 79572 44746 02596 [Mw 80] | Plastic number [87] |  |
 |
![\sqrt[3]{1 + \! \sqrt[3]{1 + \! \sqrt[3]{1 + \cdots}}} = \textstyle \sqrt[3]{\frac{1}{2}+ \! \sqrt{\frac{23}{108}}}+ \! \sqrt[3]{\frac{1}{2}- \! \sqrt{\frac{23}{108}}}](../I/m/673d57a53db2fff9853383d23046ab3b.png) |
(1+(1+(1+(1+(1+(1 )^(1/3))^(1/3))^(1/3)) ^(1/3))^(1/3))^(1/3) |
A | [1;3,12,1,1,3,2,3,2,4,2,141,80,2,5,1,2,8,2,...] | 1929 | 1.32471795724474602596090885447809734 | |
| 2.37313 82208 31250 90564 | Lévy 2 constant [88] |  |
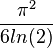 |
Pi^(2)/(6*ln(2)) | T | [2;2,1,2,8,57,9,32,1,1,2,1,2,1,2,1,2,1,3,2,...] | 1936 | 2.37313822083125090564344595189447424 | ||
| 0.85073 61882 01867 26036 [Mw 81] | Regular paperfolding sequence [89][90] |  |
 |
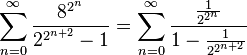 |
N[Sum[n=0 to ∞] {8^2^n/(2^2^ (n+2)-1)},37] |
[0;1,5,1,2,3,21,1,4,107,7,5,2,1,2,1,1,2,1,6,...] | 0.85073618820186726036779776053206660 | |||
| 1.15636 26843 32269 71685 [Mw 82] | Cubic recurrence constant [91][92] |  |
![\prod_{n=1}^\infty n^{{3}^{-n}} = \sqrt[3] {1 \sqrt[3] {2 \sqrt[3]{3 \cdots}}} = 1^{1/3} \; 2^{1/9} \; 3^{1/27} \cdots](../I/m/757f408815d2f1869ab4e1cb636470ea.png) |
prod[n=1 to ∞] {n ^(1/3)^n} |
[1;6,2,1,1,8,13,1,3,2,2,6,2,1,2,1,1,1,10,33,...] | 1.15636268433226971685337032288736935 | ||||
| 1.26185 95071 42914 87419 [Mw 83] | Fractal dimension of the Koch snowflake [93] |  |
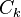 |
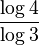 |
log(4)/log(3) | T | A100831 | [1;3,1,4,1,1,11,1,46,1,5,112,1,1,1,1,1,3,1,7,...] | 1.26185950714291487419905422868552171 | |
| 6.58088 59910 17920 97085 | Froda constant[94] | 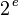 |
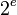 |
2^e | [6;1,1,2,1,1,2,3,1,14,11,4,3,1,1,7,5,5,2,7,...] | 6.58088599101792097085154240388648649 | ||||
| 0.26149 72128 47642 78375 [Mw 84] | Meissel-Mertens constant [95] | 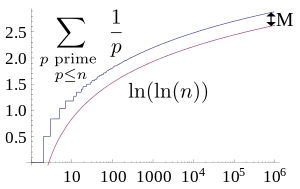 |
 |
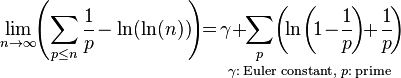 |
gamma+ Sum[n=1 to ∞] {ln(1-1/prime(n)) +1/prime(n)} |
T ? | [0;3,1,4,1,2,5,2,1,1,1,1,13,4,2,4,2,1,33,296,...] | 1866 & 1873 |
0.26149721284764278375542683860869585 | |
| 4.81047 73809 65351 65547 | John constant [96] |  |
![\sqrt[i]{i} = i^{-i} = (i^i)^{-1} = (((i)^i)^i)^i = e^{\frac{\pi}{2}} = \sqrt{\sum_{n=0}^\infty \frac{\pi^{n}}{n!}}](../I/m/2d9211c1db7a7e3e8cf1f5c966abc5c0.png) |
e^(π/2) | T | [4;1,4,3,1,1,1,1,1,1,1,1,7,1,20,1,3,6,10,3,2,...] | 4.81047738096535165547303566670383313 | |||
| -0.5 ± 0.86602 54037 84438 64676 i |
Cube Root of 1 [97] | 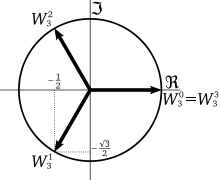 |
![\sqrt[3]{1}](../I/m/a1d45f6358f35869226f97927d7d465a.png) |
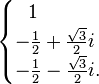 |
1, E^(2i pi/3), E^(-2i pi/3) |
C A | - [0,5] ± [0;1,6,2,6,2,6,2,6,2,6,2,6,2,6,2,6,2,6,2,...] i - [0,5] ± [0; 1, 6, 2] i |
- 0.5 ± 0.8660254037844386467637231707529 i | ||
| 0.11000 10000 00000 00000 0001 [Mw 85] | Liouville number [98] | 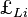 |
 |
Sum[n=1 to ∞] {10^(-n!)} |
T | [1;9,1,999,10,9999999999999,1,9,999,1,9] | 0.11000100000000000000000100... | |||
| 0.06598 80358 45312 53707 [Mw 86] | Lower limit of Tetration [99] | 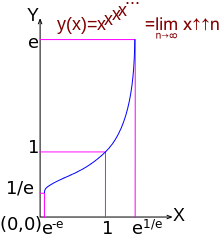 |
 |
 |
1/(e^e) | [0;15,6,2,13,1,3,6,2,1,1,5,1,1,1,9,4,1,1,1,...] | 0.06598803584531253707679018759684642 | |||
| 1.83928 67552 14161 13255 | Tribonacci constant[100] |  |
![\textstyle \frac{1+\sqrt[3]{19+3\sqrt{33}}+\sqrt[3]{19-3\sqrt{33}}}{3} = \scriptstyle \, 1+ \left(\sqrt[3]{\tfrac12 + \sqrt[3]{\tfrac12 + \sqrt[3]{\tfrac12 + ...}}}\right)^{-1}](../I/m/0707b429626a5e063af3349ccb392ec6.png) |
(1/3)*(1+(19+3 *sqrt(33))^(1/3) +(19-3 *sqrt(33))^(1/3)) |
A | [1;1,5,4,2,305,1,8,2,1,4,6,14,3,1,13,5,1,7,...] | 1.83928675521416113255185256465328660 | |||
| 0.36651 29205 81664 32701 | Median of the Gumbel distribution [101] |  |
 |
 |
-ln(ln(2)) | A074785 | [0;2,1,2,1,2,6,1,6,6,2,2,2,1,12,1,8,1,1,3,1,...] | 0.36651292058166432701243915823266947 | ||
| 36.46215 96072 07911 7709 | Pi^pi [102] | 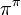 |
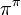 |
pi^pi | [36;2,6,9,2,1,2,5,1,1,6,2,1,291,1,38,50,1,2,...] | 36.4621596072079117709908260226921236 | ||||
| 0.53964 54911 90413 18711 | Ioachimescu constant [103] |  |
 |
γ +N[ sum[n=1 to ∞] {((-1)^(2n) gamma_n) /(2^n n!)}] |
2- |
[0;1,1,5,1,4,6,1,1,2,6,1,1,2,1,1,1,37,3,2,1,...] | 0.53964549119041318711050084748470198 | |||
| 15.15426 22414 79264 1897 [Mw 87] | Exponential reiterated constant [104] |  |
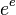 |
 |
Sum[n=0 to ∞] {(e^n)/n!} |
[15;6,2,13,1,3,6,2,1,1,5,1,1,1,9,4,1,1,1,6,7,...] | 15.1542622414792641897604302726299119 | |||
| 0.64624 54398 94813 30426 [Mw 88] | Masser–Gramain constant [105] | 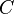 |
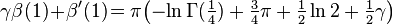
|
Pi/4*(2*Gamma + 2*Log[2] + 3*Log[Pi]- 4 Log[Gamma[1/4]]) |
[0;1,1,1,4,1,3,2,3,9,1,33,1,4,3,3,5,3,1,3,4,...] | 0.64624543989481330426647339684579279 | ||||
| 1.11072 07345 39591 56175 [Mw 89] | The ratio of a square and circle circumscribed [106] |  |
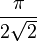 |
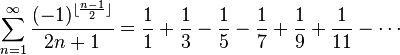 |
sum[n=1 to ∞] {(-1)^(floor( (n-1)/2)) /(2n-1)} |
T | [1;9,31,1,1,17,2,3,3,2,3,1,1,2,2,1,4,9,1,3,...] | 1.11072073453959156175397024751517342 | ||
| 1.45607 49485 82689 67139 [Mw 90] | Backhouse's constant [107] | 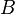 |
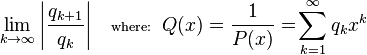
|
1/( FindRoot[0 == 1 + Sum[x^n Prime[n], {n, 10000}], {x, {1}}) | [1;2,5,5,4,1,1,18,1,1,1,1,1,2,13,3,1,2,4,16,...] | 1995 | 1.45607494858268967139959535111654355 | |||
| 1.85193 70519 82466 17036 [Mw 91] | Gibbs constant [108] |  |
 Sin integral |
|
SinIntegral[Pi] | [1;1,5,1,3,15,1,5,3,2,7,2,1,62,1,3,110,1,39,...] | 1.85193705198246617036105337015799136 | |||
| 0.23571 11317 19232 93137 [Mw 92] | Copeland–Erdős constant [109] | 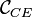 |
 |
sum[n=1 to ∞] {prime(n) /(n+(10^ sum[k=1 to n]{floor (log_10 prime(k))}))} |
I | [0;4,4,8,16,18,5,1,1,1,1,7,1,1,6,2,9,58,1,3,...] | 0.23571113171923293137414347535961677 | |||
| 1.52362 70862 02492 10627 [Mw 93] | Fractal dimension of the boundary of the dragon curve [110] | 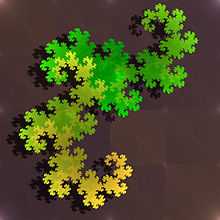 |
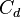 |
![\frac{\log\left(\frac{1+\sqrt[3]{73-6\sqrt{87}}+\sqrt[3]{73+6\sqrt{87}}}{3}\right)}
{\log(2)}](../I/m/a808f46362f022c14afa2d9a89aeebcc.png) |
(log((1+(73-6 sqrt(87))^1/3+ (73+6 sqrt(87))^1/3) /3))/ log(2))) |
T | [1;1,1,10,12,2,1,149,1,1,1,3,11,1,3,17,4,1,...] | 1.52362708620249210627768393595421662 | ||
| 1.78221 39781 91369 11177 [Mw 94] | Grothendieck constant [111] | 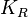 |
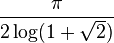 |
pi/(2 log(1+sqrt(2))) | [1;1,3,1,1,2,4,2,1,1,17,1,12,4,3,5,10,1,1,3,...] | 1.78221397819136911177441345297254934 | ||||
| 1.58496 25007 21156 18145 [Mw 95] | Hausdorff dimension, Sierpinski triangle [112] |  |
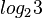 |
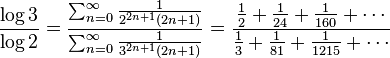 |
( Sum[n=0 to ∞] {1/ (2^(2n+1) (2n+1))})/ (Sum[n=0 to ∞] {1/ (3^(2n+1) (2n+1))}) |
T | [1;1,1,2,2,3,1,5,2,23,2,2,1,1,55,1,4,3,1,1,...] | 1.58496250072115618145373894394781651 | ||
| 1.30637 78838 63080 69046 [Mw 96] | Mills' constant [113] | 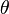 |
 primes primes |
Nest[ NextPrime[#^3] &, 2, 7]^(1/3^8) | [1;3,3,1,3,1,2,1,2,1,4,2,35,21,1,4,4,1,1,3,2,...] | 1947 | 1.30637788386308069046861449260260571 | |||
| 2.02988 32128 19307 25004 [Mw 97] | Figure eight knot hyperbolic volume [114] |  |
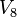 |
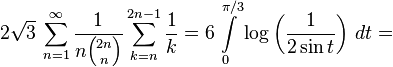
|
6 integral[0 to pi/3] {log(1/(2 sin (n)))} |
[2;33,2,6,2,1,2,2,5,1,1,7,1,1,1,113,1,4,5,1,...] | 2.02988321281930725004240510854904057 | |||
| 262 53741 26407 68743 .99999 99999 99250 073 [Mw 98] |
Hermite–Ramanujan constant[115] | 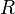 |
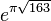 |
e^(π sqrt(163)) | T | [262537412640768743;1,1333462407511,1,8,1,1,5,...] | 1859 | 262537412640768743.999999999999250073 | ||
| 1.74540 56624 07346 86349 [Mw 99] | Khinchin harmonic mean [116] |  |
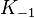 |

a1 ... an are elements of a continued fraction [a0; a1, a2, ..., an] |
(log 2)/ (sum[n=1 to ∞] {1/n log(1+ 1/(n(n+2))} |
[1;1,2,1,12,1,5,1,5,13,2,13,2,1,9,1,6,1,3,1,...] | 1.74540566240734686349459630968366106 | |||
| 1.64872 12707 00128 14684 [Ow 3] | Square root of the number e [117] | 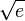 |
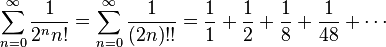 |
Sum[n=0 to ∞] {1/(2^n n!)} |
T | [1;1,1,1,5,1,1,9,1,1,13,1,1,17,1,1,21,1,1,...] = [1;1,1,1,4p+1], p∈ℕ |
1.64872127070012814684865078781416357 | |||
| 1.01734 30619 84449 13971 [Mw 100] | Zeta(6) [118] |  |
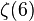 |
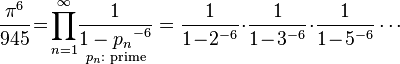 |
Prod[n=1 to ∞] {1/(1-ithprime (n)^-6)} |
T | [1;57,1,1,1,15,1,6,3,61,1,5,3,1,6,1,3,3,6,1,...] | 1.01734306198444913971451792979092052 | ||
| 0.10841 01512 23111 36151 [Mw 101] | Trott constant [119] |  |
![\textstyle [1, 0, 8, 4, 1, 0, 1, 5, 1, 2, 2, 3, 1, 1, 1, 3, 6,...]](../I/m/1609612d4e686145ff3672c2198c1b7d.png)
|
Trott Constant | [0;9,4,2,5,1,2,2,3,1,1,1,3,6,1,5,1,1,2,...] | 0.10841015122311136151129081140641509 | ||||
| 0.00787 49969 97812 3844 [Mw 102] | Chaitin constant [120] | 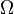 |
See also: Halting problem |
T | [0; 126, 1, 62, 5, 5, 3, 3, 21, 1, 4, 1] | 1975 | 0.0078749969978123844 | |||
| 0.83462 68416 74073 18628 [Mw 103] | Gauss constant [121] |  |
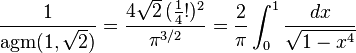
|
(4 sqrt(2)((1/4)!)^2) /pi^(3/2) |
T | [0;1,5,21,3,4,14,1,1,1,1,1,3,1,15,1,3,7,1,...] | 0.83462684167407318628142973279904680 | |||
| 1.45136 92348 83381 05028 [Mw 104] | Ramanujan–Soldner constant[122][123] |  |
 |
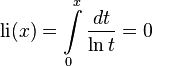 li = Logarithmic integral li = Logarithmic integral
|
FindRoot[li(x) = 0] | I | [1;2,4,1,1,1,3,1,1,1,2,47,2,4,1,12,1,1,2,2,1,...] | 1792 to 1809 |
1.45136923488338105028396848589202744 | |
| 0.64341 05462 88338 02618 [Mw 105] | Cahen's constant [124] | 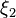 |
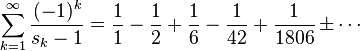
Where sk is the kth term of Sylvester's sequence 2, 3, 7, 43, 1807, ...
|
T | [0; 1, 1, 1, 4, 9, 196, 16641, 639988804, ...] | 1891 | 0.64341054628833802618225430775756476 | |||
| 1.41421 35623 73095 04880 [Mw 106] | Square root of 2, Pythagoras constant.[125] | 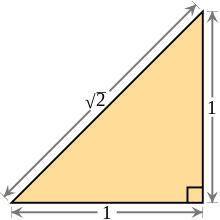 |
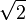 |
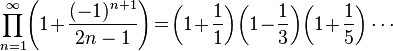 |
prod[n=1 to ∞] {1+(-1)^(n+1) /(2n-1)} |
A | [1;2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,2,...] = [1;2...] |
1.41421356237309504880168872420969808 | ||
| 1.77245 38509 05516 02729 [Mw 107] | Carlson–Levin constant [126] | 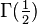 |
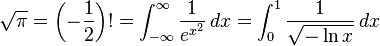 |
sqrt (pi) | T | [1;1,3,2,1,1,6,1,28,13,1,1,2,18,1,1,1,83,1,...] | 1.77245385090551602729816748334114518 | |||
| 1.05946 30943 59295 26456 [Ow 4] | Musical interval between each half tone [127][128] |
|
![\sqrt[12]{2}](../I/m/70b8b8fc763c20423a65bd934e378085.png) |
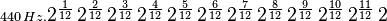
|
2^(1/12) | A | [1;16,1,4,2,7,1,1,2,2,7,4,1,2,1,60,1,3,1,2,...] | 1.05946309435929526456182529494634170 | ||
| 1.01494 16064 09653 62502 [Mw 108] | Gieseking constant [129] |  |

|
sqrt(3)*3/4 *(1 -Sum[n=0 to ∞] {1/((3n+2)^2)} +Sum[n=1 to ∞] {1/((3n+1)^2)}) |
[1;66,1,12,1,2,1,4,2,1,3,3,1,4,1,56,2,2,11,...] | 1912 | 1.01494160640965362502120255427452028 | |||
| 2.62205 75542 92119 81046 [Mw 109] | Lemniscate constant [130] |  |
 |
 |
4 sqrt(2/pi) ((1/4)!)^2 |
T | [2;1,1,1,1,1,4,1,2,5,1,1,1,14,9,2,6,2,9,4,1,...] | 1798 | 2.62205755429211981046483958989111941 | |
| 1.28242 71291 00622 63687 [Mw 110] | Glaisher–Kinkelin constant [131] |  |
 |
e^(1/12-zeta´{-1}) | T ? | [1;3,1,1,5,1,1,1,3,12,4,1,271,1,1,2,7,1,35,...] | 1.28242712910062263687534256886979172 | |||
| -4.22745 35333 76265 408 [Mw 111] | Digamma (1/4) [132] | 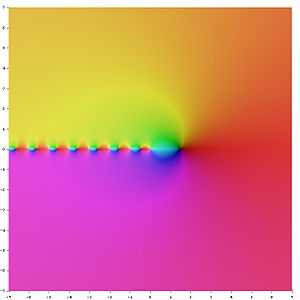 |
 |
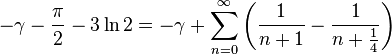 |
-EulerGamma -\pi/2 -3 log 2 |
-[4;4,2,1,1,10,1,5,9,11,1,22,1,1,14,1,2,1,4,...] | -4.2274535333762654080895301460966835 | |||
| 0.28674 74284 34478 73410 [Mw 112] | Strongly Carefree constant [133] | 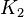 |
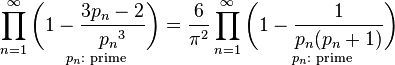 |
N[ prod[k=1 to ∞] {1-(3*prime(k)-2) /(prime(k)^3)}] |
[0;3,2,19,3,12,1,5,1,5,1,5,2,1,1,1,1,1,3,7,...] | 0.28674742843447873410789271278983845 | ||||
| 1.78107 24179 90197 98523 [Mw 113] | Exp.gamma, Barnes G-function [134] |
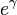 |
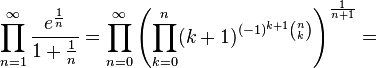
|
Prod[n=1 to ∞] {e^(1/n)} /{1 + 1/n} |
[1;1,3,1,1,3,5,4,1,1,2,2,1,7,9,1,16,1,1,1,2,...] | 1.78107241799019798523650410310717954 | ||||
| 3.62560 99082 21908 31193 [Mw 114] | Gamma(1/4)[135] | 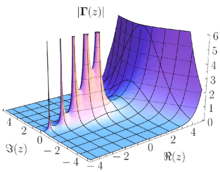 |
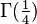 |
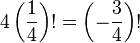 |
4(1/4)! | T | [3;1,1,1,2,25,4,9,1,1,8,4,1,6,1,1,19,1,1,4,1,...] | 1729 | 3.62560990822190831193068515586767200 | |
| 1.66168 79496 33594 12129 [Mw 115] | Somos' quadratic recurrence constant [136] | 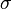 |
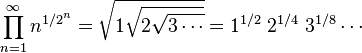 |
prod[n=1 to ∞] {n ^(1/2)^n} |
T ? | [1;1,1,1,21,1,1,1,6,4,2,1,1,2,1,3,1,13,13,...] | 1.66168794963359412129581892274995074 | |||
| 0.95531 66181 245092 78163 | Magic angle [137] | 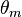 |
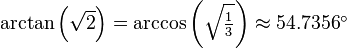 |
arctan(sqrt(2)) | T | [0;1,21,2,1,1,1,2,1,2,2,4,1,2,9,1,2,1,1,1,3,...] | 0.95531661812450927816385710251575775 | |||
| 0.74759 79202 53411 43517 [Mw 116] | Rényi's Parking Constant [138] | 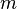 |
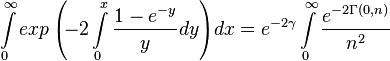 |
[e^(-2*Gamma)] * Int{n,0,∞}[ e^(- 2 *Gamma(0,n)) /n^2] |
[0;1,2,1,25,3,1,2,1,1,12,1,2,1,1,3,1,2,1,43,...] | 0.74759792025341143517873094383017817 | ||||
| 1.44466 78610 09766 13365 [Mw 117] | Steiner number, Iterated exponential Constant [139] |  |
![\sqrt[e]{e}](../I/m/2947b01962d821fccb87efc04ad82256.png) |
 = Upper Limit of Tetration = Upper Limit of Tetration |
e^(1/e) | T | [1;2,4,55,27,1,1,16,9,3,2,8,3,2,1,1,4,1,9,...] | 1.44466786100976613365833910859643022 | ||
| 0.69220 06275 55346 35386 [Mw 118] | Minimum value of función ƒ(x) = xx [140] |
 |
 = Inverse Steiner Number = Inverse Steiner Number |
e^(-1/e) | [0;1,2,4,55,27,1,1,16,9,3,2,8,3,2,1,1,4,1,9,...] | 0.69220062755534635386542199718278976 | ||||
| 0.34053 73295 50999 14282 [Mw 119] | Pólya Random walk constant [141] |  |
 |
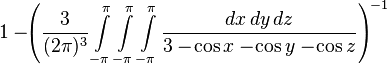
|
1-16*Sqrt[2/3]*Pi^3 /(Gamma[1/24] *Gamma[5/24] *Gamma[7/24] *Gamma[11/24]) |
[0;2,1,14,1,3,8,1,5,2,7,1,12,1,5,59,1,1,1,3,...] | 0.34053732955099914282627318443290289 | |||
| 0.54325 89653 42976 70695 [Mw 120] | Bloch–Landau constant [142] |  |
 |
gamma(1/3) *gamma(5/6) /gamma(1/6) |
[0;1,1,5,3,1,1,2,1,1,6,3,1,8,11,2,1,1,27,4,...] | 1929 | 0.54325896534297670695272829530061323 | |||
| 0.18785 96424 62067 12024 [Mw 121] [Ow 5] | MRB Constant, Marvin Ray Burns [143][144][145] | 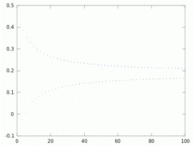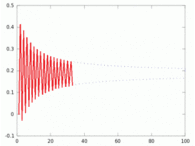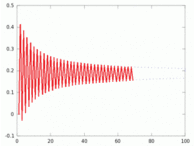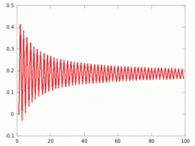 |
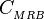 |
![\sum_{n=1}^{\infty} (-1)^n (n^{1/n}-1) = - \sqrt[1]{1} + \sqrt[2]{2} - \sqrt[3]{3} + \cdots](../I/m/37e54794ffbaae421fb075cb1af8f0be.png) |
Sum[n=1 to ∞] {(-1)^n (n^(1/n)-1)} |
[0;5,3,10,1,1,4,1,1,1,1,9,1,1,12,2,17,2,2,1,...] | 1999 | 0.18785964246206712024851793405427323 | ||
| 1.27323 95447 35162 68615 | Ramanujan–Forsyth series[146] | 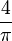 |
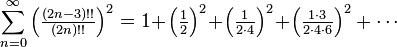 |
Sum[n=0 to ∞] {[(2n-3)!! /(2n)!!]^2} |
I | [1;3,1,1,1,15,2,72,1,9,1,17,1,2,1,5,1,1,10,...] | 1.27323954473516268615107010698011489 | |||
| 1.46707 80794 33975 47289 [Mw 122] | Porter Constant[147] | 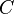 |
|
6*ln2/pi^2(3*ln2+ 4 EulerGamma- WeierstrassZeta'(2) *24/pi^2-2)-1/2 | [1;2,7,10,1,2,38,5,4,1,4,12,5,1,5,1,2,3,1,...] | 1974 | 1.46707807943397547289779848470722995 | |||
| 4.66920 16091 02990 67185 [Mw 123] | Feigenbaum constant δ [148] |  |
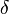 |
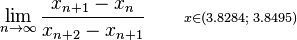
|
T | [4;1,2,43,2,163,2,3,1,1,2,5,1,2,3,80,2,5,...] | 1975 | 4.66920160910299067185320382046620161 | ||
| 2.50290 78750 95892 82228 [Mw 124] | Feigenbaum constant α[149] |  |
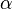 |
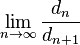 |
T ? | [2;1,1,85,2,8,1,10,16,3,8,9,2,1,40,1,2,3,...] | 1979 | 2.50290787509589282228390287321821578 | ||
| 0.62432 99885 43550 87099 [Mw 125] | Golomb–Dickman constant [150] | 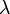 |
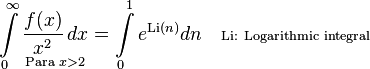 |
N[Int{n,0,1}[e^Li(n)],34] | [0;1,1,1,1,1,22,1,2,3,1,1,11,1,1,2,22,2,6,1,...] | 1930 & 1964 |
0.62432998854355087099293638310083724 | |||
| 23.14069 26327 79269 0057 [Mw 126] | Gelfond constant [151] | 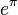 |
 |
Sum[n=0 to ∞] {(pi^n)/n!} |
T | [23;7,9,3,1,1,591,2,9,1,2,34,1,16,1,30,1,...] | 23.1406926327792690057290863679485474 | |||
| 7.38905 60989 30650 22723 | Conic constant, Schwarzschild constant [152] | 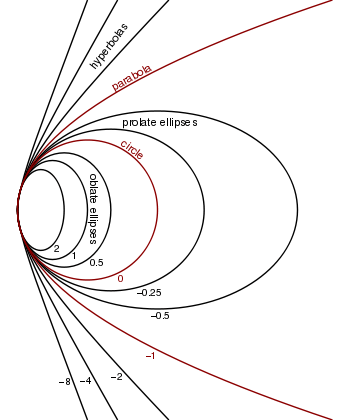 |
 |
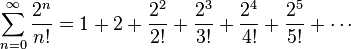 |
Sum[n=0 to ∞] {2^n/n!} |
T | [7;2,1,1,3,18,5,1,1,6,30,8,1,1,9,42,11,1,...] = [7,2,1,1,n,4*n+6,n+2], n = 3, 6, 9, etc. |
7.38905609893065022723042746057500781 | ||
| 0.35323 63718 54995 98454 [Mw 127] | Hafner–Sarnak–McCurley constant (1) [153] | 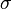 |
![\prod_{k=1}^{\infty}\left\{1-[1-\prod_{j=1}^n \underset{p_k: \text{ prime}}{(1-p_k^{-j})]^2}\right\}](../I/m/0610e4c349e18cf66dfbb8b3fac552d7.png) |
prod[k=1 to ∞] {1-(1-prod[j=1 to n] {1-ithprime(k)^-j})^2} | [0;2,1,4,1,10,1,8,1,4,1,2,1,2,1,2,6,1,1,1,3,...] | 1993 | 0.35323637185499598454351655043268201 | |||
| 0.60792 71018 54026 62866 [Mw 128] | Hafner–Sarnak–McCurley constant (2) [154] | 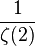 |
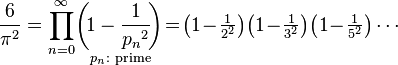 |
Prod{n=1 to ∞} (1-1/ithprime(n)^2) |
T | [0;1,1,1,1,4,2,4,7,1,4,2,3,4,10,1,2,1,1,1,...] | 0.60792710185402662866327677925836583 | |||
| 0.12345 67891 01112 13141 [Mw 129] | Champernowne constant [155] | 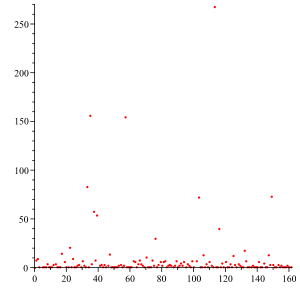 |
 |
 |
T | [0;8,9,1,149083,1,1,1,4,1,1,1,3,4,1,1,1,15,...] | 1933 | 0.12345678910111213141516171819202123 | ||
| 0.76422 36535 89220 66299 [Mw 130] | Landau-Ramanujan constant [156] | 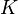 |
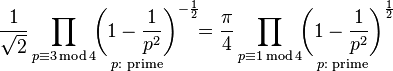 |
T ? | [0;1,3,4,6,1,15,1,2,2,3,1,23,3,1,1,3,1,1,6,4,...] | 0.76422365358922066299069873125009232 | ||||
| 1.92878 00... [Mw 131] | Wright constant [157] |  |
 |
[1; 1, 13, 24, 2, 1, 1, 3, 1, 1, 3] | 1.9287800... | |||||
| 2.71828 18284 59045 23536 [Mw 132] | Number e, Euler's number [158] |  |
 |
 |
Sum[n=0 to ∞] {1/n!} |
T | [2;1,2,1,1,4,1,1,6,1,1,8,1,1,10,1,1,12,1,...] = [2;1,2p,1], p∈ℕ |
2.71828182845904523536028747135266250 | ||
| 0.36787 94411 71442 32159 [Mw 133] | Inverse of Number e [159] |  |
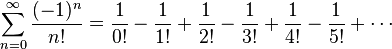 |
Sum[n=2 to ∞] {(-1)^n/n!} |
T | [0;2,1,1,2,1,1,4,1,1,6,1,1,8,1,1,10,1,1,12,...] = [0;2,1,1,2p,1], p∈ℕ |
1618 | 0.36787944117144232159552377016146086 | ||
| 0.69034 71261 14964 31946 | Upper iterated exponential [160] | 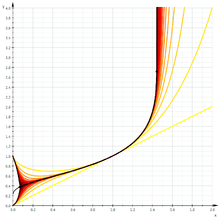 |
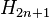 |
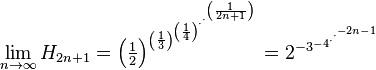 |
2^-3^-4^-5^-6^ -7^-8^-9^-10^ -11^-12^-13 … |
[0;1,2,4,2,1,3,1,2,2,1,4,1,2,4,3,1,1,10,1,3,2,...] | 0.69034712611496431946732843846418942 | |||
| 0.65836 55992 ... | Lower límit iterated exponential [161] | 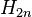 |
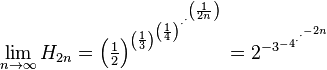 |
2^-3^-4^-5^-6^ -7^-8^-9^-10^ -11^-12 … |
[0;1,1,1,12,1,2,1,1,4,3,1,1,2,1,2,1,51,2,2,1,...] | 0.6583655992... | ||||
| 3.14159 26535 89793 23846 [Mw 134] | π number, Archimedes number [162] |  |
 |
Sum[n=0 to ∞] {(-1)^n 4/(2n+1)} |
T | [3;7,15,1,292,1,1,1,2,1,3,1,14,2,1,1,2,2,2,...] | 3.14159265358979323846264338327950288 | |||
| 0.46364 76090 00806 11621 | Machin–Gregory series[163] |  |
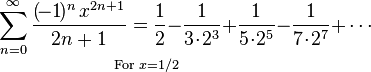 |
Sum[n=0 to ∞] {(-1)^n (1/2)^(2n+1) /(2n+1)} |
I | [0;2,6,2,1,1,1,6,1,2,1,1,2,10,1,2,1,2,1,1,1,...] | 0.46364760900080611621425623146121440 | |||
| 1.90216 05831 04 [Mw 135] | Brun 2 constant = Σ inverse of Twin primes [164] |  |
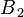 |
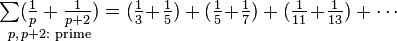 |
[1; 1, 9, 4, 1, 1, 8, 3, 4, 4, 2, 2] | 1.902160583104 | ||||
| 0.87058 83799 75 [Mw 136] | Brun 4 constant = Σ inv.prime quadruplets [165] | 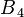 |

|
[0; 1, 6, 1, 2, 1, 2, 956, 3, 1, 1] | 0.870588379975 | |||||
| Buffon constant[166] |  |
 |
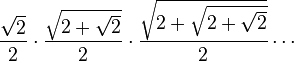 Viète product Viète product |
2/Pi | T | [0;1,1,1,3,31,1,145,1,4,2,8,1,6,1,2,3,1,4,...] | 1540 to 1603 |
0.63661977236758134307553505349005745 | ||
| 0.59634 73623 23194 07434 [Mw 138] | Euler–Gompertz constant [167] |  |
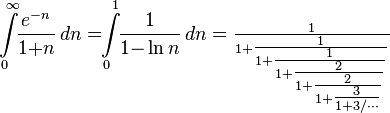 |
integral[0 to ∞] {(e^-n)/(1+n)} |
I | [0;1,1,2,10,1,1,4,2,2,13,2,4,1,32,4,8,1,1,1,...] | 0.59634736232319407434107849936927937 | |||
| Imaginary number [168] |  |
 |
 |
sqrt(-1) | C I | 1501 to 1576 |
||||
| 0.69777 46579 64007 98200 [Mw 140] | Continued fraction constant, Bessel function[169] |  |
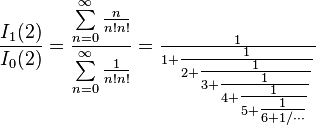 |
(Sum [n=0 to ∞] {n/(n!n!)}) / (Sum [n=0 to ∞] {1/(n!n!)}) |
I | [0;1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,...] = [0;p+1], p∈ℕ |
0.69777465796400798200679059255175260 | |||
| 2.74723 82749 32304 33305 | Ramanujan nested radical [170] |  |
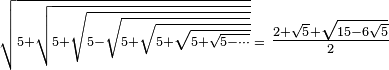 |
(2+sqrt(5) +sqrt(15 -6 sqrt(5)))/2 |
A | [2;1,2,1,21,1,7,2,1,1,2,1,2,1,17,4,4,1,1,4,2,...] | 2.74723827493230433305746518613420282 | |||
| 0.56714 32904 09783 87299 [Mw 141] | Omega constant, Lambert W function [171] |  |
 |
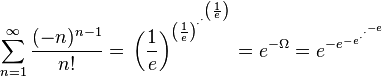 |
Sum[n=1 to ∞] {(-n)^(n-1)/n!} |
T | [0;1,1,3,4,2,10,4,1,1,1,1,2,7,306,1,5,1,2,1,...] | 0.56714329040978387299996866221035555 | ||
| 0.96894 61462 59369 38048 | Beta(3) [172] | 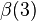 |
 |
Sum[n=1 to ∞] {(-1)^(n+1) /(-1+2n)^3} |
T | [0;1,31,4,1,18,21,1,1,2,1,2,1,3,6,3,28,1,...] | 0.96894614625936938048363484584691860 | |||
| 2.23606 79774 99789 69640 | Square root of 5, Gauss sum [173] |  |
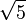 |
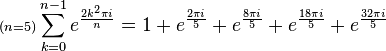 |
Sum[k=0 to 4] {e^(2k^2 pi i/5)} |
A | [2;4,4,4,4,4,4,4,4,4,4,4,4,4,4,4,4,4,4,4,4,...] = [2;4,...] |
2.23606797749978969640917366873127624 | ||
| 3.35988 56662 43177 55317 [Mw 142] | Prévost constant Reciprocal Fibonacci constant[174] | 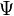 |
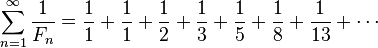
Fn: Fibonacci series |
Sum[n=1 to ∞] {1/Fibonacci[n]} |
I | [3;2,1,3,1,1,13,2,3,3,2,1,1,6,3,2,4,362,...] | ? | 3.35988566624317755317201130291892717 | ||
| 2.68545 20010 65306 44530 [Mw 143] | Khinchin's constant [175] |  |
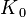 |
![\prod_{n=1}^\infty \left[{1+{1\over n(n+2)}}\right]^{\ln n/\ln 2}](../I/m/19064fb7149fa1652c2522ebc3e8cd75.png) |
Prod[n=1 to ∞] {(1+1/(n(n+2))) ^(ln(n)/ln(2))} |
T | [2;1,2,5,1,1,2,1,1,3,10,2,1,3,2,24,1,3,2,...] | 1934 | 2.68545200106530644530971483548179569 | |
See also
External links
- Inverse Symbolic Calculator, Plouffe's Inverter
- Constants - from Wolfram MathWorld
- Inverse symbolic calculator (CECM, ISC) (tells you how a given number can be constructed from mathematical constants)
- On-Line Encyclopedia of Integer Sequences (OEIS)
- Steven Finch's page of mathematical constants
- Xavier Gourdon and Pascal Sebah's page of numbers, mathematical constants and algorithms
Notes
- ↑ Steven R. Finch (2003). Cambridge University Press, ed. Mathematical Constants. p. 287. ISBN 3-540-67695-3.
- ↑ Dmitrii Kouznetsov (2009). Institute for Laser Science (ILS), (UEC). Japan, ed. SOLUTION OF F(z + 1) = exp F(z) IN COMPLEX z-PLANE (PDF).
- ↑ World eBooK Library (ed.). EULER'S INFINITE TETRATION THEOREM.
- ↑ Lloyd N. Trefethen (2013). SIAM., ed. Approximation Theory and Approximation Practice. p. 211. ISBN 978-1-611972-39-9.
- ↑ R. M. ABRAROV AND S. M. ABRAROV (2011). arxiv.org, ed. PROPERTIES AND APPLICATIONS OF THE PRIME DETECTING FUNCTION (PDF). p. 8.
- ↑ Sergey Kitaev and Toufik Mansour (2007). The problem of the pawns (PDF).
- ↑ Richard J. Mathar (2013). Circumscribed Regular Polygons (PDF). arxiv.org.
- ↑ Christoph Lanz. Technischen Universit¨at Wien, ed. k-Automatic Reals (PDF).
- ↑ NÚMERO DE BRONCE. PROPORCIÓN DE BRONCE (PDF).
- ↑ SERGI ELIZALDE. Arxiv.org, ed. ASYMPTOTIC ENUMERATION OF PERMUTATIONS AVOIDING GENERALIZED PATTERNS (PDF).
- ↑ David Cohen (2006). Thomson Learning Inc., ed. Precalculus: With Unit Circle Trigonometry. p. 328. ISBN 0-534-40230-5.
- ↑ Marek Wolf (2010). Two arguments that the nontrivial zeros of the Riemann zeta function are irrational (PDF). Instytut Fizyki Teoretycznej.
- ↑ DIVAKAR VISWANATH (1999). RANDOM FIBONACCI SEQUENCES AND THE NUMBER 1.13198824... (PDF). MATHEMATICS OF COMPUTATION.
- ↑ Helmut Brass,Knut Petras (2010). AMS, ed. Quadrature Theory: The Theory of Numerical Integration on a Compact Interval. p. p.274. ISBN 978-0-8218-5361-0.
- ↑ Steven Finch (2007). Harvard University, ed. Continued Fraction Transformation (PDF). p. 7.
- ↑ Clifford A. Pickover (2009). Sterling Publishing, ed. The Math Book. p. 266. ISBN 978-1-4027-5796-9.
- ↑ Steven Finch (2004). Harvard.edu, ed. Unitarism and Infinitarism (PDF). p. 1.
- ↑ Mireille Bousquet-Mélou. CNRS, LaBRI, Bordeaux, France, ed. Two-dimensional self-avoiding walks (PDF).
- ↑ Hugo Duminil-Copin and Stanislav Smirnov (2011). Universite de Geneve., ed. The connective constant of the honeycomb lattice √ (2 + √ 2) (PDF).
- ↑ W.A. Coppel (2000). Springer, ed. Number Theory: An Introduction to Mathematics. p. 480. ISBN 978-0-387-89485-0.
- ↑ James Stuart Tanton (2005). Encyclopedia of Mathematics. p. 529. ISBN 9781438110080.
- ↑ Robert Baillie (2013). arxiv, ed. Summing The Curious Series of Kempner and Irwin (PDF). p. 9.
- ↑ Leonhard Euler (1749). Consideratio quarumdam serierum, quae singularibus proprietatibus sunt praeditae. p. 108.
- ↑ Timothy Gowers, June Barrow-Green, Imre Leade (2007). Princeton University Press, ed. The Princeton Companion to Mathematics. p. 316. ISBN 978-0-691-11880-2.
- ↑ Vijaya AV (2007). Dorling Kindcrsley (India) Pvt. Lid., ed. Figuring Out Mathematics. p. 15. ISBN 978-81-317-0359-5.
- ↑ Steven Finch (2014). Harvard.edu, ed. Errata and Addenda to Mathematical Constants (PDF). p. 59.
- ↑ Steven Finch (2007). Harvard.edu, ed. Series involving Arithmetric Functions (PDF). p. 1.
- ↑ Nayar. Tata McGraw-Hill Education., ed. The Steel Handbook. p. 953.
- ↑ ECKFORD COHEN (1962). University of Tennessee, ed. SOME ASYMPTOTIC FORMULAS IN THE THEORY OF NUMBERS (PDF). p. 220.
- ↑ Paul B. Slater (2013). University of California, ed. A Hypergeometric Formula ... (PDF). p. 9.
- ↑ Ivan Niven. Averages of exponents in factoring integers (PDF).
- ↑ Steven Finch (2014). Harvard.edu, ed. Errata and Addenda to Mathematical Constants (PDF).
- ↑ Steven Finch (2014). Harvard.edu, ed. Errata and Addenda to Mathematical Constants (PDF). p. 53.
- ↑ FRANZ LEMMERMEYER (2003). arxiv.org, ed. HIGHER DESCENT ON PELL CONICS. I. FROM LEGENDRE TO SELMER (PDF). p. 13.
- ↑ Howard Curtis (2014). Elsevier, ed. Orbital Mechanics for Engineering Students. p. 159. ISBN 978-0-08-097747-8.
- ↑ Andrew Granville and K. Soundararajan (1999). Arxiv, ed. The spectrum of multiplicative functions (PDF). p. 3.
- ↑ John Horton Conway, Richard K. Guy (1995). Copernicus, ed. The Book of Numbers. p. 242. ISBN 0-387-97993-X.
- ↑ John Derbyshire (2003). Joseph Henry Press, ed. Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics. p. 147. ISBN 0-309-08549-7.
- ↑ Annie Cuyt, Vigdis Brevik Petersen, Brigitte Verdonk, Haakon Waadelantl, William B. Jones. (2008). Handbook of Continued Fractions for Special Functions. Springer. p. 188. ISBN 978-1-4020-6948-2.
- ↑ Henri Cohen (2000). Number Theory: Volume II: Analytic and Modern Tools. Springer. p. 127. ISBN 978-0-387-49893-5.
- ↑ H. M. Srivastava,Choi Junesang (2001). Series Associated With the Zeta and Related Functions. Kluwer Academic Publishers. p. 30. ISBN 0-7923-7054-6.
- ↑ E. Catalan (1864). Mémoire sur la transformation des séries, et sur quelques intégrales définies, Comptes rendus hebdomadaires des séances de l’Académie des sciences 59. Kluwer Academic éditeurs. p. 618.
- ↑ Lennart Råde,Bertil (2000). Springer-Verlag, ed. Mathematics Handbook for Science and Engineering. p. 423. ISBN 3-540-21141-1.
- ↑ J. B. Friedlander, A. Perelli, C. Viola, D.R. Heath-Brown, H.Iwaniec, J. Kaczorowski (2002). Springer, ed. Analytic Number Theory. p. 29. ISBN 978-3-540-36363-7.
- ↑ William Dunham (2005). Princeton University Press, ed. The Calculus Gallery: Masterpieces from Newton to Lebesgue. p. 51. ISBN 978-0-691-09565-3.
- ↑ Jean Jacquelin (2010). SOPHOMORE'S DREAM FUNCTION.
- ↑ Simon Plouffe. Sum of the product of inverse of primes.
- ↑ Simon Plouffe (1998). Université du Québec à Montréal, ed. The Computation of Certain Numbers Using a Ruler and Compass. p. Vol. 1 (1998), Article 98.1.3.
- ↑ John Srdjan Petrovic (2014). CRC Press, ed. Advanced Calculus: Theory and Practice. p. 65. ISBN 978-1-4665-6563-0.
- ↑ Steven R. Finch (1999). Several Constants Arising in Statistical Mechanics (PDF). p. 5.
- ↑ Federico Ardila, Richard Stanley. Department of Mathematics, MIT, Cambridge, ed. Several Constants Arising in Statistical Mechanics (PDF).
- ↑ Andrija S. Radovic. A REPRESENTATION OF FACTORIAL FUNCTION, THE NATURE OF CONSTAT AND A WAY FOR SOLVING OF FUNCTIONAL EQUATION F(x) = x . F(x - 1) (PDF).
- ↑ Kunihiko Kaneko,Ichiro Tsuda (1997). Complex Systems: Chaos and Beyond. p. 211. ISBN 3-540-67202-8.
- ↑ Steven Finch (2005). Harvard University, ed. Minkowski-Siegel Mass Constants (PDF). p. 5.
- ↑ University of Florida, Department of Mechanical and Aerospace Engineering (ed.). Evaluation of the complete elliptic integrals by the agm method (PDF).
- ↑ Steven R. Finch (2003). Cambridge University Press, ed. Mathematical Constants. p. 479. ISBN 3-540-67695-3.
- ↑ Facts On File, Incorporated (1997). Mathematics Frontiers. p. 46. ISBN 978-0-8160-5427-5.
- ↑ Aleksandr I͡Akovlevich Khinchin (1997). Courier Dover Publications, ed. Continued Fractions. p. 66. ISBN 978-0-486-69630-0.
- ↑ Jean-Pierre Serre (1969–1970). Travaux de Baker (PDF). NUMDAM, Séminaire N. Bourbaki. p. 74.
- ↑ Julian Havil (2003). Gamma: Exploring Euler's Constant. Princeton University Press. p. 31. ISBN 9780691141336.
- ↑ Horst Alzer (2002). Journal of Computational and Applied Mathematics, Volume 139, Issue 2 (PDF). Elsevier. pp. 215–230.
- ↑ Steven R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 238. ISBN 3-540-67695-3.
- ↑ Steven Finch (2007). Continued Fraction Transformation III (PDF). Harvard University. p. 5.
- ↑ Andrei Vernescu (2007). Gazeta Matematica Seria a revista de cultur Matematica Anul XXV(CIV)Nr. 1, Constante de tip Euler generalizate (PDF). p. 14.
- ↑ Mauro Fiorentini. Nielsen – Ramanujan (costanti di).
- ↑ Annie Cuyt, Vigdis Brevik Petersen, Brigitte Verdonk, Haakon Waadeland, William B. Jones (2008). Handbook of Continued Fractions for Special Functions. Springer. p. 182. ISBN 978-1-4020-6948-2.
- ↑ Eric W. Weisstein (2003). CRC Concise Encyclopedia of Mathematics, Second Edition. CRC Press. p. 151. ISBN 1-58488-347-2.
- ↑ P. HABEGGER (2003). MULTIPLICATIVE DEPENDENCE AND ISOLATION I (PDF). Institut für Mathematik, Universit¨at Basel, Rheinsprung Basel, Switzerland. p. 2.
- ↑ Steven Finch (2005). Class Number Theory (PDF). Harvard University. p. 8.
- ↑ James Stewart (2010). Single Variable Calculus: Concepts and Contexts. Brooks/Cole. p. 314. ISBN 978-0-495-55972-6.
- ↑ Steven Finch (2005). Class Number Theory (PDF). Harvard University. p. 8.
- ↑ Steven R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 421. ISBN 3-540-67695-3.
- ↑ Steven R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 122. ISBN 3-540-67695-3.
- ↑ Jorg Waldvogel (2008). Analytic Continuation of the Theodorus Spiral (PDF). p. 16.
- ↑ Robert Kaplan,Ellen Kaplan (2014). Oxford University Press, Bloomsburv Press, ed. The Art of the Infinite: The Pleasures of Mathematics. p. 238. ISBN 978-1-60819-869-6.
- ↑ Steven R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 121. ISBN 3-540-67695-3.
- ↑ Eric W. Weisstein (2002). CRC Concise Encyclopedia of Mathematics, Second Edition. CRC Press. p. 1356.
- ↑ Chebfun Team (2010). Lebesgue functions and Lebesgue constants. MATLAB Central.
- ↑ Simon J. Smith (2005). Lebesgue constants in polynomial interpolation. La Trobe University, Bendigo, Australia.
- ↑ D. R. Woodall (2005). University of Nottingham, ed. CHROMATIC POLYNOMIALS OF PLANE TRIANGULATIONS (PDF). p. 5.
- ↑ Benjamin Klopsch (2013). NOTE DI MATEMATICA: Representation growth and representation zeta functions of groups (PDF). Universita del Salento. p. 114. ISSN 1590-0932.
- ↑ Nikos Bagis. Some New Results on Prime Sums (3 The Euler Totient constant) (PDF). Aristotle University of Thessaloniki. p. 8.
- ↑ Robinson, H.P. (1971–2011). MATHEMATICAL CONSTANTS. Lawrence Berkeley National Laboratory. p. 40.
- ↑ Annmarie McGonagle (2011). A New Parameterization for Ford Circles (PDF). Plattsburgh State University of New York.
- ↑ V. S. Varadarajan (2000). Euler Through Time: A New Look at Old Themes. AMS. ISBN 0-8218-3580-7.
- ↑ Leonhard Euler, Joseph Louis Lagrange (1810). Elements of Algebra, Volumen 1. J. Johnson and Company. p. 333.
- ↑ Ian Stewart (1996). Professor Stewart's Cabinet of Mathematical Curiosities. Birkhäuser Verlag. ISBN 978-1-84765-128-0.
- ↑ H.M. Antia (2000). Numerical Methods for Scientists and Engineers. Birkhäuser Verlag. p. 220. ISBN 3-7643-6715-6.
- ↑ Francisco J. Aragón Artacho, David H. Baileyy, Jonathan M. Borweinz, Peter B. Borwein (2012). Tools for visualizing real numbers. (PDF). p. 33.
- ↑ Papierfalten (PDF). 1998.
- ↑ Sondow, Jonathan; Hadjicostas, Petros (2008). "The generalized-Euler-constant function γ(z) and a generalization of Somos's quadratic recurrence constant". Journal of Mathematical Analysis and Applications 332: 292–314. arXiv:math/0610499. doi:10.1016/j.jmaa.2006.09.081.
- ↑ J. Sondow. Generalization of Somos Quadratic (PDF).
- ↑ Chan Wei Ting ... Moire patterns + fractals (PDF). p. 16.
- ↑ Christoph Zurnieden (2008). Descriptions of the Algorithms (PDF).
- ↑ Julian Havil (2003). Gamma: Exploring Euler's Constant. Princeton University Press. p. 64. ISBN 9780691141336.
- ↑ Steven R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 466. ISBN 3-540-67695-3.
- ↑ James Stuart Tanton (2007). Encyclopedia of Mathematics. p. 458. ISBN 0-8160-5124-0.
- ↑ Calvin C. Clawson (2003). Mathematical Traveler: Exploring the Grand History of Numbers. Perseus. p. 187. ISBN 0-7382-0835-3.
- ↑ Jonathan Sondowa, Diego Marques (2010). Algebraic and transcendental solutions of some exponential equations (PDF). Annales Mathematicae et Informaticae.
- ↑ T. Piezas. Tribonacci constant & Pi.
- ↑ Steven Finch. Addenda to Mathematical Constants (PDF).
- ↑ Renzo Sprugnoli. Introduzione alla Matematica (PDF).
- ↑ Chao-Ping Chen. Ioachimescu's constant (PDF).
- ↑ R. A. Knoebel. Exponentials Reiterated (PDF). Maa.org.
- ↑ Eric W. Weisstein (2003). CRC Concise Encyclopedia of Mathematics, Second Edition. CRC Press. p. 1688. ISBN 1-58488-347-2.
- ↑ Richard J.Mathar. Table of Dirichlet L-series and Prime Zeta (PDF). Arxiv.
- ↑ Eric W. Weisstein (2003). CRC Concise Encyclopedia of Mathematics, Second Edition. CRC Press. p. 151. ISBN 1-58488-347-2.
- ↑ Dave Benson (2006). Music: A Mathematical Offering. Cambridge University Press. p. 53. ISBN 978-0-521-85387-3.
- ↑ Yann Bugeaud (2012). Distribution Modulo One and Diophantine Approximation. Cambridge University Press. p. 87. ISBN 978-0-521-11169-0.
- ↑ Angel Chang y Tianrong Zhang. On the Fractal Structure of the Boundary of Dragon Curve.
- ↑ Joe Diestel (1995). Absolutely Summing Operators. Cambridge University Press. p. 29. ISBN 0-521-43168-9.
- ↑ Eric W. Weisstein (2002). CRC Concise Encyclopedia of Mathematics, Second Edition. CRC Press. p. 1356. ISBN 1-58488-347-2.
- ↑ Laith Saadi (2004). Stealth Ciphers. Trafford Publishing. p. 160. ISBN 978-1-4120-2409-9.
- ↑ Jonathan Borwein,David Bailey (2008). Mathematics by Experiment, 2nd Edition: Plausible Reasoning in the 21st Century. A K Peters, Ltd. p. 56. ISBN 978-1-56881-442-1.
- ↑ L. J. Lloyd James Peter Kilford (2008). Modular Forms: A Classical and Computational Introduction. Imperial College Press. p. 107. ISBN 978-1-84816-213-6.
- ↑ Continued Fractions from Euclid till Present. IHES, Bures sur Yvette. 1998.
- ↑ Julian Havil (2012). The Irrationals: A Story of the Numbers You Can't Count On. Princeton University Press. p. 98. ISBN 978-0-691-14342-2.
- ↑ Lennart R©Æde,Bertil Westergren (2004). Mathematics Handbook for Science and Engineering. Springer-Verlag. p. 194. ISBN 3-540-21141-1.
- ↑ Michael Trott. Finding Trott Constants (PDF). Wolfram Research.
- ↑ David Darling (2004). The Universal Book of Mathematics: From Abracadabra to Zeno's Paradoxes. Wiley & Sons inc. p. 63. ISBN 0-471-27047-4.
- ↑ Keith B. Oldham,Jan C. Myland,Jerome Spanier (2009). An Atlas of Functions: With Equator, the Atlas Function Calculator. Springer. p. 15. ISBN 978-0-387-48806-6.
- ↑ Johann Georg Soldner (1809). Lindauer, München, ed. Théorie et tables d’une nouvelle fonction transcendante (in French). p. 42.
- ↑ Lorenzo Mascheroni (1792). Petrus Galeatius, Ticini, ed. Adnotationes ad calculum integralem Euleri (in Latin). p. 17.
- ↑ Yann Bugeaud (2004). Series representations for some mathematical constants. p. 72. ISBN 0-521-82329-3.
- ↑ Calvin C Clawson (2001). Mathematical sorcery: revealing the secrets of numbers. p. IV. ISBN 978 0 7382 0496-3.
- ↑ H.M. Antia (2000). Numerical Methods for Scientists and Engineers. Birkhäuser Verlag. p. 220. ISBN 3-7643-6715-6.
- ↑ Bart Snapp (2012). Numbers and Algebra (PDF).
- ↑ George Gheverghese Joseph (2011). The Crest of the Peacock: Non-European Roots of Mathematics. Princeton University Press. p. 295. ISBN 978-0-691-13526-7.
- ↑ Steven Finch. Volumes of Hyperbolic 3-Manifolds (PDF). Harvard University.
- ↑ J. Coates,Martin J. Taylor (1991). L-Functions and Arithmetic. Cambridge University Press. p. 333. ISBN 0-521-38619-5.
- ↑ Jan Feliksiak (2013). The Symphony of Primes, Distribution of Primes and Riemann’s Hypothesis. Xlibris Corporation. p. 18. ISBN 978-1-4797-6558-4.
- ↑ Horst Alzera, Dimitri Karayannakisb, H.M. Srivastava (2005). Series representations for some mathematical constants. Elsevier Inc. p. 149.
- ↑ Steven R. Finch (2005). Quadratic Dirichlet L-Series (PDF). p. 12.
- ↑ H. M. Srivastava,Junesang Choi (2012). Zeta and q-Zeta Functions and Associated Series and Integrals. Elsevier. p. 613. ISBN 978-0-12-385218-2.
- ↑ Refaat El Attar (2006). Special Functions And Orthogonal Polynomials. Lulu Press. p. 58. ISBN 1-4116-6690-9.
- ↑ Jesus Guillera and Jonathan Sondow. arxiv.org, ed. Double integrals and infinite products... (PDF).
- ↑ Andras Bezdek (2003). Discrete Geometry. Marcel Dekkcr, Inc. p. 150. ISBN 0-8247-0968-3.
- ↑ Weisstein, Eric W. Rényi's Parking Constants. MathWorld. p. (4).
- ↑ Eli Maor (2006). e: The Story of a Number. Princeton University Press. ISBN 0-691-03390-0.
- ↑ Clifford A. Pickover (2005). A Passion for Mathematics. John Wiley & Sons, Inc. p. 90. ISBN 0-471-69098-8.
- ↑ Steven R. Finch (2003). Mathematical Constants. Cambridge University Press. p. 322. ISBN 3-540-67695-3.
- ↑ Eric W. Weisstein (2003). CRC Concise Encyclopedia of Mathematics, Second Edition. CRC Press. p. 1688. ISBN 1-58488-347-2.
- ↑ Richard E. Crandall (2012). Unified algorithms for polylogarithm, L-series, and zeta variants (PDF). perfscipress.com.
- ↑ RICHARD J. MATHAR (2010). NUMERICAL EVALUATION OF THE OSCILLATORY INTEGRAL OVER exp(I pi x)x^1/x BETWEEN 1 AND INFINITY (PDF). http://arxiv.org/abs/0912.3844.
- ↑ M.R.Burns (1999). Root constant. http://marvinrayburns.com/.
- ↑ H. K. Kuiken (2001). Practical Asymptotics. KLUWER ACADEMIC PUBLISHERS. p. 162. ISBN 0-7923-6920-3.
- ↑ Michel A. Théra (2002). Constructive, Experimental, and Nonlinear Analysis. CMS-AMS. p. 77. ISBN 0-8218-2167-9.
- ↑ Kathleen T. Alligood (1996). Chaos: An Introduction to Dynamical Systems. Springer. ISBN 0-387-94677-2.
- ↑ K. T. Chau,Zheng Wang (201). Chaos in Electric Drive Systems: Analysis, Control and Application. John Wiley & Son. p. 7. ISBN 978-0-470-82633-1.
- ↑ Eric W. Weisstein (2002). CRC Concise Encyclopedia of Mathematics. Crc Press. p. 1212.
- ↑ David Wells (1997). The Penguin Dictionary of Curious and Interesting Numbers. Penguin Books Ltd. p. 4.
- ↑ Jvrg Arndt,Christoph Haenel. [http://books.google.com/?id=mchJCvIsSXwC&pg=PA67&dq=7.38905#v=onepage&q=7.38905&f=false v Pi: Algorithmen, Computer, Arithmetik]. Springer. p. 67. ISBN 3-540-66258-8.
- ↑ Steven R. Finch (2003). Mathematical Constants. p. 110. ISBN 3-540-67695-3.
- ↑ Holger Hermanns,Roberto Segala (2000). Process Algebra and Probabilistic Methods. Springer-Verlag. p. 270. ISBN 3-540-67695-3.
- ↑ Michael J. Dinneen,Bakhadyr Khoussainov,Prof. Andre Nies (2012). Computation, Physics and Beyond. Springer. p. 110. ISBN 978-3-642-27653-8.
- ↑ Richard E. Crandall,Carl B. Pomerance (2005). Prime Numbers: A Computational Perspective. Springer. p. 80. ISBN 978-0387-25282-7.
- ↑ Paulo Ribenboim (2000). My Numbers, My Friends: Popular Lectures on Number Theory. Springer-Verlag. p. 66. ISBN 0-387-98911-0.
- ↑ E.Kasner y J.Newman. (2007). Mathematics and the Imagination. Conaculta. p. 77. ISBN 978-968-5374-20-0.
- ↑ Eli Maor (1994). "e": The Story of a Number. Princeton University Press. p. 37. ISBN 978-0-691-14134-3.
- ↑ Theo Kempermann (2005). Zahlentheoretische Kostproben. Freiburger graphische betriebe. p. 139. ISBN 3-8171-1780-9.
- ↑ Steven Finch (2003). Mathematical Constants. Cambridge University Press. p. 449. ISBN 0-521-81805-2.
- ↑ Michael Trott (2004). The Mathematica GuideBook for Programming. Springer Science. p. 173. ISBN 0-387-94282-3.
- ↑ John Horton Conway, Richard K. Guy. (1995). The Book of Numbers. Copernicus. p. 242. ISBN 0-387-97993-X.
- ↑ Thomas Koshy (2007). Elementary Number Theory with Applications. Elsevier. p. 119. ISBN 978-0-12-372-487-8.
- ↑ Pascal Sebah and Xavier Gourdon (2002). Introduction to twin primes and Brun’s constant computation (PDF).
- ↑ Jorg Arndt,Christoph Haenel (2000). Pi -- Unleashed. Verlag Berlin Heidelberg. p. 13. ISBN 3-540-66572-2.
- ↑ Annie Cuyt, Viadis Brevik Petersen, Brigitte Verdonk, William B. Jones (2008). Handbook of continued fractions for special functions. Springer Science. p. 190. ISBN 978-1-4020-6948-2.
- ↑ Keith J. Devlin (1999). Mathematics: The New Golden Age. Columbia University Press. p. 66. ISBN 0-231-11638-1.
- ↑ Simon Plouffe. Miscellaneous Mathematical Constants.
- ↑ Bruce C. Berndt,Robert Alexander Rankin (2001). Ramanujan: essays and surveys. American Mathematical Society, London Mathematical Society. p. 219. ISBN 0-8218-2624-7.
- ↑ Albert Gural. Infinite Power Towers.
- ↑ Michael A. Idowu (2012). Fundamental relations between the Dirichlet beta function, euler numbers, and Riemann zeta function for positive integers. arXiv:1210.5559. p. 1.
- ↑ P A J Lewis (2008). Essential Mathematics 9. Ratna Sagar. p. 24. ISBN 9788183323673.
- ↑ Gérard P. Michon (2005). Numerical Constants. Numericana.
- ↑ Julian Havil (2003). Gamma: Exploring Euler's Constant. Princeton University Press. p. 161. ISBN 9780691141336.
Site MathWorld Wolfram.com
- ↑ Weisstein, Eric W., "Goh-Schmutz Constant", MathWorld.
- ↑ Weisstein, Eric W., "Bernstein´s Constant", MathWorld.
- ↑ Weisstein, Eric W., "Twin Primes Constant", MathWorld.
- ↑ Weisstein, Eric W., "Fibonacci Factorial Constant", MathWorld.
- ↑ Weisstein, Eric W., "Polygon Inscribing", MathWorld.
- ↑ Weisstein, Eric W., "Komornik-Loreti Constant", MathWorld.
- ↑ Weisstein, Eric W., "Silver Ratio", MathWorld.
- ↑ Weisstein, Eric W., "Disk Covering Problem", MathWorld.
- ↑ Weisstein, Eric W., "Gelfond-Schneider Constant", MathWorld.
- ↑ Weisstein, Eric W., "Levy Constant", MathWorld.
- ↑ Weisstein, Eric W., "Chi", MathWorld.
- ↑ Weisstein, Eric W., "Random Fibonacci Sequence", MathWorld.
- ↑ Weisstein, Eric W., "Favard Constants", MathWorld.
- ↑ Weisstein, Eric W., "Lochs' Constant", MathWorld.
- ↑ Weisstein, Eric W., "Reuleaux Triangle", MathWorld.
- ↑ Weisstein, Eric W., "Self-Avoiding Walk Connective Constant", MathWorld.
- ↑ Weisstein, Eric W., "Gauss-Kuzmin-Wirsing Constant", MathWorld.
- ↑ Weisstein, Eric W., "Wallis Formula", MathWorld.
- ↑ Weisstein, Eric W., "Erdos-Borwein Constant", MathWorld.
- ↑ Weisstein, Eric W., "Golden Ratio", MathWorld.
- ↑ Weisstein, Eric W., "Riemann Zeta Function Zeta 2", MathWorld.
- ↑ Weisstein, Eric W., "Theodorus's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Nested Radical Constant", MathWorld.
- ↑ Weisstein, Eric W., "Universal Parabolic Constant", MathWorld.
- ↑ Weisstein, Eric W., "Silverman Constant", MathWorld.
- ↑ Weisstein, Eric W., "Twenty-Vertex Entropy Constant", MathWorld.
- ↑ Weisstein, Eric W., "Feller-Tornier Constant", MathWorld.
- ↑ Weisstein, Eric W., "Baxter's Four-Coloring Constant", MathWorld.
- ↑ Weisstein, Eric W., "Tetranacci Constant", MathWorld.
- ↑ Weisstein, Eric W., "Prince Rupert's Cube", MathWorld.
- ↑ Weisstein, Eric W., "Niven's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Central Binomial Coefficient", MathWorld.
- ↑ Weisstein, Eric W., "Hermite Constants", MathWorld.
- ↑ Weisstein, Eric W., "Thue-Morse Constant", MathWorld.
- ↑ Weisstein, Eric W., "Pell Constant", MathWorld.
- ↑ Weisstein, Eric W., "Laplace Limit", MathWorld.
- ↑ Weisstein, Eric W., "Hall-Montgomery Constant", MathWorld.
- ↑ Weisstein, Eric W., "Calabi's Triangle", MathWorld.
- ↑ Weisstein, Eric W., "Gamma Function", MathWorld.
- ↑ Weisstein, Eric W., "Apery's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Catalan's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Dirichlet Beta Function", MathWorld.
- ↑ Weisstein, Eric W., "Heath-Brown-Moroz Constant", MathWorld.
- ↑ Weisstein, Eric W., "Sophomore's Dream", MathWorld.
- ↑ Weisstein, Eric W., "Sophomore's Dream", MathWorld.
- ↑ Weisstein, Eric W., "Primorial", MathWorld.
- ↑ Weisstein, Eric W., "Plouffe's Constants", MathWorld.
- ↑ Weisstein, Eric W., "Plouffe's Constants", MathWorld.
- ↑ Weisstein, Eric W., "Domino Tiling", MathWorld.
- ↑ Weisstein, Eric W., "Wallis's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Sarnak's Constant", MathWorld.
- ↑ Weisstein, Eric W., "e", MathWorld.
- ↑ Weisstein, Eric W., "Lemniscate Constant", MathWorld.
- ↑ Weisstein, Eric W., "Robbins Constant", MathWorld.
- ↑ Weisstein, Eric W., "Conway's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Levy Constant", MathWorld.
- ↑ Weisstein, Eric W., "Kempner Series", MathWorld.
- ↑ Weisstein, Eric W., "Lebesgue Constants", MathWorld.
- ↑ Weisstein, Eric W., "Du Bois Reymond Constants", MathWorld.
- ↑ Weisstein, Eric W., "Lüroth's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Foias Constant", MathWorld.
- ↑ Weisstein, Eric W., "Foias Constant", MathWorld.
- ↑ Weisstein, Eric W., "Nielsen-Ramanujan Constants", MathWorld.
- ↑ Weisstein, Eric W., "Natural Logarithm of 2", MathWorld.
- ↑ Weisstein, Eric W., "Weierstrass Constant", MathWorld.
- ↑ Weisstein, Eric W., "Euler-Mascheroni Constant", MathWorld.
- ↑ Weisstein, Eric W., "Golden Spiral", MathWorld.
- ↑ Weisstein, Eric W., "Stephen's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Dottie Number", MathWorld.
- ↑ Weisstein, Eric W., "Euler Product", MathWorld.
- ↑ Weisstein, Eric W., "Lemniscate Case", MathWorld.
- ↑ Weisstein, Eric W., "i", MathWorld.
- ↑ Weisstein, Eric W., "Alladi-Grinstead Constants", MathWorld.
- ↑ Weisstein, Eric W., "Sierpinski Constant", MathWorld.
- ↑ Weisstein, Eric W., "Lebesgue Constants", MathWorld.
- ↑ Weisstein, Eric W., "Silver Constant", MathWorld.
- ↑ Weisstein, Eric W., "Totient Summatory Function", MathWorld.
- ↑ Weisstein, Eric W., "Ford Circle", MathWorld.
- ↑ Weisstein, Eric W., "Riemann Zeta Function", MathWorld.
- ↑ Weisstein, Eric W., "Plastic Constant", MathWorld.
- ↑ Weisstein, Eric W., "Paper Folding Constant", MathWorld.
- ↑ Weisstein, Eric W., "Paper Folding Constant", MathWorld.
- ↑ Weisstein, Eric W., "Koch Snowflake", MathWorld.
- ↑ Weisstein, Eric W., "Mertens Constant", MathWorld.
- ↑ Weisstein, Eric W., "Liouville's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Power Tower", MathWorld.
- ↑ Weisstein, Eric W., "Power Tower", MathWorld.
- ↑ Weisstein, Eric W., "Masser-Gramain Constant", MathWorld.
- ↑ Weisstein, Eric W., "Bifoliate", MathWorld.
- ↑ Weisstein, Eric W., "Backhouse's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Wilbraham-Gibbs Constant", MathWorld.
- ↑ Weisstein, Eric W., "Copeland-Erdos Constant", MathWorld.
- ↑ Weisstein, Eric W., "Dragon Curve", MathWorld.
- ↑ Weisstein, Eric W., "Grothendieck's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Pascal's Triangle", MathWorld.
- ↑ Weisstein, Eric W., "Mills Constant", MathWorld.
- ↑ Weisstein, Eric W., "Figure Eight Knot", MathWorld.
- ↑ Weisstein, Eric W., "Ramanujan Constant", MathWorld.
- ↑ Weisstein, Eric W., "KhinchinHarmonicMean", MathWorld.
- ↑ Weisstein, Eric W., "Riemann Zeta Constant", MathWorld.
- ↑ Weisstein, Eric W., "Trott Constant", MathWorld.
- ↑ Weisstein, Eric W., "Chaitin's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Gauss's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Soldner's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Cahen's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Pythagoras's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Carlson-Levin Constant", MathWorld.
- ↑ Weisstein, Eric W., "Gieseking's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Lemniscate Constant", MathWorld.
- ↑ Weisstein, Eric W., "Glaisher-Kinkelin Constant", MathWorld.
- ↑ Weisstein, Eric W., "Gausss Digamma Theorem", MathWorld.
- ↑ Weisstein, Eric W., "Carefree Couple", MathWorld.
- ↑ Weisstein, Eric W., "Euler-Mascheroni Constant", MathWorld.
- ↑ Weisstein, Eric W., "Gamma Function", MathWorld.
- ↑ Weisstein, Eric W., "SomossQuadraticRecurrence Constant", MathWorld.
- ↑ Weisstein, Eric W., "Renyi's Parking Constant", MathWorld.
- ↑ Weisstein, Eric W., "Steiner's Problem", MathWorld.
- ↑ Weisstein, Eric W., "Power Tower", MathWorld.
- ↑ Weisstein, Eric W., "Polya's Random Walk Constant", MathWorld.
- ↑ Weisstein, Eric W., "Landau Constant", MathWorld.
- ↑ Weisstein, Eric W., "MRB Constant", MathWorld.
- ↑ Weisstein, Eric W., "Porter's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Feigenbaum Constant", MathWorld.
- ↑ Weisstein, Eric W., "Feigenbaum Constant", MathWorld.
- ↑ Weisstein, Eric W., "Golomb-Dickman Constant", MathWorld.
- ↑ Weisstein, Eric W., "Gelfonds Constant", MathWorld.
- ↑ Weisstein, Eric W., "Hafner-Sarnak-McCurley Constant", MathWorld.
- ↑ Weisstein, Eric W., "Relatively Prime", MathWorld.
- ↑ Weisstein, Eric W., "Champernowne Constant", MathWorld.
- ↑ Weisstein, Eric W., "Landau-Ramanujan Constant", MathWorld.
- ↑ Weisstein, Eric W., "PrimeFormulas", MathWorld.
- ↑ Weisstein, Eric W., "e", MathWorld.
- ↑ Weisstein, Eric W., "Factorial Sums", MathWorld.
- ↑ Weisstein, Eric W., "Pi Formulas", MathWorld.
- ↑ Weisstein, Eric W., "Brun's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Brun's Constant", MathWorld.
- ↑ Weisstein, Eric W., "Prime Products", MathWorld.
- ↑ Weisstein, Eric W., "Gompertz Constant", MathWorld.
- ↑ Weisstein, Eric W., "i", MathWorld.
- ↑ Weisstein, Eric W., "ContinuedFraction Constant", MathWorld.
- ↑ Weisstein, Eric W., "Omega Constant", MathWorld.
- ↑ Weisstein, Eric W., "Reciprocal Fibonacci Constant", MathWorld.
- ↑ Weisstein, Eric W., "Khinchin's Constant", MathWorld.

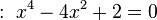
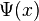 is an analytic function with
is an analytic function with 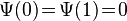 .
.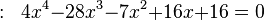
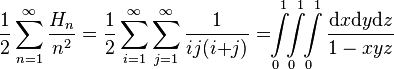

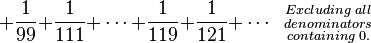
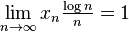
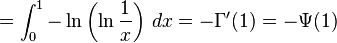
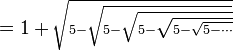
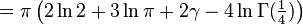
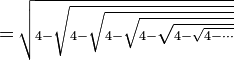
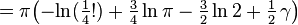
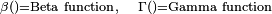

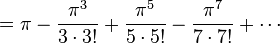

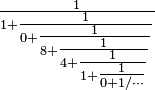
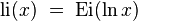 Ei =
Ei = 

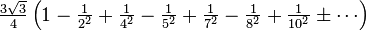 .
.