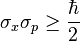Madelung equations
The Madelung equations are Erwin Madelung's equivalent alternative formulation of the Schrödinger equation.
Equations
The Madelung equations[1] [2] are quantum Euler equations:
where  is the flow velocity in the quantum probability space with mass density
is the flow velocity in the quantum probability space with mass density  . The circulation
of the flow velocity field along any closed path obeys the auxiliary condition[3]
. The circulation
of the flow velocity field along any closed path obeys the auxiliary condition[3]
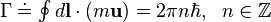 . The term in the brackets represents a quantum chemical potential. The kinetic energy operator from the Hamiltonian
. The term in the brackets represents a quantum chemical potential. The kinetic energy operator from the Hamiltonian 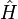 results in a non-local quantum pressure tensor
results in a non-local quantum pressure tensor
or alternatively to the Bohm quantum potential. While the latter is the icon of the de Broglie–Bohm theory, the quantum symbol of the Madelung hydrodynamics is  . The integral energy stored in the quantum pressure tensor is proportional to the Fisher information, which accounts for the quality of measurements. Hence, according to the Cramér–Rao bound, the Heisenberg Uncertainty principle is equivalent to a standard inequality for the efficiency (statistics) of measurements.[4]
. The integral energy stored in the quantum pressure tensor is proportional to the Fisher information, which accounts for the quality of measurements. Hence, according to the Cramér–Rao bound, the Heisenberg Uncertainty principle is equivalent to a standard inequality for the efficiency (statistics) of measurements.[4]
See also
- Quantum potential
- Quantum hydrodynamics
- Bohmian quantum mechanics
References
- ↑ Madelung, E. (1926). "Eine anschauliche Deutung der Gleichung von Schrödinger". Naturwissenschaften 14 (45): 1004–1004. Bibcode:1926NW.....14.1004M. doi:10.1007/BF01504657.
- ↑ Madelung, E. (1927). "Quantentheorie in hydrodynamischer Form". Z. Phys. 40 (3–4): 322–326. Bibcode:1927ZPhy...40..322M. doi:10.1007/BF01400372.
- ↑ I. Bialynicki-Birula, M. Cieplak, J. Kaminski (1992), Theory of Quanta, Oxford University Press, ISBN 0195071573
- ↑ Tsekov, R. (2012). "Bohmian Mechanics versus Madelung Quantum Hydrodynamics". doi:10.13140/RG.2.1.3663.8245.
Further reading
- Schönberg, M. (1954). "On the hydrodynamical model of the quantum mechanics". Il Nuovo Cimento 12 (1): 103–133. doi:10.1007/BF02820368.