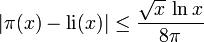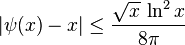Lowell Schoenfeld
| Lowell Schoenfeld | |
|---|---|
 | |
| Born | April 1, 1920 |
| Died | February 6, 2002 (aged 81) |
| Nationality |
|
| Fields | Mathematics |
| Alma mater | University of Pennsylvania |
| Doctoral advisor | Hans Rademacher |
| Doctoral students |
John Rowland Samuel Lawn |
Lowell Schoenfeld (April 1, 1920 – February 6, 2002) was an American mathematician known for his work in analytic number theory. He received his Ph.D. in 1944 from University of Pennsylvania under the direction of Hans Rademacher. He is known for obtaining the following results in 1976, assuming the Riemann hypothesis:
for all x ≥ 2657, based on the prime-counting function π(x) and the logarithmic integral function li(x), and
for all x ≥ 73.2, based on the second Chebyshev function ψ(x).[1]
His Erdős number is 2.
References
- ↑ ——— (1976), "Sharper Bounds for the Chebyshev Functions θ(x) and ψ(x). II", Mathematics of Computation 30 (134): 337–360, doi:10.2307/2005976.