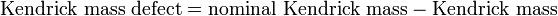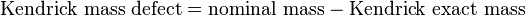Kendrick mass
The Kendrick mass is defined by setting the mass of a chosen molecular fragment, typically CH2, to an integer value in atomic mass units. It is different from the IUPAC definition, which is based on setting the mass of 12C isotope to exactly 12 u. The Kendrick mass is often used to identify homologous compounds differing only by a number of base units in high resolution mass spectra.[1][2] This definition of mass was first suggested in 1963 by chemist Edward Kendrick, and it has been adopted by scientists working in the area of high-resolution mass spectrometry, environmental analysis, proteomics, petroleomics, metabolomics, etc.
Definition
According to the procedure outlined by Kendrick, the mass of CH2 is defined as exactly 14 Da, instead of the IUPAC mass of 14.01565 Da.[3][4]
To convert an IUPAC mass of a particular compound to the Kendrick mass, the equation
-
 .
.
is used.[2][5][6][7] The mass in dalton units (Da) can be converted to the Kendrick scale by dividing by 1.0011178.[1][8]
Other groups of atoms in addition to CH2 can be used define the Kendrick mass, for example CO2, H2, H2O, and O.[7][9][10] In this case, the Kendrick mass for a family of compounds F is given by
 .
.
For the hydrocarbon analysis, F=CH2.
A recent publication has suggested that Kendrick mass be expressed in Kendrick units with symbol Ke.[11]
Kendrick mass defect
The Kendrick mass defect is defined as the exact Kendrick mass subtracted from the nominal (integer) Kendrick mass:[12][13]
In recent years the equation has changed due to rounding errors to:
The members of an alkylation series have the same degree of unsaturation and number of heteroatoms (nitrogen, oxygen and sulfur) but differ in the number of CH2 units. Members of an alkylation series have the same Kendrick mass defect.
The Kendrick mass defect has also been defined as
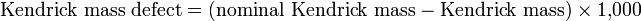 .[14]
.[14]
The abbreviations KM and KMD have been used for Kendrick mass and Kendrick mass defect, respectively. In some definitions, the KMD [15]
Kendrick mass analysis

In a Kendrick mass analysis, the Kendrick mass defect is plotted as function of nominal Kendrick mass for ions observed in a mass spectrum.[5] Ions of the same family, for example the members of an alkylation series, have the same Kendrick mass defect but different nominal Kendrick mass and are positioned along a horizontal line on the plot. If the composition of one ion in the family can be determined, the composition of the other ions can be inferred. Horizontal lines of different Kendrick mass defect correspond to ions of different composition, for example degree of saturation or heteroatom content.
A Kendrick mass analysis is often used in conjunction with a Van Krevelen diagram, a two- or three- dimensional graphical analysis in which the elemental composition of the compounds are plotted according to the atomic ratios H/C, O/C, or N/C.[7][16]
See also
Notes
- ↑ 1.0 1.1 Kendrick, Edward (1963), "A mass scale based on CH2 = 14.00000 for high resolution mass spectrometry of organic compounds", Anal. Chem. 35 (13): 2146–2154, doi:10.1021/ac60206a048.
- ↑ 2.0 2.1 Marshall AG, Rodgers RP (January 2004), "Petroleomics: the next grand challenge for chemical analysis", Acc. Chem. Res. 37 (1): 53–9, doi:10.1021/ar020177t, PMID 14730994.
- ↑ Mopper, Kenneth; Stubbins, Aron; Ritchie, Jason D.; Bialk, Heidi M.; Hatcher, Patrick G. (2007), "Advanced Instrumental Approaches for Characterization of Marine Dissolved Organic Matter: Extraction Techniques, Mass Spectrometry, and Nuclear Magnetic Resonance Spectroscopy", Chemical Reviews 107 (2): 419, doi:10.1021/cr050359b, PMID 17300139
- ↑ Meija, Juris (2006), "Mathematical tools in analytical mass spectrometry", Analytical and Bioanalytical Chemistry 385 (3): 486, doi:10.1007/s00216-006-0298-4, PMID 16514517
- ↑ 5.0 5.1 Headley, John V.; Peru, Kerry M.; Barrow, Mark P. (2009), "Mass spectrometric characterization of naphthenic acids in environmental samples: A review", Mass Spectrometry Reviews 28 (1): 121, doi:10.1002/mas.20185, PMID 18677766
- ↑ Ohta, Daisaku; Kanaya, Shigehiko; Suzuki, Hideyuki (2010), "Application of Fourier-transform ion cyclotron resonance mass spectrometry to metabolic profiling and metabolite identification", Current Opinion in Biotechnology 21 (1): 35, doi:10.1016/j.copbio.2010.01.012, PMID 20171870
- ↑ 7.0 7.1 7.2 Reemtsma, Thorsten (2009), "Determination of molecular formulas of natural organic matter molecules by (ultra-) high-resolution mass spectrometryStatus and needs", Journal of Chromatography A 1216 (18): 3687, doi:10.1016/j.chroma.2009.02.033, PMID 19264312
- ↑ Panda, Saroj K.; Andersson, Jan T.; Schrader, Wolfgang (2007), "Mass-spectrometric analysis of complex volatile and nonvolatile crude oil components: a challenge", Analytical and Bioanalytical Chemistry 389 (5): 1329, doi:10.1007/s00216-007-1583-6, PMID 17885749
- ↑ Kim, Sunghwan; Kramer, Robert W.; Hatcher, Patrick G. (2003), "Graphical Method for Analysis of Ultrahigh-Resolution Broadband Mass Spectra of Natural Organic Matter, the Van Krevelen Diagram", Analytical Chemistry 75 (20): 5336, doi:10.1021/ac034415p, PMID 14710810
- ↑ Nizkorodov, Sergey A.; Laskin, Julia; Laskin, Alexander (2011), "Molecular chemistry of organic aerosols through the application of high resolution mass spectrometry", Physical Chemistry Chemical Physics 13 (9): 3612, Bibcode:2011PCCP...13.3612N, doi:10.1039/C0CP02032J, PMID 21206953
- ↑ Junninen, H.; Ehn, M.; Petäjä, T.; Luosujärvi, L.; Kotiaho, T.; Kostiainen, R.; Rohner, U.; Gonin, M.; Fuhrer, K.; Kulmala, M.; Worsnop, D. R. (2010), "A high-resolution mass spectrometer to measure atmospheric ion composition", Atmospheric Measurement Techniques 3 (4): 1039, doi:10.5194/amt-3-1039-2010
- ↑ Hughey CA, Hendrickson CL, Rodgers RP, Marshall AG, Qian K (October 2001), "Kendrick mass defect spectrum: a compact visual analysis for ultrahigh-resolution broadband mass spectra", Anal. Chem. 73 (19): 4676–81, doi:10.1021/ac010560w, PMID 11605846.
- ↑ Marshall, A. G.; Rodgers, R. P. (2008), "Mass Spectrometry Special Feature: Petroleomics: Chemistry of the underworld", Proceedings of the National Academy of Sciences 105 (47): 18090, Bibcode:2008PNAS..10518090M, doi:10.1073/pnas.0805069105.
- ↑ Panda, Saroj K.; Andersson, Jan T.; Schrader, Wolfgang (2007), "Mass-spectrometric analysis of complex volatile and nonvolatile crude oil components: a challenge", Analytical and Bioanalytical Chemistry 389 (5): 1329, doi:10.1007/s00216-007-1583-6, PMID 17885749
- ↑ Reemtsma, Thorsten (2009), "Determination of molecular formulas of natural organic matter molecules by (ultra-) high-resolution mass spectrometry Status and needs", Journal of Chromatography A 1216 (18): 3687, doi:10.1016/j.chroma.2009.02.033, PMID 19264312
- ↑ Wu, Zhigang; Rodgers, Ryan P.; Marshall, Alan G. (2004), "Two- and Three-Dimensional van Krevelen Diagrams: A Graphical Analysis Complementary to the Kendrick Mass Plot for Sorting Elemental Compositions of Complex Organic Mixtures Based on Ultrahigh-Resolution Broadband Fourier Transform Ion Cyclotron Resonance Mass Measurements", Analytical Chemistry 76 (9): 2511, doi:10.1021/ac0355449, PMID 15117191