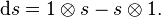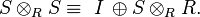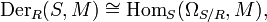Kähler differential

In mathematics, Kähler differentials provide an adaptation of differential forms to arbitrary commutative rings or schemes.

Presentation

The idea was introduced by Erich Kähler in the 1930s. It was adopted as standard, in commutative algebra and algebraic geometry, somewhat later, following the need to adapt methods from geometry over the complex numbers, and the free use of calculus methods, to contexts where such methods are not available.

Let R and S be commutative rings and φ:R → S a ring homomorphism. An important example is for R a field and S a unital algebra over R (such as the coordinate ring of an affine variety).

An R-linear derivation on S is a map 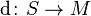$\mathrm d \colon S \to M$ to an S-module M with R in its kernel, satisfying Leibniz rule 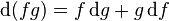$\mathrm d (fg) = f \mathrm \, \mathrm dg + g \, \mathrm df$ . The module of Kähler differentials is defined as the R-linear derivation $\mathrm d \colon S \to \Omega_{S/R}$ that factors all others.

Construction

The idea is now to give a universal construction of a derivation

d:S → Ω_S/R

over R, where Ω_S/R is an S-module, which is a purely algebraic analogue of the exterior derivative. This means that d is a homomorphism of R-modules such that

d(st) = s dt + t ds

for all s and t in S, and d is the best possible such derivation in the sense that any other derivation may be obtained from it by composition with an S-module homomorphism.

The actual construction of Ω_S/R and d can proceed by introducing formal generators ds for s in S, and imposing the relations

dr = 0 for r in R,
d(s + t) = ds + dt,
d(st) = s dt + t ds

for all s and t in S.

Another construction proceeds by letting I be the ideal in the tensor product 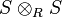$S \otimes_R S$ , defined as the kernel of the multiplication map: 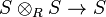$S \otimes_R S\to S$ , given by $\Sigma s_i \otimes t_i \mapsto \Sigma s_i.t_i$ . Then the module of Kähler differentials of "S" can be equivalently defined by^[1] Ω_S/R = I/I², together with the morphism

\mathrm ds = 1 \otimes s - s \otimes 1. \,

To see that this construction is equivalent to the previous one, note that I is the kernel of the projection 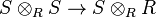$S \otimes_R S\to S \otimes_R R$ , given by 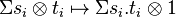$\Sigma s_i \otimes t_i \mapsto \Sigma s_i.t_i\otimes 1$ . Thus we have:

S \otimes_R S \equiv\,\,{} I \,{} \oplus S \otimes_R R.\,

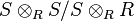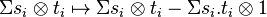Then $S \otimes_R S/ S\otimes_R R$ may be identified with I, by the map induced by the complementary projection which is given by $\Sigma s_i \otimes t_i \mapsto \Sigma s_i \otimes t_i-\Sigma s_i.t_i\otimes 1$ .

Thus this map identifies I with the S module generated by the formal generators ds for s in S, subject to the first two relations given above (with the second relation strengthened to demanding that d is R-linear). The elements set to zero by the final relation map to precisely I² in I.

Use in algebraic geometry

Geometrically, in terms of affine schemes, I represents the ideal defining the diagonal in the fiber product of Spec(S) with itself over Spec(S) → Spec(R). This construction therefore has a more geometric flavor, in the sense that the notion of first infinitesimal neighbourhood of the diagonal is thereby captured, via functions vanishing modulo functions vanishing at least to second order (see cotangent space for related notions).

For any S-module M, the universal property of Ω_S/R leads to a natural isomorphism

\operatorname{Der}_R(S,M)\cong \operatorname{Hom}_S(\Omega_{S/R},M), \,

where the left hand side is the S-module of all R-linear derivations from S to M. As in the case of adjoint functors (though this isn't an adjunction), this is more than just an isomorphism of modules; it commutes with S-module homomorphisms M → M' and hence is an isomorphism of functors.

To get Ω^p_S/R, the Kähler p-forms for p > 1, one takes the R-module exterior power of degree p. The behaviour of the construction under localization of a ring (applied to R and S) ensures that there is a geometric notion of sheaf of (relative) Kähler p-forms available for use in algebraic geometry.

Use in algebraic number theory

In algebraic number theory, the Kähler differentials may be used to study the ramification in an extension of algebraic number fields. If L/K is a finite extension with rings of integers O and o respectively then the different ideal δ_L/K, which encodes the ramification data, is the annihilator of the O-module Ω_O/o:^[2]

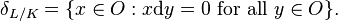
$\delta_{L/K} = \{ x \in O : x \mathrm{d} y = 0 \text{ for all } y \in O \} .$

References

↑ Hartshorne (1977) p.172
↑ Neukirch (1999) p.201

Johnson, J. (1969). "Kähler differentials and differential algebra". Annals of Mathematics 89: 92–98. doi:10.2307/1970810. Zbl 0179.34302.
Hartshorne, Robin (1977), Algebraic Geometry, Graduate Texts in Mathematics 52, New York: Springer-Verlag, ISBN 978-0-387-90244-9, MR 0463157
Matsumura, Hideyuki (1986). Commutative ring theory. Cambridge University Press.
Neukirch, Jürgen (1999), Algebraic Number Theory, Grundlehren der mathematischen Wissenschaften 322, Berlin: Springer-Verlag, ISBN 978-3-540-65399-8, Zbl 0956.11021, MR 1697859
Rosenlicht, M. (1976). "On Liouville's theory of elementary functions". Pacific J. Math. 65: 485–492. doi:10.2140/pjm.1976.65.485. Zbl 0318.12107.
Fu, G. et al. (2011). "Some remarks on Kähler differentials and ordinary differentials in nonlinear control systems". Systems and Control Letters 60: 699–703. doi:10.1016/j.sysconle.2011.05.006.

External links

A thread devoted to the question on MathOverflow