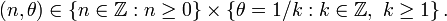Integer broom topology
In general topology, a branch of mathematics, the integer broom topology, is an example of a topology on the so-called integer broom space X.[1]
Definition of the integer broom space

The integer broom space X is a subset of the plane R2. Assume that the plane is parametrised by polar coordinates. The integer broom contains the origin and the points (n,θ) ∈ R2 such that n is a non-negative integer, and θ ∈ {1/k : k ∈ N and k ≥ 1}.[1] The image on the right gives an illustration for 0 ≤ n ≤ 5 and 1/15 ≤ θ ≤ 1. Geometrically, the space consists of a series of convergent sequences. For a fixed n, we have a sequence of points − lying on circle with centre (0,0) and radius n − that converges to the point (n,0).
Definition of the integer broom topology
We define a topology on X by means of a product topology. The Integer Broom space is given by the polar coordinates
Let us write (n,θ) ∈ U × V for simplicity. The Integer Broom topology on X is the product topology induced by giving U the right order topology, and V the subspace topology from R.[1]
Properties
The integer broom space, together with the integer broom topology, is a compact topological space. It is a so-called Kolmogorov space, but it is neither a Fréchet space nor a Hausdorff space. The space is locally connected and path connected, while not arc connected.[2]
See also
References
- ↑ 1.0 1.1 1.2 Steen, L. A.; Seebach, J. A. (1995), Counterexamples in Topology, Dover, p. 140, ISBN 0-486-68735-X
- ↑ Steen, L. A.; Seebach, J. A. (1995), Counterexamples in Topology, Dover, pp. 200–201, ISBN 0-486-68735-X