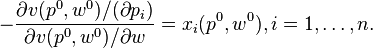Indirect utility function

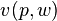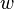In economics, a consumer's indirect utility function $v(p, w)$ gives the consumer's maximal attainable utility when faced with a vector $p$ of goods prices and an amount of income $w$ . It reflects both the consumer's preferences and market conditions.

This function is called indirect because consumers usually think about their preferences in terms of what they consume rather than prices. A consumer's indirect utility 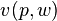$v(p, w)$ can be computed from his or her utility function 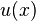$u(x)$ by first computing the most preferred bundle, represented by the vector 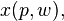$x(p, w),$ by solving the utility maximization problem, and second, computing the utility 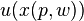$u(x(p, w))$ the consumer derives from that bundle.

Formally, the indirect utility function is:

Continuous on Rⁿ₊₊+ R₊;
Decreasing in prices;
Strictly increasing in income;
Homogenous with degree zero in prices and income; if prices and income are all multiplied by a given constant the same bundle of consumption represents a maximum, so optimal utility does not change.
quasi convex in (p,w);

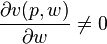Moreover, Roy's identity states that if v(p,w) is differentiable at $(p^0, w^0)$ and $\frac{\partial v(p,w)}{\partial w} \neq 0$ , then

-\frac{\partial v(p^0,w^0)/(\partial p_i)}{\partial v(p^0,w^0)/\partial w}=x_i (p^0,w^0), i=1, \dots, n.

References

Mas-Colell, Andreu; Whinston, Michael D.; Green, Jerry R. (2007). Microeconomic Theory (Indian ed.). pp. 56–57.
Jehle, G. A.; Reny, P. J. (2011). Advanced Microeconomic Theory (Third ed.). Prentice Hall. pp. 28–33.
Nicholson, Walter (1978). Microeconomic Theory: Basic Principles and Extensions (Second ed.). Hinsdale: Dryden Press. pp. 57–59. ISBN 0-03-020831-9.

Indirect utility function

See also

References