Huber's equation

Huber's equation, first derived by a Polish engineer Tytus Maksymilian Huber, is a basic formula in elastic material tension calculations, an equivalent of the equation of state, but applying to solids. In most simple expression and commonly in use it looks like this:

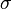$\sigma_{red}=\sqrt{({\sigma}^2) + 3({\tau}^2)}$

where $\sigma$ is the tensile stress, and 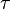$\tau$ is the shear stress, measured in newtons per square meter (N/m², also called pascals, Pa), while 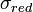$\sigma_{red}$ - called a reduced tension, is the resultant tension of the material.

Very useful in calculating the span width of the bridges like Golden Gate Bridge or Verrazano-Narrows Bridge, their beam cross-sections, etc.

Huber's equation

See also