Horizon problem

This article is about the astronomical "horizon problem". For the problem relating to artificial intelligence, see Horizon effect.
When we look at the CMB it comes from 46 billion comoving light years away. However when the light was emitted the universe was much younger (300,000 years old). In that time light would have only reached as far as the smaller circles. The two points indicated on the diagram would not have been able to contact each other because their spheres of causality do not overlap.

The horizon problem is a problem with the standard cosmological model of the Big Bang which was identified in the late 1960s, primarily by Charles Misner. It points out that different regions of the universe have not "contacted" each other because of the great distances between them, but nevertheless they have the same temperature and other physical properties. This should not be possible, given that the transfer of information (or energy, heat, etc.) can occur, at most, at the speed of light.

One theory that attempts to solve the horizon problem is the theory of cosmic inflation. However, a recurrent criticism of inflation is that the invoked inflation field does not correspond to any known physical field, and that its potential energy curve seems to be an ad hoc contrivance to accommodate almost any data obtainable. Paul J. Steinhardt, one of the founding fathers of inflationary cosmology, has recently become one of its sharpest critics.

Basic concept

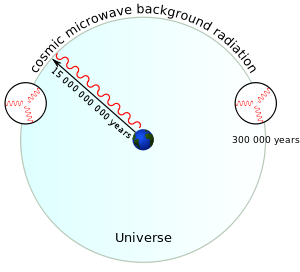
When one looks out into the night sky, distances also correspond to time into the past. A galaxy measured at ten billion light years in distance appears to us as it was ten billion years ago, because the light has taken that long to travel to the viewer. If one were to look at a galaxy ten billion light years away in one direction, say "west", and another in the opposite direction, "east", the total distance between them is twenty billion light years. This means that the light from the first has not yet reached the second, because the 13.8 billion years that the universe has existed simply is not a long enough time to allow it to occur. In a more general sense, there are portions of the universe that are visible to us, but invisible to each other, outside each other's respective particle horizons.

In standard physical theories, no information can travel faster than the speed of light. In this context, "information" means "any sort of physical interaction". For instance, heat will naturally flow from a hotter area to a cooler one, and in physics terms this is one example of information exchange. Given the example above, the two galaxies in question cannot have shared any sort of information; they are not in "causal contact". One would expect, then, that their physical properties would be different, and more generally, that the universe as a whole would have varying properties in different areas.

Contrary to this expectation, the universe is in fact extremely isotropic, which also implies homogeneity. The cosmic microwave background radiation (CMB), which fills the universe, is almost precisely the same temperature everywhere in the sky, about 2.728 +/- 0.004  K. The differences in temperature are so slight that it has only recently become possible to develop instruments capable of making the required measurements. This presents a serious problem; if the universe had started with even slightly different temperatures in different areas, then there would simply be no way it could have evened itself out to a common temperature by this point in time.

According to the Big Bang model, as the density of the universe dropped (while it expanded) it eventually reached a point where photons in the "mix" of particles were no longer immediately impacting matter; they "decoupled" from the plasma and spread out into the universe as a burst of light. This is thought to have occurred about 300,000 years after the Big Bang. The volume of any possible information exchange at that time was 900,000 light years across, using the speed of light and the rate of expansion of space in the early universe. Instead, the entire sky has the same temperature, a volume 1088 times larger.

Inflation

The theory of cosmic inflation has attempted to solve the problem[1] (along with several other problems such as the flatness problem) by postulating a short 10−32 second period of exponential expansion (dubbed "inflation") in the first seconds of the history of the universe. During inflation, the universe would have increased in size by an enormous factor. Prior to the inflation the entire universe was small and causally connected; it was during this period that the physical properties evened out. Inflation then expanded the universe rapidly, "locking in" the uniformity at large distances.

One consequence of cosmic inflation is that the anisotropies in the Big Bang are reduced but not entirely eliminated. Differences in the temperature of the cosmic background are smoothed by cosmic inflation, but they still exist. The theory predicts a spectrum for the anisotropies in the microwave background which is mostly[2] consistent with observations from WMAP and COBE.

However, in order to work, and as pointed out by Roger Penrose from 1986 on, inflation requires extremely specific initial conditions of its own, so that the problem (or pseudo-problem) of initial conditions is not solved: "There is something fundamentally misconceived about trying to explain the uniformity of the early universe as resulting from a thermalization process. [...] For, if the thermalization is actually doing anything [...] then it represents a definite increasing of the entropy. Thus, the universe would have been even more special before the thermalization than after."[3]

Variable speed of light theories

The varying speed of light cosmology has been proposed independently by Jean-Pierre Petit in 1988,[4][5][6][7] John Moffat in 1992,[8] and the two-man team of Andreas Albrecht and João Magueijo in 1998[9][10][11][12][13][14] to explain the horizon problem of cosmology and propose an alternative to cosmic inflation. An alternative VSL model has also been proposed.[15]

In Petit's VSL model, the variation of c accompanies the joint variations of all physical constants combined to space and time scale factors changes, so that all equations and measurements of these constants remain unchanged through the evolution of the universe. The Einstein field equations remain invariant through convenient joint variations of c and G in Einstein's constant. According to this model, the cosmological horizon grows like R, the space scale, which ensures the homogeneity of the primeval universe, which fits the observational data. Late-model restricts the variation of constants to the higher energy density of the early universe, at the very beginning of the radiation-dominated era where spacetime is identified to space-entropy with a metric conformally flat.[16][17]

The idea from Moffat and the team Albrecht–Magueijo is that light propagated as much as 60 orders of magnitude faster in the early universe, thus distant regions of the expanding universe have had time to interact at the beginning of the universe. There is no known way to solve the horizon problem with variation of the fine-structure constant, because its variation does not change the causal structure of spacetime. To do so would require modifying gravity by varying Newton's constant or redefining special relativity . Classically, varying speed of light cosmologies propose to circumvent this by varying the dimensionful quantity c by breaking the Lorentz invariance of Einstein's theories of general and special relativity in a particular way.[18][19] More modern formulations preserve local Lorentz invariance.[11]

See also

References

  1. ↑ An Exposition on Inflationary Cosmology, Gary Scott Watson, Dept. of Physics, Brown University
  2. ↑ Starkman, Glenn D. and Dominic J. Schwarz; Scientific American (subscription required)
  3. ↑ Penrose, Roger (2004). The Road to Reality: A Complete Guide to the Laws of the Universe. London: Vintage Books, p. 755. See also Penrose, Roger (1989). "Difficulties with Inflationary Cosmology". Annals of the New York Academy of Sciences 271: 249–264. Bibcode:1989NYASA.571..249P. doi:10.1111/j.1749-6632.1989.tb50513.x
  4. ↑ J.P. Petit (1988). "An interpretation of cosmological model with variable light velocity" (PDF). Mod. Phys. Lett. A 3 (16): 1527–1532. Bibcode:1988MPLA....3.1527P. doi:10.1142/S0217732388001823.
  5. ↑ J.P. Petit (1988). "Cosmological model with variable light velocity: the interpretation of red shifts" (PDF). Mod. Phys. Lett. A 3 (18): 1733–1744. Bibcode:1988MPLA....3.1733P. doi:10.1142/S0217732388002099.
  6. ↑ J.P. Petit, M. Viton (1989). "Gauge cosmological model with variable light velocity. Comparizon with QSO observational data" (PDF). Mod. Phys. Lett. A 4 (23): 2201–2210. Bibcode:1989MPLA....4.2201P. doi:10.1142/S0217732389002471.
  7. ↑ P. Midy, J.P. Petit (1989). "Scale invariant cosmology" (PDF). Int. J. Mod. Phys. D (8): 271–280.
  8. ↑ J. Moffat (1993). "Superluminary Universe: A Possible Solution to the Initial Value Problem in Cosmology". Int. J. Mod. Phys. D 2 (3): 351–366. arXiv:gr-qc/9211020. Bibcode:1993IJMPD...2..351M. doi:10.1142/S0218271893000246.
  9. ↑ J.D. Barrow (1998). "Cosmologies with varying light-speed". Physical Review D 59 (4). arXiv:astro-ph/9811022. Bibcode:1999PhRvD..59d3515B. doi:10.1103/PhysRevD.59.043515.
  10. ↑ A. Albrecht, J. Magueijo (1999). "A time varying speed of light as a solution to cosmological puzzles". Phys. Rev. D59: 043516. arXiv:astro-ph/9811018. Bibcode:1999PhRvD..59d3516A. doi:10.1103/PhysRevD.59.043516.
  11. ↑ 11.0 11.1 J. Magueijo (2000). "Covariant and locally Lorentz-invariant varying speed of light theories". Phys. Rev. D62: 103521. arXiv:gr-qc/0007036. Bibcode:2000PhRvD..62j3521M. doi:10.1103/PhysRevD.62.103521.
  12. ↑ J. Magueijo (2001). "Stars and black holes in varying speed of light theories". Phys. Rev. D63: 043502. arXiv:astro-ph/0010591. Bibcode:2001PhRvD..63d3502M. doi:10.1103/PhysRevD.63.043502.
  13. ↑ J. Magueijo (2003). "New varying speed of light theories". Rept. Prog. Phys. 66 (11): 2025. arXiv:astro-ph/0305457. Bibcode:2003RPPh...66.2025M. doi:10.1088/0034-4885/66/11/R04.
  14. ↑ J. Magueijo (2003). Faster Than the Speed of Light: The Story of a Scientific Speculation. Massachusetts: Perseus Books Group. ISBN 0-7382-0525-7.
  15. ↑ J. Casado (2003). "A Simple Cosmological Model with Decreasing Light Speed". arXiv:astro-ph/0310178 [astro-ph].
  16. ↑ J.P. Petit, P. Midy, F. Landsheat (2001). "Twin matter against dark matter" (PDF). "Where is the matter?" (See sections 14 and 15 pp. 21–26). Int. Conf. on Astr. & Cosm.
  17. ↑ J.P Petit, G. d'Agostini (2007). "Bigravity: a bimetric model of the Universe with variable constants, including VSL (variable speed of light)". arXiv:0803.1362 [physics.gen-ph].
  18. ↑ M. A. Clayton, J. W. Moffat (1999). "Dynamical Mechanism for Varying Light Velocity as a Solution to Cosmological Problems". Phys. Lett. B460: 263–270. arXiv:astro-ph/9812481. Bibcode:1999PhLB..460..263C. doi:10.1016/S0370-2693(99)00774-1.
  19. ↑ B.A. Bassett, S. Liberati, C. Molina-Paris, M. Visser (2000). "Geometrodynamics of variable-speed-of-light cosmologies". Phys. Rev. D62: 103518. arXiv:astro-ph/0001441. Bibcode:2000PhRvD..62j3518B. doi:10.1103/PhysRevD.62.103518.