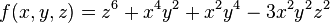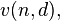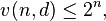Hilbert's seventeenth problem
Hilbert's seventeenth problem is one of the 23 Hilbert problems set out in a celebrated list compiled in 1900 by David Hilbert. It concerns the expression of positive definite rational functions as sums of quotients of squares. The original question may be stated as:
- Given a multivariate polynomial that takes only non-negative values over the reals, can it be represented as a sum of squares of rational functions?
This was solved in the affirmative, in 1927, by Emil Artin, for positive definite functions over the reals or more generally real-closed fields. An algorithmic solution was found by Charles Delzell in 1984.[1] A result of Albrecht Pfister[2] shows that a positive semidefinite form in n variables can be expressed as a sum of 2n squares.[3]
Dubois showed in 1967 that the answer is negative in general for ordered fields.[4] In this case one can say that a positive polynomial is a sum of weighted squares of rational functions with positive coefficients.[5]
A generalization to the matrix case (matrices with rational function entries that are always positive semidefinite are sums of symmetric squares) was given by Gondard, Ribenboim[6] and Procesi, Schacher,[7] with an elementary proof given by Hillar and Nie.[8]
The formulation of the question takes into account that there are polynomials, for example[9]
which are non-negative over reals and yet which cannot be represented as a sum of squares of other polynomials, as Hilbert had shown in 1888 but without giving an example: the first explicit example was found by Motzkin in 1966.
Explicit sufficient conditions for a polynomial to be a sum of squares of other polynomials have been found.[10][11] However every real nonnegative polynomial can be approximated as closely as desired (in the 
-norm of its coefficient vector) by a sequence of polynomials that are sums of squares of polynomials.[12]
It is an open question what is the smallest number
such that any n-variate, non-negative polynomial of degree d can be written as sum of at most 
square rational functions over the reals.
The best known result (as of 2008) is
due to Pfister in 1967.[2]
In complex analysis the Hermitian analogue, requiring the squares to be squared norms of holomorphic mappings, is somewhat more complicated, but true for positive polynomials by a result of Quillen.[13] The result of Pfister on the other hand fails in the Hermitian case, that is there is no bound on the number of squares required, see D'Angelo–Lebl.[14]
See also
References
- ↑ Delzell, C.N. (1984). "A continuous, constructive solution to Hilbert's 17th problem". Inventiones Mathematicae 76: 365–384. doi:10.1007/BF01388465. Zbl 0547.12017.
- ↑ 2.0 2.1 Pfister, Albrecht (1967). "Zur Darstellung definiter Funktionen als Summe von Quadraten". Inventiones Mathematicae (in German) 4: 229–237. doi:10.1007/bf01425382. Zbl 0222.10022.
- ↑ Lam (2005) p.391
- ↑ Dubois, D.W. (1967). "Note on Artin's solution of Hilbert's 17th problem". Bull. Am. Math. Soc. 73: 540–541. doi:10.1090/s0002-9904-1967-11736-1. Zbl 0164.04502.
- ↑ Lorenz (2008) p.16
- ↑ Gondard, Danielle; Ribenboim, Paulo (1974). "Le 17e problème de Hilbert pour les matrices". Bull. Sci. Math. (2) 98 (1): 49–56. MR 432613. Zbl 0298.12104.
- ↑ Procesi, Claudio; Schacher, Murray (1976). "A non-commutative real Nullstellensatz and Hilbert's 17th problem". Ann. of Math. (2) 104 (3): 395–406. doi:10.2307/1970962. MR 432612. Zbl 0347.16010.
- ↑ Hillar, Christopher J.; Nie, Jiawang (2008). "An elementary and constructive solution to Hilbert's 17th problem for matrices". Proc. Am. Math. Soc. 136 (1): 73–76. arXiv:math/0610388. doi:10.1090/s0002-9939-07-09068-5. Zbl 1126.12001.
- ↑ Marie-Françoise Roy. The role of Hilbert's problems in real algebraic geometry. Proceedings of the ninth EWM Meeting, Loccum, Germany 1999
- ↑ Lasserre, Jean B. (2007). "Sufficient conditions for a real polynomial to be a sum of squares". Arch. Math. 89 (5): 390–398. doi:10.1007/s00013-007-2251-y. Zbl 1149.11018.
- ↑
- ↑ Lasserre, Jean B. (2007). "A sum of squares approximation of nonnegative polynomials". SIAM Rev. 49 (4): 651–669. doi:10.1137/070693709. ISSN 0036-1445. Zbl 1129.12004.
- ↑ Quillen, Daniel G. (1968). "On the representation of hermitian forms as sums of squares". Invent. Math. 5: 237–242. doi:10.1007/bf01389773. Zbl 0198.35205.
- ↑ D'Angelo, John P.; Lebl, Jiri (2012). "Pfister's theorem fails in the Hermitian case". Proc. Am. Math. Soc. 140 (4): 1151–1157. arXiv:1010.3215. doi:10.1090/s0002-9939-2011-10841-4. Zbl 06028329.
- Pfister, Albrecht (1976). "Hilbert's seventeenth problem and related problems on definite forms". In Felix E. Browder. Mathematical Developments Arising from Hilbert Problems. Proceedings of Symposia in Pure Mathematics. XXVIII.2. American Mathematical Society. pp. 483–489. ISBN 0-8218-1428-1.
- Lam, Tsit-Yuen (2005). Introduction to Quadratic Forms over Fields. Graduate Studies in Mathematics 67. American Mathematical Society. ISBN 0-8218-1095-2. Zbl 1068.11023.
- Lorenz, Falko (2008). Algebra. Volume II: Fields with Structure, Algebras and Advanced Topics. Springer-Verlag. pp. 15–27. ISBN 978-0-387-72487-4. Zbl 1130.12001.
- Rajwade, A. R. (1993). Squares. London Mathematical Society Lecture Note Series 171. Cambridge University Press. ISBN 0-521-42668-5. Zbl 0785.11022.