Generated collection

In diatonic set theory, a generated collection is a collection or scale formed by repeatedly adding a constant interval in integer notation, the generator, also known as an interval cycle, around the chromatic circle until a complete collection or scale is formed. All scales which have the deep scale property may be generated by any interval coprime with (in twelve-tone equal temperament) twelve. (Johnson 2003, p.83)

The C major diatonic collection may be generated by adding a cycle of perfect fifths (C7) starting at F: F-C-G-D-A-E-B = C-D-E-F-G-A-B. Using integer notation and modulo 12: 5 + 7 = 0, 0 + 7 = 7, 7 + 7 = 2, 2 + 7 = 9, 9 + 7 = 4, 4 + 7 = 11.

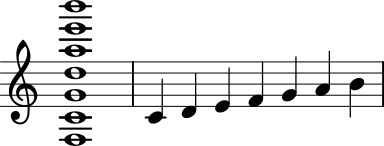
The C major scale could also be generated using cycle of perfect fourths (C5), as 12 minus any coprime of twelve is also coprime with twelve: 12 − 7 = 5. B-E-A-D-G-C-F.

A generated collection for which a single generic interval corresponds to the single generator or interval cycle used is a MOS (for "moment of symmetry") or well formed generated collection. For example, the diatonic collection is well formed, for the perfect fifth (the generic interval 4) corresponds to the generator 7. Though not all fifths in the diatonic collection are perfect (B-F is a diminished fifth, tritone, or 6), a well formed generated collection will have only one specific interval between scale members (in this case 6) which corresponds to the generic interval (4, a fifth) but to not the generator (7) and this one differing interval will always be the generator (7) plus or minus one (7 − 1 = 6) if the total number of specific intervals (12) and the generic interval's corresponding specific interval (7) are coprime (12 and 7 are). The pentatonic scale is also well formed. (ibid)

The properties of generated and well-formedness were described by Norman Carey and David Clampitt in "Aspects of Well-Formed Scales" (1989), (ibid, p. 151.) In earlier (1975) work, non-academic theoretician Erv Wilson considered the idea, and called such a scale a MOS, an acronym for "Moment of Symmetry". While unpublished, this terminology became widely known and used in the microtonal music community.

A degenerate well-formed collection are scales in which the generator and the interval required to complete the circle or return to the initial note are equivalent and include all scales with equal notes, such as the whole-tone scale. (ibid, p.158n14)

A bisector is a weaker substitute used to create collections which may not be generated.

See also

• distance model
• circle of fifths
• Pythagorean tuning

External links

• Handwritten letter of Erv Wilson

References

• Carey, Norman and Clampitt, David (1989). "Aspects of Well-Formed Scales", Music Theory Spectrum 11: 187–206.
• Clough, Engebretsen, and Kochavi. "Scales, Sets, and Interval Cycles", 79.
• Johnson, Timothy (2003). Foundations of Diatonic Theory: A Mathematically Based Approach to Music Fundamentals. Key College Publishing. ISBN 1-930190-80-8.

Musical set theory All-interval tetrachord Complement Forte number Identity Interval class Interval vector Multiplication Permutation Pitch class Pitch interval Pitch-interval class Set List Similarity relation Transformation Z-relation Diatonic set theory Bisector Cardinality equals variety Common tone Diatonic scale Deep scale property Generated collection Generic interval Maximal evenness Myhill's property Rothenberg propriety Specific interval Structure implies multiplicity