General selection model

The General Selection Model (GSM) is a model of population genetics that describes how a population's allele frequencies will change when acted upon by natural selection.

Equation

The General Selection Model applied to a single gene with two alleles (let's call them A1 and A2) is encapsulated by the equation:

$\Delta q=\frac{pq \big[q(W_2-W_1) + p(W_1 - W_0)\big ]}{\overline{W}}$

where:

p

is the frequency of allele A1

q

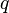is the frequency of allele A2

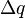\Delta q

is the rate of evolutionary change of the frequency of allele A2

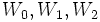W_0,W_1, W_2

are the relative fitnesses of homozygous A1, heterozygous (A1A2), and homozygous A2 genotypes respectively.

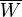\overline{W}

is the mean population relative fitness.

In words:

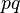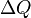The product of the relative frequencies, $pq$ , is a measure of the genetic variance. The quantity pq is maximized when there is an equal frequency of each gene, when $p=q$ . In the GSM, the rate of change $\Delta Q$ is proportional to the genetic variation.

The mean population fitness 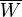$\overline{W}$ is a measure of the overall fitness of the population. In the GSM, the rate of change 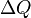$\Delta Q$ is inversely proportional to the mean fitness 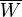$\overline{W}$ —i.e. when the population is maximally fit, no further change can occur.

The remainder of the equation, $\big[q(W_2-W_1) + p(W_1 - W_0)\big ]$ , refers to the mean effect of an allele substitution. In essence, this term quantifies what effect genetic changes will have on fitness.

General selection model

Equation

See also