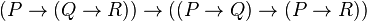Frege's theorem
In metalogic and metamathematics, Frege's theorem is a metatheorem that states that the Peano axioms of arithmetic can be derived in second-order logic from Hume's principle. It was first proven, informally, by Gottlob Frege in his Die Grundlagen der Arithmetik (Foundations of Arithmetic), published in 1884, and proven more formally in his Grundgesetze der Arithmetik (Basic Laws of Arithmetic), published in two volumes, in 1893 and 1903. The theorem was re-discovered by Crispin Wright in the early 1980s and has since been the focus of significant work. It is at the core of the philosophy of mathematics known as neo-logicism.
Frege's theorem in propositional logic
In propositional logic, Frege's theorems refers to this tautology:
References
- Zalta, Edward (2013), "Frege's Theorem and Foundations for Arithmetic", Stanford Encyclopedia of Philosophy.