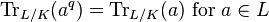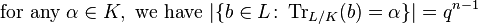Field trace
- For other uses, see Trace
In mathematics, the field trace is a particular function defined with respect to a finite field extension L/K, which is a K-linear map from L to K.
Definition
Let K be a field and L a finite extension (and hence an algebraic extension) of K. L can be viewed as a vector space over K. Multiplication by α, an element of L,
 ,
,
is a K-linear transformation of this vector space into itself. The trace, TrL/K(α), is defined as the (linear algebra) trace of this linear transformation.[1]
For α in L, let σ1(α), ..., σn(α) be the roots (counted with multiplicity) of the minimal polynomial of α over K (in some extension field of L), then
![\operatorname{Tr}_{L/K}(\alpha)=[L:K(\alpha)]\sum_{j=1}^n\sigma_j(\alpha)](../I/m/167daa50fb8fcacf7548edd1172ff818.png) .
.
If L/K is separable then each root appears only once and the coefficient above is one.[2]
More particularly, if L/K is a Galois extension and α is in L, then the trace of α is the sum of all the Galois conjugates of α, i.e.
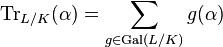 ,
,
where Gal(L/K) denotes the Galois group of L/K.
Example
Let 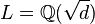 be a quadratic extension of
be a quadratic extension of 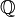 . Then a basis of
. Then a basis of  If
If  then the matrix of
then the matrix of 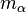 is:
is:
![\left [ \begin{matrix} a & bd \\ b & a \end{matrix} \right ]](../I/m/c02825ca913f5093ae62f77a9ca1d26b.png) ,
,
and so, 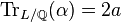 .[1] The minimal polynomial of α is X2 - 2a X + a2 - d b2.
.[1] The minimal polynomial of α is X2 - 2a X + a2 - d b2.
Properties of the trace
Several properties of the trace function hold for any finite extension.[3]
The trace TrL/K : L → K is a K-linear map (a K-linear functional), that is
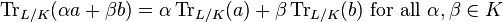 .
.
If α ∈ K then ![\operatorname{Tr}_{L/K}(\alpha) = [L:K] \alpha.](../I/m/476682fb82de0ed9946b4284b685d6cf.png)
Additionally, trace behaves well in towers of fields: if M is a finite extension of L, then the trace from M to K is just the composition of the trace from M to L with the trace from L to K, i.e.
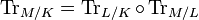 .
.
Finite fields
Let L = GF(qn) be a finite extension of a finite field K = GF(q). Since L/K is a Galois extension, if α is in L, then the trace of α is the sum of all the Galois conjugates of α, i.e.[4]
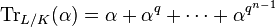 .
.
In this setting we have the additional properties,[5]
And,[6]
Theorem. For b ∈ L, let Fb be the map 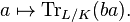 Then Fb ≠ Fc if b ≠ c. Moreover the K-linear transformations from L to K are exactly the maps of the form Fb as b varies over the field L.
Then Fb ≠ Fc if b ≠ c. Moreover the K-linear transformations from L to K are exactly the maps of the form Fb as b varies over the field L.
When K is the prime subfield of L, the trace is called the absolute trace and otherwise it is a relative trace.[4]
Application
A quadratic equation, 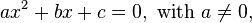 and coefficients in the finite field
and coefficients in the finite field 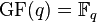 has either 0, 1 or 2 roots in GF(q) (and two roots, counted with multiplicity, in the quadratic extension GF(q2)). If the characteristic of GF(q) is odd, the discriminant, Δ = b2 - 4ac indicates the number of roots in GF(q) and the classical quadratic formula gives the roots. However, when GF(q) has even characteristic (i.e., q = 2h for some positive integer h), these formulas are no longer applicable.
has either 0, 1 or 2 roots in GF(q) (and two roots, counted with multiplicity, in the quadratic extension GF(q2)). If the characteristic of GF(q) is odd, the discriminant, Δ = b2 - 4ac indicates the number of roots in GF(q) and the classical quadratic formula gives the roots. However, when GF(q) has even characteristic (i.e., q = 2h for some positive integer h), these formulas are no longer applicable.
Consider the quadratic equation ax2 + bx + c = 0 with coefficients in the finite field GF(2h).[7] If b = 0 then this equation has the unique solution 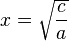 in GF(q). If b ≠ 0 then the substitution y = ax/b converts the quadratic equation to the form:
in GF(q). If b ≠ 0 then the substitution y = ax/b converts the quadratic equation to the form:
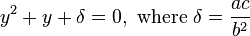 .
.
This equation has two solutions in GF(q) if and only if the absolute trace  In this case, if y = s is one of the solutions, then y = s + 1 is the other. Let k be any element of GF(q) with
In this case, if y = s is one of the solutions, then y = s + 1 is the other. Let k be any element of GF(q) with  Then a solution to the equation is given by:
Then a solution to the equation is given by:
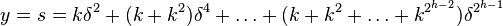 .
.
When h = 2m + 1, a solution is given by the simpler expression:
 .
.
Trace form
When L/K is separable, the trace provides a duality theory via the trace form: the map from L × L to K sending (x, y) to TrL/K(xy) is a nondegenerate, symmetric, bilinear form called the trace form. An example of where this is used is in algebraic number theory in the theory of the different ideal.
The trace form for a finite degree field extension L/K has non-negative signature for any field ordering of K.[8] The converse, that every Witt equivalence class with non-negative signature contains a trace form, is true for algebraic number fields K.[8]
If L/K is an inseparable extension, then the trace form is identically 0.[9]
See also
- Field norm
- Reduced trace
Notes
- ↑ 1.0 1.1 Rotman 2002, p. 940
- ↑ Rotman 2002, p. 941
- ↑ Roman 1995, p. 151 (1st ed.)
- ↑ 4.0 4.1 Lidl & Niederreiter 1997, p.54
- ↑ Mullen & Panario 2013, p. 21
- ↑ Lidl & Niederreiter 1997, p.56
- ↑ Hirschfeld 1979, pp. 3-4
- ↑ 8.0 8.1 Lorenz (2008) p.38
- ↑ Isaacs 1994, p. 369 as footnoted in Rotman 2002, p. 943
References
- Hirschfeld, J.W.P. (1979), Projective Geometries over Finite Fields, Oxford Mathematical Monographs, Oxford University Press, ISBN 0-19-853526-0
- Isaacs, I.M. (1994), Algebra, A Graduate Course, Brooks/Cole Publishing
- Lidl, Rudolf; Niederreiter, Harald (1997) [1983], Finite Fields, Encyclopedia of Mathematics and its Applications 20 (Second ed.), Cambridge University Press, ISBN 0-521-39231-4, Zbl 0866.11069
- Lorenz, Falko (2008). Algebra. Volume II: Fields with Structure, Algebras and Advanced Topics. Springer. ISBN 978-0-387-72487-4. Zbl 1130.12001.
- Mullen, Gary L.; Panario, Daniel (2013), Handbook of Finite Fields, CRC Press, ISBN 978-1-4398-7378-6
- Roman, Steven (2006), Field theory, Graduate Texts in Mathematics 158 (Second ed.), Springer, Chapter 8, ISBN 978-0-387-27677-9, Zbl 1172.12001
- Rotman, Joseph J. (2002), Advanced Modern Algebra, Prentice Hall, ISBN 978-0-13-087868-7
Further reading
- Conner, P.E.; Perlis, R. (1984). A Survey of Trace Forms of Algebraic Number Fields. Series in Pure Mathematics 2. World Scientific. ISBN 9971-966-05-0. Zbl 0551.10017.
- Section VI.5 of Lang, Serge (2002), Algebra, Graduate Texts in Mathematics 211 (Revised third ed.), New York: Springer-Verlag, ISBN 978-0-387-95385-4, Zbl 0984.00001, MR 1878556