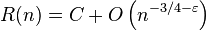Erdős–Fuchs theorem
In mathematics, in the area of combinatorial number theory, the Erdős–Fuchs theorem is a statement about the number of ways that numbers can be represented as a sum of two elements of a given set, stating that the average order of this number cannot be close to being a linear function.
The theorem is named after Paul Erdős and Wolfgang Heinrich Johannes Fuchs.
Statement
Let A be a subset of the natural numbers and r(n) denote the number of ways that a natural number n can be expressed as the sum of two elements of A (taking order into account). We consider the average
The theorem states that
cannot hold unless C = 0.
References
- P. Erdős; W.H.J. Fuchs (1956). "On a Problem of Additive Number Theory". Journal of the London Mathematical Society 31 (1): 67–73. doi:10.1112/jlms/s1-31.1.67.
- Donald J. Newman (1998). Analytic number theory. GTM 177. New York: Springer. pp. 31–38. ISBN 0-387-98308-2.