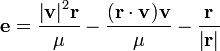Eccentricity vector

In celestial mechanics, the eccentricity vector of a Kepler orbit is the vector that points towards the periapsis and has a magnitude equal to the orbit's scalar eccentricity. The magnitude is unitless. For Kepler orbits the eccentricity vector is a constant of motion. Its main use is in the analysis of almost circular orbits, as perturbing (non-Keplerian) forces on an actual orbit will cause the osculating eccentricity vector to change continuously. For the eccentricity and argument of periapsis parameters, eccentricity zero (circular orbit) corresponds to a singularity.

Calculation

The eccentricity vector $\mathbf{e} \,$ can be calculated^[1]

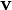\mathbf{e} = {\mathbf{v}\times\mathbf{h}\over{\mu}} - {\mathbf{r}\over{\left|\mathbf{r}\right|}}

where:

$\mathbf{v}\,\!$ is velocity vector
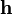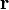$\mathbf{h}\,\!$ is specific angular momentum vector (equal to $\mathbf{r}\times\mathbf{v}$ )
$\mathbf{r}\,\!$ is position vector
$\mu\,\!$ is standard gravitational parameter

or equivalently:

\mathbf{e} = {\mathbf{\left |v \right |}^2 \mathbf{r} \over {\mu}} - {(\mathbf{r} \cdot \mathbf{v} ) \mathbf{v} \over{\mu}} - {\mathbf{r}\over{\left|\mathbf{r}\right|}}

References

↑ Cordani, Bruno (2003). The Kepler Problem. Birkhaeuser. p. 22. ISBN 3-7643-6902-7.

Eccentricity vector

Calculation

See also

References