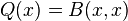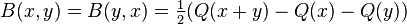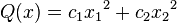Definite quadratic form
In mathematics, a definite quadratic form is a quadratic form over some real vector space V that has the same sign (always positive or always negative) for every nonzero vector of V. According to that sign, the quadratic form is called positive definite or negative definite.
A semidefinite (or semi-definite) quadratic form is defined in the same way, except that "positive" and "negative" are replaced by "not negative" and "not positive", respectively. An indefinite quadratic form is one that takes on both positive and negative values.
More generally, the definition applies to a vector space over an ordered field.[1]
Associated symmetric bilinear form
Quadratic forms correspond one-to-one to symmetric bilinear forms over the same space.[2] A symmetric bilinear form is also described as definite, semidefinite, etc. according to its associated quadratic form. A quadratic form Q and its associated symmetric bilinear form B are related by the following equations:
Example
As an example, let 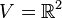 , and consider the quadratic form
, and consider the quadratic form
where x = (x1, x2) and c1 and c2 are constants. If c1 > 0 and c2 > 0, the quadratic form Q is positive definite. If one of the constants is positive and the other is zero, then Q is positive semidefinite. If c1 > 0 and c2 < 0, then Q is indefinite.
See also
- Anisotropic quadratic form
- Positive definite function
- Positive definite matrix
References
- ↑ Milnor & Husemoller (1973) p.61
- ↑ This is true only over a field of characteristic different of 2, but here we consider only ordered fields which necessarily have characteristic 0.
- Nathanael Leedom Ackerman (2006) Lecture notes Math 371, Positive definite bilinear form is definition 0.5.0.7, weblink from University of California, Berkeley.
- Kitaoka, Yoshiyuki (1993). Arithmetic of quadratic forms. Cambridge Tracts in Mathematics 106. Cambridge University Press. ISBN 0-521-40475-4. Zbl 0785.11021.
- Lang, Serge (2004), Algebra, Graduate Texts in Mathematics 211 (Corrected fourth printing, revised third ed.), New York: Springer-Verlag, p. 578, ISBN 978-0-387-95385-4
- Milnor, J.; Husemoller, D. (1973). Symmetric Bilinear Forms. Ergebnisse der Mathematik und ihrer Grenzgebiete 73. Springer-Verlag. ISBN 3-540-06009-X. Zbl 0292.10016.