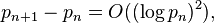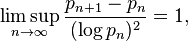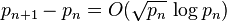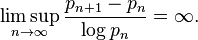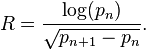Cramér's conjecture

In number theory, Cramér's conjecture, formulated by the Swedish mathematician Harald Cramér in 1936,[1] is an estimate for the size of gaps between consecutive prime numbers: intuitively, that gaps between consecutive primes are always small, and the conjecture quantifies asymptotically just how small they must be. It states that

p_{n+1}-p_n=O((\log p_n)^2),\

where pn denotes the nth prime number, O is big O notation, and "log" is the natural logarithm. While this is the statement explicitly conjectured by Cramér, his argument actually supports the stronger statement

\limsup_{n\rightarrow\infty} \frac{p_{n+1}-p_n}{(\log p_n)^2} = 1,

and this formulation is often called Cramér's conjecture in the literature.

Neither form of Cramér's conjecture has yet been proven or disproven.

Conditional proven results on prime gaps

Cramér gave a conditional proof of the much weaker statement that

p_{n+1}-p_n = O(\sqrt{p_n}\,\log p_n)

on the assumption of the Riemann hypothesis.[1]

In the other direction, E. Westzynthius proved in 1931 that prime gaps grow more than logarithmically. That is,[2]

\limsup_{n\to\infty}\frac{p_{n+1}-p_n}{\log p_n}=\infty.

Heuristic justification

Cramér's conjecture is based on a probabilistic model (essentially a heuristic) of the primes, in which one assumes that the probability of a natural number of size x being prime is 1/log x. This is known as the Cramér model of the primes. Cramér proved that in this model, the above conjecture holds true with probability one.[1]

Related conjectures and heuristics

Prime gap function

Daniel Shanks conjectured asymptotic equality of record gaps, a somewhat stronger statement than Cramér's conjecture.[3]

In the Cramér random model,

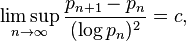\limsup_{n\rightarrow\infty} \frac{p_{n+1}-p_n}{(\log p_n)^2} = c, with c = 1.

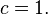
However, as pointed out by Andrew Granville,[4] Maier's theorem shows that the Cramér random model does not adequately describe the distribution of primes, and a refinement of Cramér's model taking into account divisibility by small primes suggests that 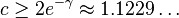c \ge 2e^{-\gamma}\approx1.1229\ldots ( A125313), where \gamma is the Euler–Mascheroni constant.


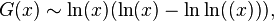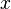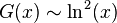In the paper <ref name=Cadwell1971">Cadwell, J. H. (1971), "Large Intervals Between Consecutive Primes", Mathematics of Computation 25 (116): 909–913, doi:10.2307/2004355</ref> J.H. Cadwell has proposed the formula for the maximal gaps: G(x)\sim \ln(x)(\ln(x)-\ln\ln((x))), which for large x goes into the Cramer's conjecture G(x)\sim \ln^2(x).

Thomas Nicely has calculated many large prime gaps.[5] He measures the quality of fit to Cramér's conjecture by measuring the ratio

R = \frac{\log(p_n)}{\sqrt{p_{n+1}-p_n}}.

He writes, “For the largest known maximal gaps, 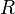R has remained near 1.13.” However, 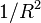1/R^2 is still less than 1, and it does not provide support to Granville's refinement that c should be greater than 1.

See also

References

  1. ↑ 1.0 1.1 1.2 Cramér, Harald (1936), "On the order of magnitude of the difference between consecutive prime numbers", Acta Arithmetica 2: 23–46
  2. ↑ Westzynthius, E. (1931), "Über die Verteilung der Zahlen die zu den n ersten Primzahlen teilerfremd sind", Commentationes Physico-Mathematicae Helsingsfors (in German) 5: 1–37, JFM 57.0186.02, Zbl 0003.24601.
  3. ↑ Shanks, Daniel (1964), "On Maximal Gaps between Successive Primes", Mathematics of Computation (American Mathematical Society) 18 (88): 646–651, doi:10.2307/2002951, JSTOR 2002951, Zbl 0128.04203.
  4. ↑ Granville, A. (1995), "Harald Cramér and the distribution of prime numbers", Scandinavian Actuarial Journal 1: 12–28, doi:10.1080/03461238.1995.10413946.
  5. ↑ Nicely, Thomas R. (1999), "New maximal prime gaps and first occurrences", Mathematics of Computation 68 (227): 1311–1315, doi:10.1090/S0025-5718-99-01065-0, MR 1627813.

External links