Cohn's irreducibility criterion

Arthur Cohn's irreducibility criterion is a sufficient condition for a polynomial to be irreducible in \mathbb{Z}[x]—that is, for it to be unfactorable into the product of lower-degree polynomials with integer coefficients.

The criterion is often stated as follows:

If a prime number p is expressed in base 10 as p=a_m10^m+a_{m-1}10^{m-1}+\cdots+a_110+a_0 (where 0\leq a_i\leq 9) then the polynomial
f(x)=a_mx^m+a_{m-1}x^{m-1}+\cdots+a_1x+a_0
is irreducible in \mathbb{Z}[x].

The theorem can be generalized to other bases as follows:

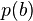Assume that b \ge 2 is a natural number and p(x)=a_kx^k+a_{k-1}x^{k-1}+\cdots+a_1x+a_0 is a polynomial such that 0\leq a_i\leq b-1. If p(b) is a prime number then p(x) is irreducible in \mathbb{Z}[x].

The base-10 version of the theorem attributed to Cohn by Pólya and Szegő in one of their books[1] while the generalization to any base, 2 or greater, is due to Brillhart, Filaseta, and Odlyzko.[2]

In 2002, Ram Murty gave a simplified proof as well as some history of the theorem in a paper that is available online.[3]

The converse of this criterion is that, if p is an irreducible polynomial with integer coefficients that have greatest common divisor 1, then there exists a base such that the coefficients of p form the representation of a prime number in that base; this is the Bunyakovsky conjecture and its truth or falsity remains an open question.

Historical notes

See also

References

  1. ↑ Pólya, George; Szegő, Gábor (1925). Aufgaben und Lehrsätze aus der Analysis, Bd 2. Springer, Berlin. OCLC 73165700. English translation in: Pólya, George; Szegő, Gábor (2004). Problems and theorems in analysis, volume 2 2. Springer. p. 137. ISBN 3-540-63686-2.
  2. ↑ Brillhart, John; Filaseta, Michael; Odlyzko, Andrew (1981). "On an irreducibility theorem of A. Cohn". Canadian Journal of Mathematics 33 (5): 1055–1059. doi:10.4153/CJM-1981-080-0.
  3. ↑ Murty, Ram (2002). "Prime Numbers and Irreducible Polynomials". American Mathematical Monthly (The American Mathematical Monthly, Vol. 109, No. 5) 109 (5): 452–458. doi:10.2307/2695645. JSTOR 2695645. (dvi file)
  4. ↑ Arthur Cohn's entry at the Mathematics Genealogy Project
  5. ↑ Siegmund-Schultze, Reinhard (2009). Mathematicians Fleeing from Nazi Germany: Individual Fates and Global Impact. Princeton, N.J.: Princeton University Press. p. 346. ISBN 9781400831401.

External links