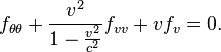Chaplygin's equation

In mathematics, Chaplygin's equation, named after Sergei Alekseevich Chaplygin, is a partial differential equation useful in the study of transonic flow.[1] It is

f_{\theta\theta}+ \frac{v^2}{1-\frac{v^2}{c^2}}f_{vv}+v f_v=0.

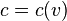Here, c=c(v) is the speed of sound, determined by the equation of state of the fluid and Bernoulli's principle.

Traveling wave plots

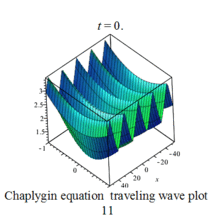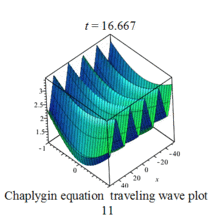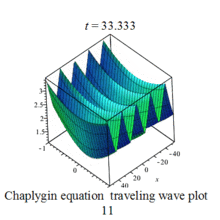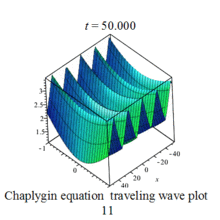Chaplygin equation traveling wave plot
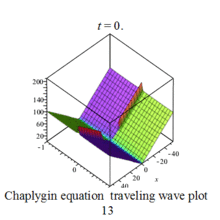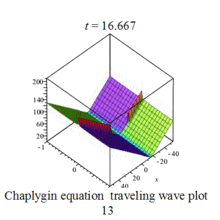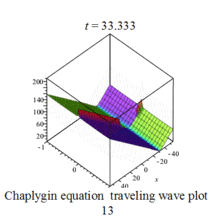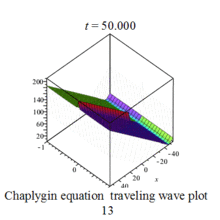Chaplygin equation traveling wave plot
Chaplygin equation traveling wave plot
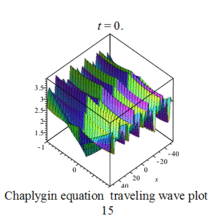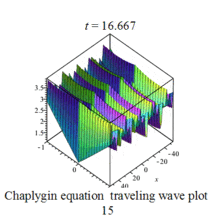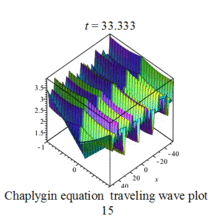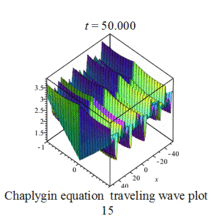Chaplygin equation traveling wave plot
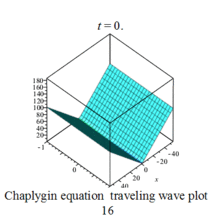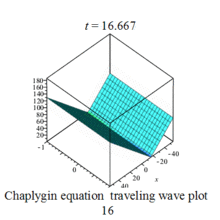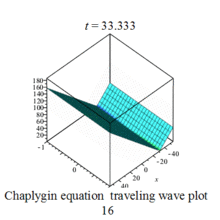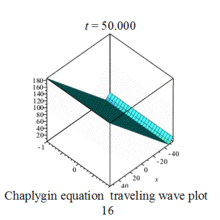Chaplygin equation traveling wave plot
Chaplygin equation traveling wave plot
Chaplygin equation traveling wave plot
Chaplygin equation traveling wave plot
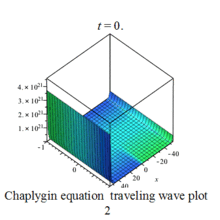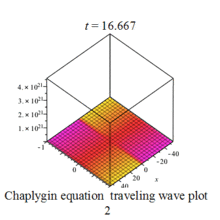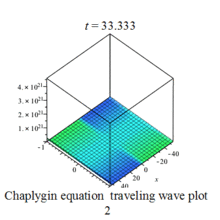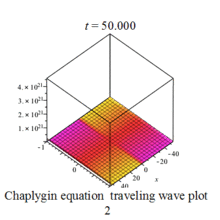Chaplygin equation traveling wave plot
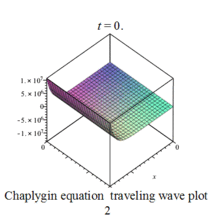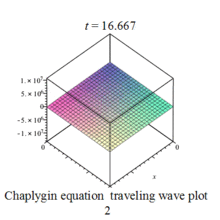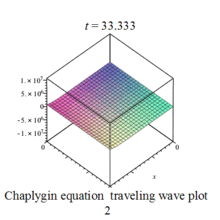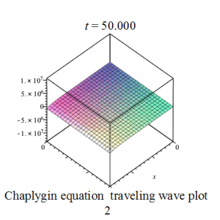Chaplygin equation traveling wave plot

References

  1. ↑ Landau, L. D.; Lifshitz, E. M. (1982). Fluid Mechanics (2 ed.). Pergamon Press. p. 432.