Canonical basis

In mathematics, a canonical basis is a basis of an algebraic structure that is canonical in a sense that depends on the precise context:

In a coordinate space, and more generally in a free module, it refers to the standard basis defined by the Kronecker delta.
In a polynomial ring, it refers to its standard basis given by the monomials, $(X^i)_i$ .
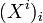For finite extension fields, it means the polynomial basis.

Representation theory

In representation theory there are several basis that are called "canonical", e.g. Lusztig's canonical basis and closely related Kashiwara's crystal basis in quantum groups and their representations. There is a general concept underlying these basis:

Consider the ring of integral Laurent polynomials $\mathcal{Z}:=\mathbb{Z}[v,v^{-1}]$ with its two subrings $\mathcal{Z}^{\pm}:=\mathbb{Z}[v^{\pm 1}]$ and the automorphism $\overline{\cdot}$ that is defined by $\overline{v}:=v^{-1}$ .

A precanonical structure on a free 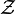$\mathcal{Z}$ -module 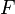$F$ consists of

A standard basis 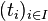$(t_i)_{i\in I}$ of $F$ ,
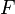A partial order on 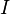$I$ that is interval finite, i.e. $(-\infty,i] := \{j\in I | j\leq i\}$ is finite for all $i\in I$ ,
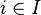A dualization operation, i.e. a bijection 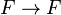$F\to F$ of order two that is $\overline{\cdot}$ -semilinear and will be denoted by $\overline{\cdot}$ as well.

If a precanonical structure is given, then one can define the 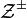$\mathcal{Z}^{\pm}$ submodule 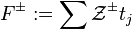$F^{\pm} := \sum \mathcal{Z}^{\pm} t_j$ of $F$ .

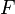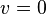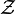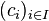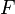A canonical basis at $v=0$ of the precanonical structure is then a $\mathcal{Z}$ -basis $(c_i)_{i\in I}$ of $F$ that satisfies:

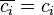$\overline{c_i}=c_i$ and
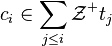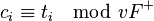
$c_i \in \sum_{j\leq i} \mathcal{Z}^+ t_j$ and $c_i \equiv t_i \mod vF^+$

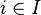for all $i\in I$ . A canonical basis at 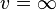$v=\infty$ is analogously defined to be a basis 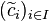$(\widetilde{c}_i)_{i\in I}$ that satisfies

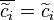$\overline{\widetilde{c}_i}=\widetilde{c}_i$ and
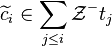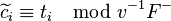
$\widetilde{c}_i \in \sum_{j\leq i} \mathcal{Z}^- t_j$ and $\widetilde{c}_i \equiv t_i \mod v^{-1}F^-$

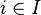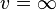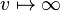for all $i\in I$ . The naming "at $v=\infty$ " alludes to the fact $\lim_{v\to\infty} v^{-1} =0$ and hence the "specialization" $v\mapsto\infty$ corresponds to quotienting out the relation $v^{-1}=0$ .

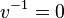One can show that there exists at most one canonical basis at v=0 (and at most one at 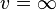$v=\infty$ ) for each precanonical structure. A sufficient condition for existence is that the polynomials 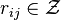$r_{ij}\in\mathcal{Z}$ defined by 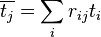$\overline{t_j}=\sum_i r_{ij} t_i$ satisfy 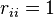$r_{ii}=1$ and $r_{ij}\neq 0 \implies i\leq j$ .

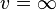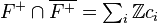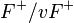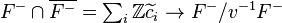A canonical basis at v=0 ( $v=\infty$ ) induces an isomorphism from $\textstyle F^+\cap \overline{F^+} = \sum_i \mathbb{Z}c_i$ to $F^+/vF^+$ ( $\textstyle F^{-} \cap \overline{F^{-}}=\sum_i \mathbb{Z}\widetilde{c}_i \to F^{-}/v^{-1} F^{-}$ respectively).

Examples

Quantum groups

The canonical basis of quantum groups in the sense of Lusztig and Kashiwara are canonical basis at 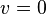$v=0$ .

Hecke algebras

Let $(W,S)$ be a Coxeter group. The corresponding Iwahori-Hecke algebra 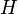$H$ has the standard basis 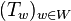$(T_w)_{w\in W}$ , the group is partially ordered by the Bruhat order which is interval finite and has a dualization operation defined by 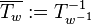$\overline{T_w}:=T_{w^{-1}}^{-1}$ . 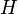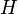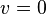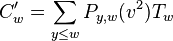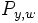This is a precanonical structure on $H$ that satisfies the sufficient condition above and the corresponding canonical basis of $H$ at $v=0$ is the Kazhdan-Lusztig basis $C_w' = \sum_{y\leq w} P_{y,w}(v^2) T_w$ with $P_{y,w}$ being the Kazhdan-Lusztig polynomials.

References