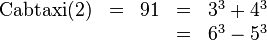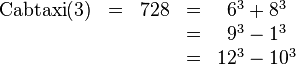Cabtaxi number

In mathematics, the n-th cabtaxi number, typically denoted Cabtaxi(n), is defined as the smallest positive integer that can be written as the sum of two positive or negative or 0 cubes in n ways. Such numbers exist for all n (since taxicab numbers exist for all n); however, only 10 are known (sequence A047696 in OEIS):

\begin{matrix}\mathrm{Cabtaxi}(1)&=&1&=&1^3 \pm 0^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(2)&=&91&=&3^3 + 4^3 \\&&&=&6^3 - 5^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(3)&=&728&=&6^3 + 8^3 \\&&&=&9^3 - 1^3 \\&&&=&12^3 - 10^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(4)&=&2741256&=&108^3 + 114^3 \\&&&=&140^3 - 14^3 \\&&&=&168^3 - 126^3 \\&&&=&207^3 - 183^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(5)&=&6017193&=&166^3 + 113^3 \\&&&=&180^3 + 57^3 \\&&&=&185^3 - 68^3 \\&&&=&209^3 - 146^3 \\&&&=&246^3 - 207^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(6)&=&1412774811&=&963^3 + 804^3 \\&&&=&1134^3 - 357^3 \\&&&=&1155^3 - 504^3 \\&&&=&1246^3 - 805^3 \\&&&=&2115^3 - 2004^3 \\&&&=&4746^3 - 4725^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(7)&=&11302198488&=&1926^3 + 1608^3 \\&&&=&1939^3 + 1589^3 \\&&&=&2268^3 - 714^3 \\&&&=&2310^3 - 1008^3 \\&&&=&2492^3 - 1610^3 \\&&&=&4230^3 - 4008^3 \\&&&=&9492^3 - 9450^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(8)&=&137513849003496&=&22944^3 + 50058^3 \\&&&=&36547^3 + 44597^3 \\&&&=&36984^3 + 44298^3 \\&&&=&52164^3 - 16422^3 \\&&&=&53130^3 - 23184^3 \\&&&=&57316^3 - 37030^3 \\&&&=&97290^3 - 92184^3 \\&&&=&218316^3 - 217350^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(9)&=&424910390480793000&=&645210^3 + 538680^3 \\&&&=&649565^3 + 532315^3 \\&&&=&752409^3 - 101409^3 \\&&&=&759780^3 - 239190^3 \\&&&=&773850^3 - 337680^3 \\&&&=&834820^3 - 539350^3 \\&&&=&1417050^3 - 1342680^3 \\&&&=&3179820^3 - 3165750^3 \\&&&=&5960010^3 - 5956020^3\end{matrix}
\begin{matrix}\mathrm{Cabtaxi}(10)&=&933528127886302221000&=&77480130^3 - 77428260^3 \\&&&=&41337660^3 - 41154750^3 \\&&&=&18421650^3 - 17454840^3 \\&&&=&10852660^3 - 7011550^3 \\&&&=&10060050^3 - 4389840^3 \\&&&=&9877140^3 - 3109470^3 \\&&&=&9781317^3 - 1318317^3 \\&&&=&9773330^3 - 84560^3 \\&&&=&8444345^3 + 6920095^3 \\&&&=&8387730^3 + 7002840^3\end{matrix}

Cabtaxi(5), Cabtaxi(6) and Cabtaxi(7) were found by Randall L. Rathbun; Cabtaxi(8) was found by Daniel J. Bernstein; Cabtaxi(9) was found by Duncan Moore, using Bernstein's method. Cabtaxi(10) was first reported as an upper bound by Christian Boyer in 2006 and verified as Cabtaxi(10) by Uwe Hollerbach and reported on the NMBRTHRY mailing list on May 16, 2008.

See also

External links