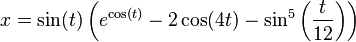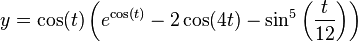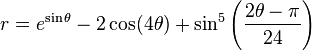Butterfly curve (transcendental)

The butterfly curve.

The butterfly curve is a transcendental plane curve discovered by Temple H. Fay. The curve is given by the following parametric equations:

x = \sin(t) \left(e^{\cos(t)} - 2\cos(4t) - \sin^5\left({t \over 12}\right)\right)
y = \cos(t) \left(e^{\cos(t)} - 2\cos(4t) - \sin^5\left({t \over 12}\right)\right)

or by the following polar equation:

r=e^{\sin \theta} - 2 \cos (4 \theta ) + \sin^5\left(\frac{2 \theta - \pi}{24}\right)

See also

References

External links