Black Hole (solitaire)

A Deal of Black Hole Solitaire in PySolFC

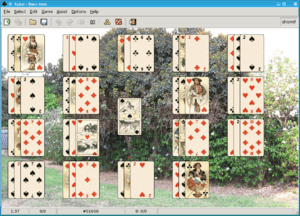
Black Hole is a solitaire card game that is akin to Golf and Tri Peaks, but its tableau is somewhat like that of La Belle Lucie. Invented by David Parlett, this game's objective is to compress the entire deck into one foundation.

The cards are dealt to the tableau in piles of three. The leftover card, dealt first or last, is placed as a single foundation called the Black Hole. This card usually is the Ace of Spades, but any card can do.^[1]

Only the top cards of each pile in the tableau are available for play and in order for a card to be placed in the Black Hole, it must be a rank higher or lower than the top card on the Black Hole. This is the only allowable move in the entire game.^[2]

The game ends if there are no more top cards that can be moved to the Black Hole. The game is won if all of the cards end up in the Black Hole.

Solvers and Solvability Statistics

Shlomi Fish has written an open-source solver for Black Hole Solitaire^[3] first as a Perl-based CPAN module, but which was later re-implemented in the C programming language. The solver was run on the first one million PySolFC Black Hole Solitaire deals^[4] and generated some statistics of using its Depth-first search (DFS) scan.

Out of the million deals 869,413 could be solved and the 130,587 others were fully traversed without a possible final solution. The search iterations counts of both the solved and unsolved deals had fairly large averages (roughly 292,400 and 553,884) and standard deviations which indicates that some deals result in many false ends. The median number of iterations for the solved states was also relatively high — about 79,000.

References

1. ↑ Free World Group - Black Hole Solitaire instructions
2. ↑ PySolFC Game Rules for Black Hole
3. ↑ Black Hole Solitaire Solver
4. ↑ Solving Statistics for the First 1 Million PySolFC Black Hole Solitaire Deals

Similar Games

• All in a Row - played with 13 columns of 4-cards each, and at the start of play, the first move can be made from any column.

Patience or Solitaire (US) - (list) Adding and pairing Baroness Fourteen Out Gay Gordons Golf Monte Carlo Nestor Pyramid Bisley Baker's Dozen Capricieuse Martha Perseverance Castle Beleaguered Castle Congress Fortress Seahaven Towers Canfield American Toad Duchess Eagle Wing Fan Alternation Bristol Flower Garden House in the Woods Intelligence La Belle Lucie The Plot Shamrocks Forty Thieves Deuces Diplomat Emperor Miss Milligan Napoleon's Square FreeCell Baker's Game Eight Off Penguin Stalactites Klondike Agnes Batsford Gargantua King Albert Seven Devils Westcliff Yukon Non-builder Accordion Clock Gaps Maze Perpetual Motion Poker Squares Royal Marriage Pocket Decade One-Handed Spider Auld Lang Syne Blockade Curds and Whey Labyrinth Mrs. Mop Scorpion Simple Simon Other Aces and Kings Algerian Black Hole Carpet King's Audience The Clock Glossary Grandfather's Clock