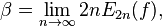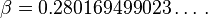Bernstein's constant

Binary	0.01000111101110010011000000110011…
Decimal	0.280169499…
Hexadecimal	0.47B930338AAD…
Continued fraction	$\cfrac{1}{3 + \cfrac{1}{1 + \cfrac{1}{1 + \cfrac{1}{3 + \cfrac{1}{9+ \ddots}}}}}$

Bernstein's constant, usually denoted by the Greek letter β (beta), is a mathematical constant named after Sergei Natanovich Bernstein and is approximately equal to 0.2801694990.

Definition

Let E_n(ƒ) be the error of the best uniform approximation to a real function ƒ(x) on the interval [−1, 1] by real polynomials of no more than degree n. In the case of ƒ(x) = |x|, Bernstein (1914) showed that the limit

\beta=\lim_{n \to \infty}2nE_{2n}(f),\,

called Bernstein's constant, exists and is between 0.278 and 0.286. His conjecture that the limit is:

\frac {1}{2\sqrt {\pi}}=0.28209\dots\,.

was disproven by Varga & Carpenter (1987), who calculated

\beta=0.280169499023\dots\,.

References

Bernstein, S. N. (1914), "Sur la meilleure approximation de x par des polynomes de degrés donnés", Acta Math. 37: 1–57, doi:10.1007/BF02401828
Varga, Richard S.; Carpenter, Amos J. (1987), "A conjecture of S. Bernstein in approximation theory", Math. USSR Sbornik 57 (2): 547–560, doi:10.1070/SM1987v057n02ABEH003086, MR 0842399
Weisstein, Eric W., "Bernstein's Constant", MathWorld.