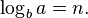Base (exponentiation)

Calculation results
Addition (+)
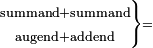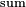\scriptstyle\left.\begin{matrix}\scriptstyle\text{summand}+\text{summand}\\\scriptstyle\text{augend}+\text{addend}\end{matrix}\right\}= \scriptstyle\text{sum}
Subtraction (−)
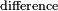\scriptstyle\text{minuend}-\text{subtrahend}= \scriptstyle\text{difference}
Multiplication (×)
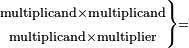\scriptstyle\left.\begin{matrix}\scriptstyle\text{multiplicand}\times\text{multiplicand}\\\scriptstyle\text{multiplicand}\times\text{multiplier}\end{matrix}\right\}= \scriptstyle\text{product}
Division (÷)
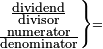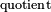\scriptstyle\left.\begin{matrix}\scriptstyle\frac{\scriptstyle\text{dividend}}{\scriptstyle\text{divisor}}\\\scriptstyle\frac{\scriptstyle\text{numerator}}{\scriptstyle\text{denominator}}\end{matrix}\right\}= \scriptstyle\text{quotient}
Modulation (mod)
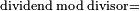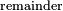\scriptstyle\text{dividend}\bmod\text{divisor}= \scriptstyle\text{remainder}
Exponentiation
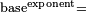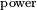\scriptstyle\text{base}^\text{exponent}= \scriptstyle\text{power}
nth root (√)
\scriptstyle\sqrt[\text{degree}]{\scriptstyle\text{radicand}}= \scriptstyle\text{root}
Logarithm (log)
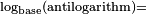\scriptstyle\log_\text{base}(\text{antilogarithm})= \scriptstyle\text{logarithm}
Exponentiation of numbers 0-10. Line labels = base. X axis = power. Y axis = result.

In exponentiation, the base is the number b in an expression of the form bn.

Related terms

The number n is called the exponent and the expression is known formally as exponentiation of b by n or the exponential of n with base b. It is more commonly expressed as "the nth power of b", "b to the nth power" or "b to the power n". For example, the fourth power of 10 is 10,000 because 104 = 10 x 10 x 10 x 10 = 10,000. The term power strictly refers to the entire expression, but is sometimes used to refer to the exponent.

Roots

When the nth power of b equals a number a, or  a = bn , then b is called an "nth root" of a. For example, 10 is a fourth root of 10,000.

Logarithms

The inverse function to exponentiation with base b (when it is well-defined) is called the logarithm to base b, denoted logb. Thus:

\log_b a = n. \,

For example, 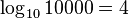\log_{10} 10000 = 4.

See also