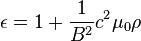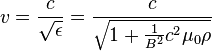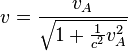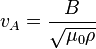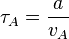Alfvén wave

In plasma physics, an Alfvén wave, named after Hannes Alfvén, is a type of magnetohydrodynamic wave in which ions oscillate in response to a restoring force provided by an effective tension on the magnetic field lines.^[1]

Definition

An Alfvén wave in a plasma is a low-frequency (compared to the ion cyclotron frequency) travelling oscillation of the ions and the magnetic field. The ion mass density provides the inertia and the magnetic field line tension provides the restoring force.

The wave propagates in the direction of the magnetic field, although waves exist at oblique incidence and smoothly change into the magnetosonic wave when the propagation is perpendicular to the magnetic field.

The motion of the ions and the perturbation of the magnetic field are in the same direction and transverse to the direction of propagation. The wave is dispersionless.

Alfvén velocity

The low-frequency relative permittivity $\epsilon\,$ of a magnetized plasma is given by

\epsilon = 1 + \frac{1}{B^2}c^2 \mu_0 \rho

where $B\,$ is the magnetic field strength, $c\,$ is the speed of light, $\mu_0\,$ is the permeability of the vacuum, and $\rho = \Sigma n_s m_s\,$ is the total mass density of the charged plasma particles. Here, $s\,$ goes over all plasma species, both electrons and (few types of) ions.

Therefore, the phase velocity of an electromagnetic wave in such a medium is

v = \frac{c}{\sqrt{\epsilon}} = \frac{c}{\sqrt{1 + \frac{1}{B^2}c^2 \mu_0 \rho}}

v = \frac{v_A}{\sqrt{1 + \frac{1}{c^2}v_A^2}}

where

v_A = \frac{B}{\sqrt{\mu_0 \rho}}

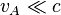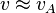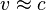is the Alfvén velocity. If $v_A \ll c$ , then $v \approx v_A$ . On the other hand, when $v_A \gg c$ , then $v \approx c$ . That is, at high field or low density, the velocity of the Alfvén wave approaches the speed of light, and the Alfvén wave becomes an ordinary electromagnetic wave.

Neglecting the contribution of the electrons to the mass density and assuming that there is a single ion species, we get

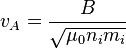v_A = \frac{B}{\sqrt{\mu_0 n_i m_i}}~~

in SI

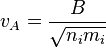v_A = \frac{B}{\sqrt{n_i m_i}}~~

in Gauss

v_A \approx (2.18\times10^{11}\,\mbox{cm/s})\,(m_i/m_p)^{-1/2}\,(n_i/{\rm cm}^{-3})^{-1/2}\,(B/{\rm gauss})

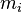where $n_i\,$ is the ion number density and $m_i\,$ is the ion mass.

Alfvén time

In plasma physics, the Alfvén time $\tau_A$ is an important timescale for wave phenomena. It is related to the Alfvén velocity by:

\tau_A = \frac{a}{v_A}

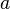where $a$ denotes the characteristic scale of the system, for example 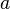$a$ is the minor radius of the torus in a tokamak.

Relativistic case

The general Alfvén wave velocity is defined by Gedalin (1993):^[2]

v = \frac{c}{\sqrt{1 + \frac{e + P}{2 P_m}}}

where

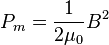$e\,$ is the total energy density of plasma particles, $P\,$ is the total plasma pressure, and $P_m = \frac{1}{2\mu_0}B^2\,$ is the magnetic field pressure. In the non-relativistic limit $P \ll e \approx \rho c^2$ , and we immediately get the expression from the previous section.

Heating the Corona

Cold plasma floating in the corona above the solar limb. Alfvén waves were observed for the first time, extrapolated from fluctuations of the plasma.

The coronal heating problem is a longstanding question in heliophysics. It is unknown why the sun's corona lives in a temperature range higher than one million degrees while the sun's surface (photosphere) is only a few thousand degrees in temperature. Natural intuition would predict a decrease in temperature while getting farther away from a heat source, but it is theorized that the photosphere, influenced by the sun's magnetic fields, emits certain waves which carry energy (i.e. heat) to the corona and solar wind. It is important to note that because the density of the corona is quite a bit smaller than the photosphere, the heat and energy level of the photosphere is much higher than the corona. Temperature is only the average speed of a species, and less energy is required to heat fewer particles to higher temperatures in the coronal atmosphere. Alfvén first proposed the existence of an electromagnetic-hydrodynamic wave in 1942 in Nature. He claimed the sun had all necessary criteria to support these waves and that they may in turn be responsible for sun spots. From his paper:

If a conducting liquid is placed in a constant magnetic field, every motion of the liquid gives rise to an E.M.F. which produces electric currents. Owing to the magnetic field, these currents give mechanical forces which change the state of motion of the liquid. Thus a kind of combined electromagnetic-hydrodynamic wave is produced.
—Hannes Alfvén, Existence of Electromagnetic-Hydrodynamic Waves, ^[3]

Beneath the sun's photosphere lies the convection zone. The rotation of the sun, as well as varying pressure gradients beneath the surface, produces the periodic electromagnetism in the convection zone which can be observed on the sun's surface. This random motion of the surface gives rise to Alfvén waves. The waves travel through the chromosphere and transition zone and interact with much of the ionized plasma. The wave itself carries energy as well as some of the electrically charged plasma. De Pointe^[4] and Haerendel ^[5] suggested in the early 1990s that Alfven waves may also be associated with the plasma jets known as spicules. It was theorized these brief spurts of superheated gas were carried by the combined energy and momentum of their own upward velocity, as well as the oscillating transverse motion of the Alfven waves. In 2007, Alfven waves were reportedly observed for the first time traveling towards the corona by Tomcyzk et al., but their predictions could not conclude that the energy carried by the Alfven waves were sufficient enough to heat the corona to its enormous temperatures, for the observed amplitudes of the waves were not high enough.^[6] However, in 2011, McIntosh et al. reported the observation of highly energetic Alfven waves combined with energetic spicules which could sustain heating the corona to its million Kelvin temperature. These observed amplitudes (20.0 km/s against 2007's observed 0.5 km/s) contained over one hundred times more energy than the ones observed in 2007.^[7] The short period of the waves also allowed more energy transfer into the coronal atmosphere. The 50,000 km long spicules may also play a part in accelerating the solar wind past the corona.^[8]

History

How this phenomenon became understood

1942: Alfvén suggests the existence of electromagnetic-hydromagnetic waves in a paper published in Nature.
1949: Laboratory experiments by S. Lundquist produce such waves in magnetized mercury, with a velocity that approximated Alfvén's formula.
1949: Enrico Fermi uses Alfvén waves in his theory of cosmic rays. According to Alex Dessler in a 1970 Science journal article, Fermi had heard a lecture at the University of Chicago, Fermi nodded his head exclaiming "of course" and the next day, the physics world said "of course".
1950: Alfvén publishes the first edition of his book, Cosmical Electrodynamics, detailing hydromagnetic waves, and discussing their application to both laboratory and space plasmas.
1952: Additional confirmation appears in experiments by Winston Bostick and Morton Levine with ionized helium
1954: Bo Lehnert produces Alfvén waves in liquid sodium
1958: Eugene Parker suggests hydromagnetic waves in the interstellar medium
1958: Berthold, Harris, and Hope detect Alfvén waves in the ionosphere after the Argus nuclear test, generated by the explosion, and traveling at speeds predicted by Alfvén formula.
1958: Eugene Parker suggests hydromagnetic waves in the Solar corona extending into the Solar wind.
1959: D. F. Jephcott produces Alfvén waves in a gas discharge
1959: C. H. Kelley and J. Yenser produce Alfvén waves in the ambient atmosphere.
1960: Coleman, et al., report the measurement of Alfvén waves by the magnetometer aboard the Pioneer and Explorer satellites
1960: Sugiura suggests evidence of hydromagnetic waves in the Earth's magnetic field
1961: Normal Alfvén modes and resonances in liquid sodium are studied by Jameson
1966: R.O.Motz generates and observes Alfven waves in mercury
1970 Hannes Alfvén wins the 1970 Nobel Prize in physics for "fundamental work and discoveries in magneto-hydrodynamics with fruitful applications in different parts of plasma physics"
1973: Eugene Parker suggests hydromagnetic waves in the intergalactic medium
1974: Hollweg suggests the existence of hydromagnetic waves in interplanetary space
1974: Ip and Mendis suggests the existence of hydromagnetic waves in the coma of Comet Kohoutek.
1984: Roberts et al. predict the presence of standing MHD waves in the solar corona, thus leading to the field of coronal seismology.
1999: Aschwanden, et al. and Nakariakov, et al. report the detection of damped transverse oscillations of solar coronal loops observed with the EUV imager on board the Transition Region And Coronal Explorer (TRACE), interpreted as standing kink (or "Alfvénic") oscillations of the loops. This fulfilled the prediction of Roberts et al. (1984).
2007: Tomczyk, et al., report the detection of Alfvénic waves in images of the solar corona with the Coronal Multi-Channel Polarimeter (CoMP) instrument at the National Solar Observatory, New Mexico. These waves were interpreted as propagating kink waves by Van Doorsselaere et al. (2008)
2007: Alfvén wave discoveries appear in articles by Jonathan Cirtain and colleagues, Takenori J. Okamoto and colleagues, and Bart De Pontieu and colleagues. De Pontieu's team proposed that the energy associated with the waves is sufficient to heat the corona and accelerate the solar wind. These results appear in a special collection of 10 articles, by scientists in Japan, Europe and the United States, in the 7 December issue of the journal Science. It was demonstrated that those waves should be interpreted in terms of kink waves of coronal plasma structures by Van Doorsselaere, et al. (2008); Ofman and Wang (2008); and Vasheghani Farahani, et al. (2009).
2008: Kaghashvili et al. proposed how the detected oscilations can be used to deduct properties of Alfven waves. The mechanism is based on the formalism developed by the Kaghashvili and his collaborators. ^[9]
2011: Experimental evidence of Alfvén wave propagation in a Gallium alloy^[10]

References

↑ Iwai, K; Shinya, K,; Takashi, K. and Moreau, R. (2003) "Pressure change accompanying Alfvén waves in a liquid metal" Magnetohydrodynamics 39(3): pp. 245-250, page 245
↑ Gedalin, M. (1993), "Linear waves in relativistic anisotropic magnetohydrodynamics", Physical Review E 47 (6): 4354–4357, Bibcode:1993PhRvE..47.4354G, doi:10.1103/PhysRevE.47.4354
↑ Hannes Alfvén (1942). "Existence of Electromagnetic-Hydrodynamic Waves". Nature 150 (3805): 405–406. Bibcode:1942Natur.150..405K. doi:10.1038/150405a0.
↑ Bart de Pontieu (18 December 1997). "Chromospheric Spicules driven by Alfvén waves". Max-Planck-Institut für extraterrestrische Physik. Retrieved 1 April 2012.
↑ Gerhard Haerendel (1992). "Weakly damped Alfven waves as drivers of solar chromospheric spicules". Nature 360: 241–243. Bibcode:1992Natur.360..241H. doi:10.1038/360241a0.
↑ Tomczyk, S., McIntosh, S.W., Keil, S.L., Judge, P.G., Schad, T., Seeley, D.H., Edmondson, J. (2007). "Alfven waves in the solar corona". Science 317 (5842): 1192–1196. doi:10.1126/science.1143304.
↑ McIntosh et al. (2011). "Alfvenic waves with sufficient energy to power the quiet solar corona and fast solar wind.". Nature 475 (7357): 477. Bibcode:2011Natur.475..477M. doi:10.1038/nature10235.
↑ Karen Fox (27 July 2011). "SDO Spots Extra Energy in the Sun's Corona.". NASA. Retrieved 2 April 2012.
↑ http://adsabs.harvard.edu/abs/2009ApJ...703.1318K
↑ Thierry Alboussière, Philippe Cardin, François Debray, Patrick La Rizza, Jean-Paul Masson, Franck Plunian, Adolfo Ribeiro, Denys Schmitt (2011). "Experimental evidence of Alfvén wave propagation in a Gallium alloy". Phys. Fluids 23 (9): 096601. arXiv:1106.4727. Bibcode:2011PhFl...23i6601A. doi:10.1063/1.3633090.

External links

Mysterious Solar Ripples Detected Dave Mosher 2 September 2007 Space.com
EurekAlert! notification of 7 December 2007 Science special issue
EurekAlert! notification: "Scientists find solution to solar puzzle"