Zero-forcing precoding

From Wikipedia, the free encyclopedia

Zero-forcing (or Null-Steering) precoding is a spatial signal processing by which the multiple antenna transmitter can null multiuser interference signals in wireless communications. Regularized zero-forcing precoding is enhanced processing to consider the impact on a background noise and unknown user interference,^[1] where the background noise and the unknown user interference can be emphasized in the result of (known) interference signal nulling.

In particular, Null-Steering is a method of beamforming for narrowband signals where we want to have a simple way of compensating delays of receiving signals from a specific source at different elements of the antenna array. In general to make use of the antenna arrays, we better to sum and average the signals coming to different elements, but this is only possible when delays are equal. Otherwise we first need to compensate the delays and then to sum them up. To reach this goal, we may only add the weighted version of the signals with appropriate weight values. We do this in such a way that the frequency domain output of this weighted sum produces a zero result. This method is called null steering. The generated weights are of course related to each other and this relation is a function of delay and central working frequency of the source.

Performance of Zero-forcing Precoding

If the transmitter knows the downlink channel state information (CSI) perfectly, ZF-precoding can achieve almost the system capacity when the number of users is large. On the other hand, with limited channel state information at the transmitter (CSIT) the performance of ZF-precoding decreases depending on the accuracy of CSIT. ZF-precoding requires the significant feedback overhead with respect to signal-to-noise-ratio (SNR) so as to achieve the full multiplexing gain.^[2] Inaccurate CSIT results in the significant throughput loss because of residual multiuser interferences. Multiuser interferences remain since they can not be nulled with beams generated by imperfect CSIT.

Mathematical description

In a multiple antenna downlink system which comprises a $N_{t}$ transmit antenna access point (AP) and $K$ single receive antenna users, the received signal of user $k$ is described as

$y_{k}={\mathbf {h}}_{k}^{T}{\mathbf {x}}+n_{k},\quad k=1,2,\ldots ,K$

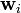where ${\mathbf {x}}=\sum _{{i=1}}^{K}s_{i}P_{i}{\mathbf {w}}_{i}$ is the $N_{t}\times 1$ vector of transmitted symbols, $n_{k}$ is the noise signal, ${\mathbf {h}}_{k}$ is the $N_{t}\times 1$ channel vector and ${\mathbf {w}}_{i}$ is the $N_{t}\times 1$ linear precoding vector. From the fact that each beam generated by ZF-precoding is orthogonal to all the other user channel vectors, one can rewrite the received signal as

$y_{k}={\mathbf {h}}_{k}^{T}\sum _{{i=1}}^{K}s_{i}P_{i}{\mathbf {w}}_{i}+n_{k}={\mathbf {h}}_{k}^{T}s_{k}P_{k}{\mathbf {w}}_{k}+n_{k},\quad k=1,2,\ldots ,K$

For comparison purpose, we describe the received signal model for multiple antenna uplink systems. In the uplink system with a $N_{r}$ receiver antenna AP and $K$ K single transmit antenna user, the received signal at the AP is described as

${\mathbf {y}}=\sum _{{i=1}}^{{K}}s_{i}{\mathbf {h}}_{i}+{\mathbf {n}}$

where $s_{i}$ is the transmitted signal of user $i$ , ${\mathbf {n}}$ is the $N_{r}\times 1$ noise vector, ${\mathbf {h}}_{k}$ is the $N_{r}\times 1$ channel vector.

Quantify the feedback amount

Quantify the amount of the feedback resource required to maintain at least a given throughput performance gap between zero-forcing with perfect feedback and with limited feedback, i.e.,

$\Delta R=R_{{ZF}}-R_{{FB}}\leq log_{2}g$ .

Jindal showed that the required feedback bits of a spatially uncorrelated channel should be scaled according to SNR of the downlink channel, which is given by:^[2]

$B=(M-1)\log _{2}\rho _{{b,m}}-(M-1)\log _{2}(g-1)$

where M is the number of transmit antennas and $\rho _{{b,m}}$ is the SNR of the downlink channel.

To feed back B bits though the uplink channel, the throughput performance of the uplink channel should be larger than or equal to 'B'

$b_{{FB}}\log _{2}(1+\rho _{{FB}})\geq B$

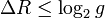where $b=\Omega _{{FB}}T_{{FB}}$ is the feedback resource consisted by multiplying the feedback frequency resource and the frequency temporal resource subsequently and $\rho _{{FB}}$ is SNR of the feedback channel. Then, the required feedback resource to satisfy $\Delta R\leq \log _{2}g$ is

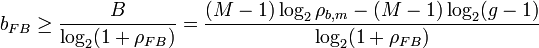$b_{{FB}}\geq {\frac {B}{\log _{2}(1+\rho _{{FB}})}}={\frac {(M-1)\log _{2}\rho _{{b,m}}-(M-1)\log _{2}(g-1)}{\log _{2}(1+\rho _{{FB}})}}$ .

Note that differently from the feedback bits case, the required feedback resource is a function of both downlink and uplink channel conditions. It is reasonable to include the uplink channel status in the calculation of the feedback resource since the uplink channel status determines the capacity, i.e., bits/second per unit frequency band (Hz), of the feedback link. Consided a case when SNR of the downlink and uplink are proportion such that $\rho _{{b,m}}/\rho _{{FB}})=C_{{up,dn}}$ is constant and both SNRs are sufficiently high. Then, the feedback resource will be only proportional to the number of transmit antennas

$b_{{FB,min}}^{*}=\lim _{{\rho _{{FB}}\to \infty }}{\frac {(M-1)\log _{2}\rho _{{b,m}}-(M-1)\log _{2}(g-1)}{\log _{2}(1+\rho _{{FB}})}}=M-1$ .

It follows from the above equation that the feedback resource ( $b_{{FB}}$ ) is not necessary to scale according to SNR of the downlink channel, which is almost contradict to the case of the feedback bits. One, hence, sees that the whole systematic analysis can reverse the facts resulted from each reductioned situation.

References

↑ B. C. B. Peel, B. M. Hochwald, and A. L. Swindlehurst (Jan. 2005). "A vector-perturbation technique for near-capacity multiantenna multiuser communication - Part I: channel inversion and regularization". IEEE Trans. Commun. 53: 195–202. doi:10.1109/TCOMM.2004.840638. Check date values in: |date= (help)
↑ 2.0 2.1 N. Jindal (Nov. 2006). "MIMO Broadcast Channels with Finite Rate Feedback". IEEE Trans. Information Theory. 52 (11): 5045–5059. doi:10.1109/TIT.2006.883550. Check date values in: |date= (help)

External links

Schelkunoff Polynomial Method (Null-Steering) www.antenna-theory.com

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.