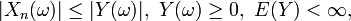Uniform integrability

From Wikipedia, the free encyclopedia

Uniform integrability is an important concept in real analysis, functional analysis and measure theory, and plays a vital role in the theory of martingales.

Formal definition

The following definition applies.^[1]

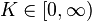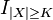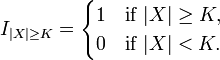A class ${\mathcal {C}}$ of random variables is called uniformly integrable (UI) if given $\epsilon >0$ , there exists $K\in [0,\infty )$ such that $E(|X|I_{{|X|\geq K}})\leq \epsilon \ {\text{ for all X}}\in {\mathcal {C}}$ , where $I_{{|X|\geq K}}$ is the indicator function $I_{{|X|\geq K}}={\begin{cases}1&{\text{if }}|X|\geq K,\\0&{\text{if }}|X|<K.\end{cases}}$ .

An alternative definition involving two clauses may be presented as follows: A class of random variables is called uniformly integrable if:
- There exists a finite $K$ such that, for every $X$ in ${\mathcal {C}}$ , ${\mathrm E}(|X|)\leqslant K$ .
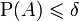- For every $\epsilon >0$ there exists $\delta >0$ such that, for every measurable $A$ such that ${\mathrm P}(A)\leqslant \delta$ and every $X$ in ${\mathcal {C}}$ , ${\mathrm E}(|X|:A)\leqslant \epsilon$ .

Related corollaries

The following results apply.^{[citation needed]}

Definition 1 could be rewritten by taking the limits as

$\lim _{{K\to \infty }}\sup _{{X\in {\mathcal {C}}}}E(|X|I_{{|X|\geq K}})=0.$

A non-UI sequence. Let $\Omega ={\mathbb {R}}$ , and define

$X_{n}(\omega )={\begin{cases}n,&\omega \in (0,1/n),\\0,&{\text{otherwise.}}\end{cases}}$

Clearly $X_{n}\in L^{1}$ , and indeed $E(|X_{n}|)=1\ ,$ for all n. However,

$E(|X_{n}|,|X_{n}|\geq K)=1\ {\text{ for all }}n\geq K,$

and comparing with definition 1, it is seen that the sequence is not uniformly integrable.

Non-UI sequence of RVs. The area under the strip is always equal to 1, but 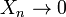$X_{n}\to 0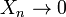$ pointwise.

By using Definition 2 in the above example, it can be seen that the first clause is not satisfied as the 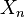$X_{n}$ s are not bounded in $L^{1}$ . If $X$ is a UI random variable, by splitting

$E(|X|)=E(|X|,|X|>K)+E(|X|,|X|<K)$

and bounding each of the two, it can be seen that a uniformly integrable random variable is always bounded in $L^{1}$ . It can also be shown that any $L^{1}$ random variable will satisfy clause 2 in Definition 2.

If any sequence of random variables 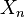$X_{n}$ is dominated by an integrable, non-negative $Y$ : that is, for all ω and n,

$\ |X_{n}(\omega )|\leq |Y(\omega )|,\ Y(\omega )\geq 0,\ E(Y)<\infty ,$

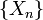then the class ${\mathcal {C}}$ of random variables $\{X_{n}\}$ is uniformly integrable.

A class of random variables bounded in 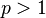$L^{p}$ ( $p>1$ ) is uniformly integrable.

Relevant theorems

Dunford–Pettis theorem^[2]

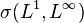A class of random variables $X_{n}\subset L^{1}(\mu )$ is uniformly integrable if and only if it is relatively compact for the weak topology $\sigma (L^{1},L^{\infty })$ .

de la Vallée-Poussin theorem^[3]

The family 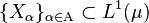$\{X_{{\alpha }}\}_{{\alpha \in \mathrm{A} }}\subset L^{1}(\mu )$ is uniformly integrable if and only if there exists a non-negative increasing convex function 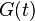$G(t)$ such that

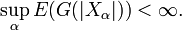
$\lim _{{t\to \infty }}{\frac {G(t)}{t}}=\infty$ and $\sup _{{\alpha }}E(G(|X_{{\alpha }}|))<\infty .$

Relation to convergence of random variables

Main article: convergence of random variables

A sequence 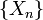$\{X_{n}\}$ converges to $X$ in the $L_{1}$ norm if and only if it converges in measure to $X$ and it is uniformly integrable. In probability terms, a sequence of random variables converging in probability also converge in the mean if and only if they are uniformly integrable.^[4] This is a generalization of the dominated convergence theorem.

Citations

↑ Williams, David (1997). Probability with Martingales (Repr. ed.). Cambridge: Cambridge Univ. Press. pp. 126–132. ISBN 978-0-521-40605-5.
↑ Dellacherie, C. and Meyer, P.A. (1978). Probabilities and Potential, North-Holland Pub. Co, N. Y. (Chapter II, Theorem T25).
↑ Meyer, P.A. (1966). Probability and Potentials, Blaisdell Publishing Co, N. Y. (p.19, Theorem T22).
↑ Bogachev, Vladimir I. (2007). Measure Theory Volume I. Berlin Heidelberg: Springer-Verlag. p. 268. doi:10.1007/978-3-540-34514-5_4. ISBN 3-540-34513-2.

References

A.N. Shiryaev (1995). Probability (2 ed.). New York: Springer-Verlag. pp. 187–188. ISBN 978-0-387-94549-1.
Walter Rudin (1987). Real and Complex Analysis (3 ed.). Singapore: McGraw–Hill Book Co. p. 133. ISBN 0-07-054234-1.
J. Diestel and J. Uhl (1977). Vector measures, Mathematical Surveys 15, American Mathematical Society, Providence, RI ISBN 978-0-8218-1515-1

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.