Truncated tesseractic honeycomb

From Wikipedia, the free encyclopedia
Truncated tesseractic honeycomb
(No image)
TypeUniform 4-honeycomb
Schläfli symbolt0,1{4,3,3,4}
Coxeter-Dynkin diagram
4-face typetruncated tesseract
16-cell
Cell typeTruncated cube
Tetrahedron
Face type{3}, {8}
Vertex figureoctahedral pyramid
Coxeter group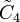{{\tilde {C}}}_{4} = [4,3,3,4]
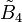{{\tilde {B}}}_{4} = [4,3,31,1]
Dual
Propertiesvertex-transitive

In four-dimensional Euclidean geometry, the truncated tesseractic honeycomb is a uniform space-filling tessellation (or honeycomb) in Euclidean 4-space. It is constructed by a truncation of a tesseractic honeycomb creating truncated tesseracts, and adding new 16-cell facets at the original vertices.

Related honeycombs

The [4,3,3,4], , Coxeter group generates 31 permutations of uniform tessellations, 21 with distinct symmetry and 20 with distinct geometry. The expanded tesseractic honeycomb (also known as the stericated tesseractic honeycomb) is geometrically identical to the tesseractic honeycomb. Three of the symmetric honeycombs are shared in the [3,4,3,3] family. Two alternations (13) and (17), and the quarter tesseractic (2) are repeated in other families.

Extended
symmetry		 Extended
diagram		 Order Honeycombs
[4,3,3,4]: ×1

1, 2, 3, 4,
5, 6, 7, 8,
9, 10, 11, 12,
13

[[4,3,3,4]] ×2 (1), (2), (13), 18
(6), 19, 20
[(3,3)[1+,4,3,3,4,1+]]
= [(3,3)[31,1,1,1]]
= [3,4,3,3]
=
= 		×6

14, 15, 16, 17

See also

Regular and uniform honeycombs in 4-space:

Notes

    References

    • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
      • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45] See p318
    • George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
    • Richard Klitzing, 4D, Euclidean tesselations#4D o3o3o *b3x4x, x4x3o3o4o - tattit - O89
    • Conway JH, Sloane NJH (1998). Sphere Packings, Lattices and Groups (3rd ed.). ISBN 0-387-98585-9. 
    Fundamental convex regular and uniform honeycombs in dimensions 2–11
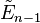    Family {{\tilde {A}}}_{{n-1}} {{\tilde {C}}}_{{n-1}} {{\tilde {B}}}_{{n-1}} {{\tilde {D}}}_{{n-1}} {{\tilde {G}}}_{2} / {{\tilde {F}}}_{4} / {{\tilde {E}}}_{{n-1}}
    Uniform tiling {3[3]} δ3 hδ3 qδ3 Hexagonal
    Uniform convex honeycomb {3[4]} δ4 hδ4 qδ4
    Uniform 5-honeycomb {3[5]} δ5 hδ5 qδ5 24-cell honeycomb
    Uniform 6-honeycomb {3[6]} δ6 hδ6 qδ6
    Uniform 7-honeycomb {3[7]} δ7 hδ7 qδ7 222
    Uniform 8-honeycomb {3[8]} δ8 hδ8 qδ8 133 • 331
    Uniform 9-honeycomb {3[9]} δ9 hδ9 qδ9 152 • 251 • 521
    Uniform n-honeycomb {3[n]} δn hδn qδn 1k2 • 2k1 • k21
    This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.