Triakis triangular tiling
| Triakis triangular tiling | |
|---|---|
 | |
| Type | Dual semiregular tiling |
| Coxeter-Dynkin diagram | |
| Faces | triangle |
| Face configuration | V3.12.12 |
| Symmetry group | p6m, [6,3], (*632) |
| Rotation group | p6, [6,3]+, (632) |
| Dual | Truncated hexagonal tiling |
| Properties | face-transitive |
In geometry, the triakis triangular tiling is a tiling of the Euclidean plane. It is an equilateral triangular tiling with each triangle divided into three obtuse triangles (angles 30-30-120) from the center point. It is labeled by face configuration V3.12.12 because each isosceles triangle face has two types of vertices: one with 3 triangles, and two with 12 triangles.
Conway calls it a kisdeltile,[1] constructed as a kis operation applied to a triangular tiling (deltille).
In Japan the pattern is called asanoha for hemp leaf, although the name also applies to other triakis shapes like the triakis icosahedron and triakis octahedron.[2]
Dual tiling
It is the dual tessellation of the truncated hexagonal tiling which has one triangle and two dodecagons at each vertex.[3]
Related polyhedra and tilings
The triakis triangular tiling is a part of a sequence of polyhedra and tilings, extending into the hyperbolic plane. These face-transitive figures have (*n32) reflectional symmetry.
| Symmetry *n32 [n,3] |
Spherical | Euclidean | Hyperbolic... | |||||
|---|---|---|---|---|---|---|---|---|
| *232 [2,3] D3h |
*332 [3,3] Td |
*432 [4,3] Oh |
*532 [5,3] Ih |
*632 [6,3] P6m |
*732 [7,3] |
*832 [8,3]... |
*∞32 [∞,3] | |
| Truncated figures |
 3.4.4 |
 3.6.6 |
 3.8.8 |
 3.10.10 |
 3.12.12 |
 3.14.14 |
 3.16.16 |
 3.∞.∞ |
| Coxeter Schläfli |
t{2,3} |
t{3,3} |
t{4,3} |
t{5,3} |
t{6,3} |
t{7,3} |
t{8,3} |
t{∞,3} |
| Uniform dual figures | ||||||||
| Triakis figures |
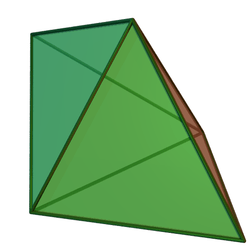 V3.4.4 |
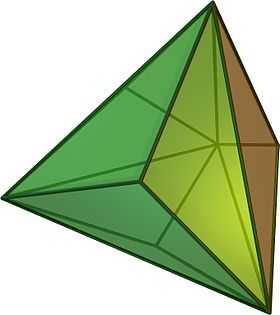 V3.6.6 |
 V3.8.8 |
 V3.10.10 |
 V3.12.12 |
 V3.14.14 |
 V3.16.16 |
 V3.∞.∞ |
| Coxeter | ||||||||
The triakis triangular tiling is a part of a set of uniform dual tilings, corresponding to the dual of the truncated hexagonal tiling.
| Symmetry: [6,3], (*632) | [6,3]+ (632) |
[1+,6,3] (*333) |
[6,3+] (3*3) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| {6,3} | t{6,3} | r{6,3} r{3[3]} |
t{3,6} t{3[3]} |
{3,6} {3[3]} |
rr{6,3} s2{6,3} |
tr{6,3} | sr{6,3} | h{6,3} {3[3]} |
h2{6,3} r{3[3]} |
s{3,6} s{3[3]} |
= |
= |
= |
= | |||||||
| Uniform duals | ||||||||||
| V63 | V3.122 | V(3.6)2 | V63 | V36 | V3.4.12.4 | V.4.6.12 | V34.6 | V36 | V(3.6)2 | V36 |
See also
| Wikimedia Commons has media related to Triakis triangular tiling. |
- Tilings of regular polygons
- List of uniform tilings
Notes
- ↑ John H. Conway, Heidi Burgiel, Chaim Goodman-Strass, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 21, Naming Archimedean and Catalan polyhedra and tilings, p288 table)
- ↑ http://www.mikworks.com/originalwork/asanoha/
- ↑ Weisstein, Eric W., "Dual tessellation", MathWorld.
References
- Grünbaum, Branko ; and Shephard, G. C. (1987). Tilings and Patterns. New York: W. H. Freeman. ISBN 0-7167-1193-1. (Chapter 2.1: Regular and uniform tilings, p. 58-65)
- Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. p39

