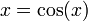Transcendental equation

From Wikipedia, the free encyclopedia

A transcendental equation is an equation containing a transcendental function. Such an equation cannot be solved for one factor in terms of another. Examples of such an equation are

x=e^{{-x}}
x=\cos(x)

Solution methods

Some methods of finding solutions to a transcendental equation use graphical or numerical methods.

For a graphical solution, one method is to set each side of a single variable transcendental equation equal to a dependent variable and plot the two graphs, using their intersecting points to find solutions.

The numerical solution extends from finding the point at which the intersections occur using some kind of numerical calculation. The solution of transcendental equation obtained by numerical methods are approximate solutions.[1] Approximations can also be made by truncating the Taylor series if the variable is considered to be small. Additionally, the fixed point iteration method, the bisection method, the method of false position, linear interpolation, the method of chords, the method of proportional parts, the Newton-Raphson method, secant method or method of tangents could be used to solve the equation.

Often special functions can be used to write the solutions to transcendental equations in closed form. In particular, the first example given above has a solution in terms of the Lambert W Function.

References

  1. ↑ Grewal, B. Higher Engineering Mathematics. Delhi: Khanna Publishers. ISBN 81-7409-195-5. 

See also

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.