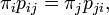Time reversibility

From Wikipedia, the free encyclopedia

Time reversibility is an attribute of some stochastic processes and some deterministic processes.

If a stochastic process is time reversible, then it is not possible to determine, given the states at a number of points in time after running the stochastic process, which state came first and which state arrived later.

If a deterministic process is time reversible, then the time-reversed process satisfies the same dynamical equations as the original process (see reversible dynamics); in other words, the equations are invariant or symmetric under a change in the sign of time. Classical mechanics and optics are both time-reversible. Modern physics is not quite time-reversible; instead it exhibits a broader symmetry, CPT symmetry.[citation needed]

Time reversibility generally occurs when, within a process, it can be broken up into sub-processes which undo the effects of each other. For example, in phylogenetics, a time-reversible nucleotide substitution model such as the generalised time reversible model has the total overall rate into a certain nucleotide equal to the total rate out of that same nucleotide.

Time Reversal, specifically in the field of acoustics, is a process by which the linearity of sound waves is used to reverse a received signal; this signal is then re-emitted and a temporal compression occurs, resulting in a reverse of the initial excitation waveform being played at the initial source. Mathias Fink is credited with proving Acoustic Time Reversal by experiment.

Stochastic processes

A formal definition of time-reversibility is stated by Tong[1] in the context of time-series. In general, a Gaussian process is time-reversible. The process defined by a time-series model which represents values as a linear combination of past values and of present and past innovations (see Autoregressive moving average model) is, except for limited special cases, not time-reversible unless the innovations have a normal distribution (in which case the model is a Gaussian process).

A stationary Markov Chain is reversible if the transition matrix {pij} and the stationary distribution {πj} satisfy

\pi _{i}p_{{ij}}=\pi _{j}p_{{ji}},\,

for all i and j.[2] Such Markov Chains provide examples of stochastic processes which are time-reversible but non-Gaussian.

Time reversal of numerous classes of stochastic processes have been studied including Lévy processes[3] stochastic networks (Kelly's lemma)[4] birth and death processes [5] Markov chains[6] and piecewise deterministic Markov processes.[7]

See also

Notes

  1. ↑ Tong(1990), Section 4.4
  2. ↑ Isham (1991), p 186
  3. ↑ Jacod, J.; Protter, P. (1988). "Time Reversal on Levy Processes". The Annals of Probability 16 (2): 620. doi:10.1214/aop/1176991776. JSTOR 2243828. 
  4. ↑ Kelly, F. P. (1976). "Networks of Queues". Advances in Applied Probability 8 (2): 416–432. doi:10.2307/1425912. JSTOR 1425912. 
  5. ↑ Tanaka, H. (1989). "Time Reversal of Random Walks in One-Dimension". Tokyo Journal of Mathematics 12: 159. doi:10.3836/tjm/1270133555. 
  6. ↑ Norris, J. R. (1998). Markov Chains. Cambridge University Press. ISBN 0521633966. 
  7. ↑ Löpker, A.; Palmowski, Z. (2013). "On time reversal of piecewise deterministic Markov processes". Electronic Journal of Probability 18. doi:10.1214/EJP.v18-1958. 

References

  • Isham, V. (1991) "Modelling stochastic phenomena". In: Stochastic Theory and Modelling, Hinkley, DV., Reid, N., Snell, E.J. (Eds). Chapman and Hall. ISBN 0-412-30390-9 .
  • Tong, H. (1990) Non-linear Time Series: A Dynamical System Approach. Oxford UP. ISBN 0-19-852300-9


This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.