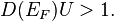Stoner criterion

From Wikipedia, the free encyclopedia

A condition to be fulfilled for the ferromagnetic order to arise in a simplified model of a solid. Named after Edmund Clifton Stoner.

Stoner model of ferromagnetism

Ferromagnetism ultimately stems from electron-electron interactions. The simplified model of a solid which is nowadays usually called the Stoner model, can be formulated in terms of dispersion relations for spin up and spin down electrons,

$E_{\uparrow }(k)=\epsilon (k)+I{\frac {N_{\uparrow }-N_{\downarrow }}{N}},\qquad E_{\downarrow }(k)=\epsilon (k)-I{\frac {N_{\uparrow }-N_{\downarrow }}{N}},$

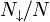where the second term accounts for the exchange energy, $N_{\uparrow }/N$ ( $N_{\downarrow }/N$ ) is the dimensionless density[1] of spin up (down) electrons and $\epsilon (k)$ is the dispersion relation of spinless electrons where the electron-electron interaction is disregarded. 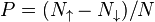If $N_{\uparrow }+N_{\downarrow }$ is fixed, $E_{\uparrow }(k),E_{\downarrow }(k)$ can be used to calculate the total energy of the system as a function of its polarization $P=(N_{\uparrow }-N_{\downarrow })/N$ . If the lowest total energy is found for P=0, the system prefers to remain paramagnetic but for larger values of I, polarized ground states occur. It can be shown that for

$2ID(E_{F})>1$

the P=0 state will spontaneously pass into a polarized one. This is the Stoner criterion, expressed in terms of the P=0 density of states[1] at the Fermi level 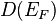$D(E_{F})$ .

Note that a non-zero P state may be favoured over P=0 even before the Stoner criterion is fulfilled.

Relationship to the Hubbard model

The Stoner model can be obtained from the Hubbard model by applying the mean-field approximation. The particle density operators are written as their mean value 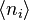$\langle n_{i}\rangle$ plus fluctuation $n_{i}-\langle n_{i}\rangle$ and the product of spin-up and spin-down fluctuations is neglected. We obtain[1]

$H=U\sum _{i}n_{{i,\uparrow }}\langle n_{{i,\downarrow }}\rangle +n_{{i,\downarrow }}\langle n_{{i,\uparrow }}\rangle -\langle n_{{i,\uparrow }}\rangle \langle n_{{i,\downarrow }}\rangle +\sum _{{i,\sigma }}\epsilon _{i}n_{{i,\sigma }}.$

Note the third term which was omitted in the definition above. With this term included, we arrive at the better-known form of the Stoner criterion

$D(E_{F})U>1.$

References

Stephen Blundell, Magnetism in Condensed Matter (Oxford Master Series in Physics).
Demonstrative derivation of the Stoner Criterion

Footnotes

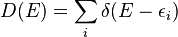1. Having a lattice model in mind, N is the number of lattice sites and $N_{\uparrow }$ is the number of spin-up electrons in the whole system. The density of states has the units of inverse energy. On a finite lattice, $\epsilon (k)$ is replaced by discrete levels $\epsilon _{i}$ and then $D(E)=\sum _{i}\delta (E-\epsilon _{i})$ .

This article is issued from Wikipedia. The text is available under the Creative Commons Attribution/Share Alike; additional terms may apply for the media files.